Points to Remember
|
1.1 |
The knee frequency , |
|
1.2 |
One neper equals 8.68588963806 dB. |
|
1.3.1 |
Large objects have more inductance and capacitance than small ones. |
|
1.3.1 |
High-frequency connectors must be very small to push the parasitic resonances up to frequencies above the bandwidth of the signal. |
|
1.3.1.2 |
Simultaneously enlarging the height and width of a transmission line has no effect on the characteristic impedance or per-unit-length delay. |
|
1.3.2 |
Lower-voltage logic is remarkably power-efficient. |
|
1.3.3 |
Shrinking every parameter of an unterminated structure speeds its settling time in direct proportion to the scale factor. |
|
1.3.3 |
Terminated structures circumvent the link between physical size and signal quality. |
|
1.3.4 |
A lower-impedance source coupled to a lower-impedance transmission line can drive larger capacitive loads. |
|
1.3.5 |
Reducing the dielectric constant of your transmission-line substrate increases the characteristic impedance and decreases the delay. |
|
1.3.6 |
Adjustments to magnetic permeability are rarely made in digital circuits. |
|
1.4 |
Low- Q , dissipative circuits can't resonate. This is a desirable feature for a digital transmission path . |
|
1.5 |
Resonance affects all physical structures, including bridges. |
|
2.1 |
Two long conductors insulated from each other with a uniform cross section make a good transmission line. |
|
2.1 |
The telegrapher's equations represent only the TEM mode of signal propagation. |
|
2.1 |
You can model almost any transmission line with the telegrapher's equations. |
|
2.2.1 |
The telegrapher's equations are derived from a cascaded lumped-element equivalent circuit model. |
|
2.2.2 |
In typical digital transmission situations on pcbs the characteristic impedance changes fairly slowly over the relevant frequency range. |
|
2.2.3 |
At frequencies above the LC and skin-effect mode onset, but below the onset of multiple waveguide modes of operation, the characteristic impedance is relatively flat and |
|
2.2.4 |
Signals propagating on a transmission line decay exponentially with distance. |
|
2.2.4 |
The per-unit-length attenuation factor H ( w ) is called the propagation function of a transmission line. |
|
2.2.4 |
The propagation coefficient g ( w ) is defined as the (negative of the) natural logarithm of H ( w ) |
|
2.2.4 |
The propagation coefficient g ( w ) may be broken down into its real and imaginary parts ( a and b ). |
|
2.2.4 |
The real part of g ( w ) defines the attenuation per unit length of a transmission structure in nepers per unit length. |
|
2.2.4 |
The imaginary part g ( w ) defines the phase shift per unit length of a transmission structure in radians per unit length. |
|
2.3 |
A lossless transmission line requires R = G = 0. |
|
2.3 |
For the special case of a lossless line |
|
2.4 |
The nonzero resistance of practical transmission lines dissipates a portion of the signal power, causing both attenuation (loss) and distortion in propagating signals. |
|
2.5 |
Shunt conductance G is practically zero at DC for the types of insulators used in most modern digital transmission applications. |
|
2.5 |
Dielectric loss models for high-frequency applications incorporate AC dielectric losses into the definition of complex permittivity, creating a capacitance term C with both real and imaginary parts. |
|
2.6 |
Magnetic fields within a conductor adjust the distribution of high frequency current, forcing it to flow only in a shallow band just underneath the surface of the conductor. |
|
2.6 |
The effective depth of penetration of current is called the skin depth . |
|
2.6 |
The increase in the apparent resistance of the conductor caused by this redistribution of current is called the skin effect . |
|
2.6 |
At frequencies above the skin-effect onset frequency w d the effective series resistance of a conductor rises with the square root of frequency. |
|
2.7 |
The distribution of current at high frequencies minimizes inductance. |
|
2.7 |
At DC, the path of least DC resistance creates a slightly higher inductance. |
|
2.7 |
Good models for skin effect take into account changes in both resistance and inductance with frequency. |
|
2.8 |
Merely adding the DC and AC models of resistance produces substantial errors at frequencies near the onset of the skin effect, and predicts the wrong value of internal inductance. |
|
2.8 |
The second-order approximation [2.52] better matches both the real and imaginary parts of the skin effect at frequencies near the transition region. |
|
2.10 |
The proximity effect distributes AC current unevenly around the perimeter of a conductor. |
|
2.10 |
The proximity factor increases the apparent AC resistance of a conductor above and beyond what you would expect from the action of the skin effect alone. |
|
2.10 |
Above that frequency where the proximity effect takes hold, the distribution of current around the perimeter of the conductor attains a minimum-inductance configuration and does not vary further with frequency. |
|
2.10 |
The skin effect and the proximity effect are two manifestations of the same principle: that magnetic lines of flux cannot penetrate a good conductor. |
|
2.10 |
Field simulators base their calculations on many assumptions, and don't always produce the right answers. |
|
2.11 |
At a microscopic level, all materials exhibit surface irregularities and bumps. |
|
2.11 |
Rough toothing profiles are purposefully etched into copper layers prior to lamination to facilitate adhesion between layers . |
|
2.11 |
Roughness on a scale comparable to the skin depth increases the mean length of the path of current, increasing the resistance. |
|
2.12 |
All insulators exhibit some degree of dielectric loss. |
|
2.12 |
Make sure you know the frequency at which a value of dielectric constant is specified. |
|
2.12.1 |
Dielectric losses in a transmission line scale in proportion to both frequency and length. |
|
2.12.1 |
The dielectric loss tangent is the tangent of the phase angle formed by the real and imaginary components of complex permittivity. |
|
2.12.1 |
For small loss tangents, l is approximately the same as the ratio of the imaginary part to the real part of complex permittivity. |
|
2.12.2 |
A mixed dielectric carries a permittivity |
|
2.12.2 |
Any air or water present in a dielectric mixture will change the dielectric constant of the resulting mixture. |
|
2.12.3 |
The loss tangent for a mixed dielectric can be calculated from the loss tangents and filling factors of the constituent materials. |
|
2.12.4 |
The filling factor for a dielectric-air mixture may be inferred from the velocity of propagation. |
|
2.12.5 |
The real and imaginary portions of any realizable network function bear certain subtle yet incontrovertible relations to each other. Specifying one without the other leads to non-realizeable circuit results. |
|
2.12.6 |
You can calculate a variation in dielectric constant to match any specified loss tangent. |
|
2.12.5 |
The Kramers-Kronig relations constrain the behavior of the real and imaginary parts of complex permittivity. |
|
2.13 |
An impedance in series with the return path affects the signal just as much as an impedance in series with the signal conductor. |
|
2.14 |
In an on-chip MIS configuration, if the electric fields penetrate to a depth of h 1 , while the magnetic fields penetrate to a futher depth h 2 , the resulting combination of large capacitance and large inductance creates an absurdly slow velocity of signal propagation. |
|
3.1 |
The signal propagation model computes the transfer function and impedance of cables made in multiwire, ribbon, UTP, STP, or coaxial format. It also works for pcb traces, both striplines and microstrips, up to a frequency of approximately 10 GHz. |
|
3.1 |
The parameter R DC provides an amount of loss that is flat with frequency. |
|
3.1 |
The parameter R provides an amount of loss that grows (in dB) in proportion to the square root of frequency |
|
3.1 |
The parameter q provides an amount of loss that grows (in dB) in direct proportion to frequency |
|
3.1 |
At all frequencies the magnitude and phase responses match to produce a causal , minimum-phase response |
|
3.2 |
Sweeping from low frequencies to high, the loss curve for a transmission line changes in a predictable way as you pass the onset of various regions of operation. |
|
3.2 |
The distinguishing features of each region are determined by the propagation coefficient, propagation function, and characteristic impedance. |
|
3.2 |
The regions usually appear in this order: lumped-element, RC, LC, skin-effect, dielectric, and waveguide. |
|
3.2.1 |
A pcb trace of any length always remains a transmission line, supportig two modes of propagation (out and back). |
|
3.2.1 |
When a transmission line is short, two modes of propagation still exist, only their temporal superposition creates the illusion of a direct connection between source and load. |
|
3.3 |
The undistorted conveyance of a signal from source to load requires a propagation function that remains flat over a band of frequencies covering the bulk of the spectral content of the data signal. |
|
3.4 |
The classification of a transmission line in the lumped-element region does not determine how the line is going to act. It determines merely how the line may be analyzed . |
|
3.4.1 |
A transmission line can always be shortened to a length below which it operates in the lumped-element region. |
|
3.4.1 |
Transmission lines short enough to operate in the lumped-element region rarely require termination. |
|
3.4.2 |
The pi model applies to any transmission line electrically short compared to the signal wavelength, and where the time constant l 2 R DC C remains small compared to the signal period. |
|
3.4.3 |
Within the lumped-element region you may use Taylor-series expansions for H and H “1 . |
|
3.4.4 |
The input impedance of a short, unloaded transmission line looks entirely capacitive. |
|
3.4.4 |
The input impedance of a short, grounded pcb-trace looks entirely inductive. |
|
3.4.5 |
Any transmission line can be shortened to the point where it acts as a perfect connection. |
|
3.4.5 |
If the source can't drive the load in the first place, then hooking the source and load together with a transmission line isn't likely to make things better. |
|
3.4.5 |
Conditions necessary such that a short, lumped-element transmission line not affect signal quality are given by [3.39] through [3.41]. |
|
3.4.6 |
Even a short transmission line may resonate horribly if used to interconnect a ferociously reactive combination of source and load. |
|
3.5 |
The terms RC transmission line, dispersive transmission line, and diffusion line all mean the same thing. |
|
3.5.1 |
The RC region extends in frequency from DC up to that point w LC where the magnitude of the line inductance ( w LC L ) equals the DC resistance ( R DC ). |
|
3.5.1 |
For any transmission line there exists a critical length below which you need never concern yourself with the distributed RC mode of operation. |
|
3.5.2 |
The input impedance of a line without reflections is predictable and independent of line length. |
|
3.5.2 |
An RC transmission line may be equalized using a suitable reactive source or load impedance network. |
|
3.5.3 |
Within the RC region, characteristic impedance is a complex function of frequency with a phase angle of “45 ° and a magnitude slope of -10 dB per decade . |
|
3.5.4 |
A perfectly -matched end termination applied to an RC transmission line renders the input impedance of the structure indepedant of line length. This advantage comes at the expense of a terrible degradation of the transfer response. |
|
3.5.4 |
A fixed resistance at the end of an RC transmission line flattens the gain curve, providing more usable bandwidth at the expense of a reduction in the received signal amplitude, and a greater variation with line length in the input impedance of the structure. |
|
3.5.4 |
A purely resistive end termination equal to |
|
3.5.6 |
A resistive termination at both ends of an RC-LC mixed-mode transmission line provides flatter gain than termination at only one end or the other. |
|
3.5.7 |
A fixed resistance at the end of an RC transmission line improves the settling time at the expense of a reduction in the received signal amplitude. |
|
3.5.8 |
The speed of operation achievable within the RC region scales inversely with the square of transmission-line length. |
|
3.5.10 |
The Elmore delay approximation takes into account only the resistance and capacitance of a transmission configuration. It applies to well-damped circuits composed of any number of series resistances, shunt capacitances, and distributed RC transmission lines. |
|
3.5.10 |
The Elmore delay approximation does not apply circuits involving inductance, resonance, overshoot, or any form of poorly damped or nonmonotonic behavior. |
|
3.5.10 |
The Elmore delay for a lumped resistance R feeding a total downstream capacitance of C is RC . |
|
3.5.10 |
The Elmore delay for a distributed RC transmission line having total resistance R and distributed capacitance C is (1/2) RC . |
|
3.6.1 |
The LC region begins where the magnitude of the line inductance ( w L ) exceeds the DC resistance ( R DC ). |
|
3.6.1 |
Within the LC region the line attenuation does not much vary with frequency. |
|
3.6.1 |
Unfortunately, in the practical transmission lines used in most digital designs the LC region is relatively narrow (or sometimes nonexistent). |
|
3.6.2 |
At frequencies far above w LC the characteristic impedance of a pure LC-mode transmission line asymptotically approaches |
|
3.6.2 |
At a frequency ten times greater than w LC the complex value of characterisitic impedance differs from Z by only about 4%. |
|
3.6.3 |
A fixed series resistance induces an upward tilt in the TDR measurement, indicating a gradually increasing impedance at lower frequencies. |
|
3.6.4 |
The propagation function for an LC-mode transmission line is a simple delay with a fixed attenuation. |
|
3.6.4 |
Doubling the length of an LC-mode transmission line doubles the delay, and doubles the attenuation (in dB). |
|
3.6.5 |
In the LC region a signal can accumulate a substantial amount of phase delay without suffering much attenuation. This property indicates that a transmission line can act as an extremely high-Q resonant circuit. |
|
3.6.5 |
LC-mode resonance affects only signals whose bandwidth extends into the resonant region |
|
3.6.6 |
The source, end, and both-ends terminations can all be used to eliminate LC-mode resonance. |
|
3.6.6 |
The end-termination is least sensitive to the series resistance of the transmission line. |
|
3.6.7 |
The speed of operation achievable within the LC region is not directly limited by transmission-line length. |
|
3.6.8 |
Transmission lies operated at a length greater than l RC may display characteristics of both LC and RC behavior. |
|
3.7.1 |
The skin effect region starts when the internal inductance of the signal conductor becomes significant compared to its DC resistance. |
|
3.7.1 |
Within the skin effect region the characteristic impedance remains fairly flat, while the line attenuation in dB varies in proportion to the square root of frequency. |
|
3.7.2 |
At frequencies far above w d the characteristic impedance of a skin-effect limited transmission line asymptotically approaches |
|
3.7.2 |
The asymptotic convergence is not quite as fast as for an LC transmission structure with fixed (non-frequency-varying) resistance. |
|
3.7.3 |
The skin effect induces an upward tilt in the TDR measurement with a steep initial slope, gradually tapering to a more gentle rise. |
|
3.7.4 |
The attenuation (in dB) within the skin-effect region grows in proportion to the square root of frequency. |
|
3.7.4 |
Doubling the length of a skin-effect-limited transmission line doubles the attenuation. |
|
3.7.5 |
Transmission lines in the skin-effect region fall prey to the same resonance difficulties that afflict the LC region and respond to the same means of termination. |
|
3.7.6 |
The step response associated with the skin effect has a quick rise and a long, sloping tail. |
|
3.7.7 |
The risetime of a skin-effect-limited channel scales with the square of its length. |
|
3.7.7 |
The speed of operation within the skin-effect region scales inversely with the square of transmission-line length. |
|
3.7.7 |
A conductor twice the diameter (or width) has 1/2 the AC resistance and thus 1/4 the skin-effect risetime. |
|
3.7.7 |
It's rare in pcb problems that you see skin-effect losses without also having to take into account dielectric dispersion. |
|
3.8.1 |
Skin-effect loss grows only in proportion to the square root of frequency, while the dielectric loss grows in direct proportion to frequency. Above some frequency w q the dielectric loss equals, and then exceeds, the skin-effect loss. |
|
3.8.2 |
In the vicinity of the skin-effect onset w d the skin effect increases characteristic impedance while dielectric loss decreases it. |
|
3.8.2 |
At frequencies above the onset of the dielectric-loss-limited mode w q , dielectric losses ultimately force the characteristic impedance back up above Z . |
|
3.8.3 |
Dielectric losses cause an upward tilt to a plot of characteristic impedance versus frequency. Resistive losses create a neagtive slope. Working together, the two effects can sometimes almost cancel, creating a TDR slope less steep than when either effect is present alone. |
|
3.8.4 |
The attenuation (in dB) within the dielectric-loss-limited region grows in direct proportion to frequency. |
|
3.8.4 |
Doubling the length of a dielectric-loss-limited transmission line doubles the attenuation. |
|
3.8.5 |
The dielectric effect induces a gradual rise in the characteristic impedance Z C in proportion to the log of freqeuncy. |
|
3.8.5 |
Transmission lines in the dielectric-loss-limited region fall prey to the same resonance difficulties that afflict the LC region and respond to the same means of termination. |
|
3.8.6 |
Given two systems with the same “3dB loss at frequency f 1 , one system having only dielectric losses and the other having only skin-effect losses, the dielectric step response begins more slowly than the skin-effect response, but finishes sooner. |
|
3.8.7 |
The risetime of a dielectric-loss-limited channel scales directly with its length. |
|
3.8.7 |
The speed of operation within the dielectric-loss region scales inversely with transmission-line length. |
|
3.8.7 |
A dielectric medium with twice the loss tangent incurs twice the loss (in dB) and induces a settling time twice as long. |
|
3.8.7 |
It's rare in pcb problems that you see skin-effect losses without also having to take into account dielectric dispersion. |
|
3.9.1 |
If the wavelengths of the signals conveyed approach the dimensions of your conductors, strange modes of propagation begin to appear. |
|
3.9.1 |
For ordinary digital signaling on FR-4 printed circuit boards at 10 Gbps you may use microstrip trace heights up to 20 mils without encountering significant microstrip dispersion. |
|
3.11 |
Over the range of frequencies dominated by the skin effect, you can scale the length of one coaxial cable type to cause it to mimic the performance of any other type. |
|
3.12 |
Five ways to improve the performance of a copper transmission channel: use more copper, don't go as far, use a higher characteristic impedance, add equalization, or use a better dielectric material. |
|
3.13 |
The performance of a metallic interconnection is heavily affected by its physical construction, which is comparatively well controlled in the manufacturing process. Metallic transmission systems have a relatively hard, fixed upper limit on distance that should never be exceeded. |
|
3.15 |
Intersymbol interference may be characterized by a dispersion penalty. |
|
3.15 |
The dispersion penalty may be circumvented by equalization. |
|
4.1 |
Frequency-domain simulation gives you incredible control over the exact form of frequency-dependent losses, like the skin effect and dielectric-loss effect. |
|
4.1 |
Frequency-domain simulators may be easily programmed in any software spreadsheet application (like MatLab, Mathematica, or MathCad), giving you control over every aspect of the simulation, including searching for optimum and worst-case parameter values. |
|
4.1 |
Frequency-domain simulation applies only to linear systems. |
|
4.2 |
The DFT is a discrete-time approximation to the Fourier transform. |
|
4.2 |
The popular Cooley-Tukey FFT algorithm is a clever, highly efficient implementation of the DFT that works only for N equal to a power of two. |
|
4.3 |
The FFT requires two parameters: a sample interval D T and a sample vector length N . |
|
4.3 |
The spacing D T must be small enough to fairly represent the complete signal waveform without loss of significant information. |
|
4.3 |
Always provide an N large enough to allow your simulated system to come to a steady-state condition before the end of the FFT time window. |
|
4.4 |
The FFT requires that your signal waveform have the same value at start and finish. |
|
4.5 |
Most FFT routines require external scale factors that depend on the sample interval D T and sample vector length N. |
|
4.6 |
Table 4.1 shows how to form FFT frequency vectors for test signals, data patterns, pulses , and feathered edges. |
|
4.7 |
In simulations of high-speed digital systems an inadequate sampling rate causes a waveform to "wiggle around" as a function of precisely where it is sampled. |
|
4.9 |
Frequency-domain simulation handles some pretty complicated situations. |
|
4.10 |
Before using an unfamiliar FFT routine, check the transform of a simple impulse at time 0 and also the transform of the same impulse delayed by one sample. |
|
5 |
Analysis of pcb performance generally assumes well-defined uniform paths for both signal current and returning signal current, conductors long compared to the spacing between the signal and return paths, and conductors shorter than the critical RC length l RC . |
|
5.1.1 |
If you don't already have a 2-D field solver, get one. |
|
5.1.2.1 |
A 1/2-oz copper pcb trace with 100- m m (3.9 mil) width has a DC resistance of 9.6 W /m. The DC resistance scales inversely with the width and inversely with the copper plating weight. |
|
5.1.2.2 |
Low-frequency current in a pcb trace therefore follows the path of least resistance , filling the cross-sectional area of the trace, |
|
5.1.2.2 |
The skin effect confines high-frequency current to a shallow band of depth d around the perimeter of a conductor. |
|
5.1.2.2 |
The proximity effect draws signal current towards the side of a microstrip facing the reference plane, or that side of a stripline that faces the nearest reference plane. |
|
5.1.2.2 |
The increase in resistance of a typical high-speed digital signal conductor due to the proximity effect (above and beyond simple consideration of the skin depth and trace circumference assuming a uniform current distribution) typically ranges from 25% to 50%. |
|
5.1.2.2 |
Another similar- sized increase in resistive dissipation occurs due to the nonuniform distribution of current on the reference plane. |
|
5.1.2.2 |
Traces with similar ratios of w / h inherit similar values of k p regardless of the dielectric constant. |
|
5.1.2.6 |
You can simulate the magnetic field surrounding a pc-board stripline using a rubber sheet and a Popsicle stick. |
|
5.1.2.7 |
At frequencies on the order of 1 GHz, nickel-plating the top surface of a microstrip cuts in third the effective useful length the trace. |
|
5.1.3 |
For FR-4 digital circuit board applications with risetimes of 500 ps or slower, at distances up to 10 inches, you may ignore dielectric losses. |
|
5.1.3 |
At longer distances or at higher speeds, dielectric losses can become quite significant. |
|
5.1.3.1 |
A microstrip has dielectric properties intermediate between the properties of the dielectric substrate and air. |
|
5.1.3.4 |
Core and prepreg laminate materials are now available in a staggering array of types and variations. |
|
5.1.3.4 |
The core or prepreg laminate comprises a fabric of fine threads embedded in a solidified resin. |
|
5.1.3.4 |
Inhomogeneities in a fabric-resin laminate ultimately limit the size of the thinnest dielectric that can be produced from that combination of materials. |
|
5.1.3.5 |
The dielectric loss of a backplane may change substantially with temperature. |
|
5.1.3.6 |
Copper traces on outer layers may be protected from corrosion by passivation or by coating them with an inert material. |
|
5.1.4 |
The skin-effect step produces a sharper initial rise, but a longer, more lingering tail, than does the dielectric effect. |
|
5.1.5.1 |
For normal digital signaling on FR-4 pc boards at 10 Gbps, you may use any trace height up to 0.5 mm (0.020 in.) without encountering significant microstrip dispersion. |
|
5.2 |
As you stretch the channel length to extreme distances, sensitivity-limited systems fail due to insufficient signal amplitude at the receiver. |
|
5.2 |
Dispersion-limited systems fail due to signal distortion, also called intersymbol interference (ISI). |
|
5.2 |
Amplifying the received signal does not change the performance of a dispersion-limited system. Equalization is what helps. |
|
5.2 |
Systems limited by dispersion may sometimes be improved by a change in data coding. |
|
5.2.1 |
A non-linear DC restoration system can un-do the effects of AC coupling. |
|
5.3.1.1 |
Pcb traces terminated at both ends enjoy a great advantage in immunity to reflections as compared to their singly terminated cousins. |
|
5.3.1.2 |
A small lumped-element capacitance shunting a transmission line creates a backwards -propagating reflection. |
|
5.3.1.2 |
A small lumped-element inductance in series with a transmission line does the same, but with the opposite polarity. |
|
5.3.1.3 |
Adjustments to transmission-line width can partially compensate for one small, isolated capacitive load. |
|
5.3.1.4 |
Adjustments to transmission-line width can partially compensate for one small, isolated series inductance. |
|
5.3.1.5 |
Right-angle bends in pc-board traces perform perfectly well in digital designs in speeds as fast as 2 Gbps. |
|
5.3.1.6 |
Blind or buried vias are smaller and have less effect than full-sized vias. |
|
5.3.1.7 |
Densely packed component pads greatly reduce trace impedance. |
|
5.3.1.8 |
Place a series terminator no more than a small fraction of one risetime away from the driver. |
|
5.3.1.9 |
Place an end terminator no more than a small fraction of one risetime from the end of the line. |
|
5.3.1.10 |
A low-impedance driver combined with a tight-tolerance resistor in series makes an accurate series termination. |
|
5.3.1.10 |
A high-impedance current-source driver combined with a tight-tolerance resistor in shunt across the driver also makes an accurate series termination. |
|
5.3.1.11 |
Impedance translation over any band that includes DC is accomplished using a resistive pad. |
|
5.3.2.1 |
Solid reference planes exist to control crosstalk. |
|
5.3.2.2 |
Crosstalk varies strongly with trace separation and with the trace height above the reference planes. |
|
5.3.2.2 |
A field solver is the best way to estimate crosstalk for general digital purposes, provided that no holes, slots, or gaps in the planes cross the path of either the victim or aggressor trace. |
|
5.3.2.3 |
Crosstalk is highly directional. |
|
5.3.2.3 |
Whether initially headed forward or backward, crosstalk reflects and bounces off any imperfections in the transmission structure, often ending up at both ends of the line. |
|
5.3.2.4 |
For parallel traces shorter than half the signal risetime, near-end crosstalk varies in proportion to the length of parallelism. |
|
5.3.2.4 |
For parallel traces longer than half the signal risetime, near-end crosstalk saturates at a maximum level. The ratio of crosstalk to aggressive step-size at saturation is the NEXT coefficient. |
|
5.3.2.4 |
Saturated NEXT looks like a long, low rectangle with a flat top and a duration equal to twice the trace delay plus one source risetime. |
|
5.3.2.5 |
Far-end crosstalk varies in proportion to the trace length. |
|
5.3.2.5 |
FEXT looks like a short pulse with a duration equal to the source risetime. |
|
5.3.2.6 |
The both-ends terminated stripline architecture greatly reduces , but does not completely eliminate, both FEXT and NEXT. |
|
5.3.2.7 |
Both voltage and current affect crosstalk. |
|
5.4.1 |
Connector crosstalk in open -pin-field connectors acts through a transformer-like principle. |
|
5.4.1 |
Separating the loops of signal current within a connector by providing private power or ground pins for each signal reduces crosstalk. |
|
5.4.1 |
Reducing the current in the aggressive circuit reduces crosstalk. |
|
5.4.2 |
Never assume your fabrication shop will build the board the way you ask. Always check. |
|
5.4.3 |
Three primary measures of connector performance are signal fidelity, crosstalk, and EMI. |
|
5.4.4 |
A tapered transition maintains constant impedance when interconnecting transmission lines having widely different physical scales. |
|
5.4.5 |
A straddle-mount connector locates all its pins close to the plane of the pcb. |
|
5.4.6 |
A high-frequency shield needs direct metallic contact with the product chassis, completely surrounding the signal conductors. |
|
5.5.1 |
The properties of a via are modified by the trace to which it is attached. |
|
5.5.1 |
Inductance is a property of an entire current pathway (a loop of current). Don't use partial inductance values by themselves . |
|
5.5.1 |
A via contributes incremental shunt capacitance and incremental series inductance to a trace. |
|
5.5.2 |
If the incremental capacitance or inductance of your via is not sufficient to cause an objectionable reflection, then no model is required. |
|
5.5.2 |
A first-order model reduces the via to either a single value of excess shunt capacitance, or a single value of excess series inductance, according to which effect creates the greatest reflection. |
|
5.5.2 |
If your via is so large compared to the signal risetime that you require anything more than a simple pi-model for the via, then it probably isn't going to work very well for a digital application. Use a smaller via. |
|
5.5.2 |
Narrowband applications sometimes use large vias at frequencies well beyond the useful band for digital applications. |
|
5.5.3 |
A long, dangling via can develop a resonance, exacerbating the effects of its capacitance. |
|
5.5.4 |
The incremental capacitance of a via is affected by the geometry of the via, the surrounding reference planes, the trace width used to connect to the via, and the dielectric constant of the substrate material. |
|
5.5.4 |
Via capacitance varies in proportion to the overall size of the via. |
|
5.5.5.1 |
The inductance of a signal via depends on the location of the return path associated with that signal via. |
|
5.5.5.1 |
A signal via that traverses only one plane keeps the returning signal current close at hand all along the signal pathway. |
|
5.5.5.1 |
A signal via that traverses two reference planes forces returning signal current through the nearest available interplane connection. |
|
5.5.5.1 |
If a signal changes reference planes from a ground plane to a power plane (or vice versa), the interplane return path must traverse bypass capacitors. |
|
5.5.5.1 |
Pcb vendors , often without telling you, make last-minute changes to hole, pad, and clearance sizes in an attempt to improve their finished board yield. |
|
5.5.5.2 |
Vias that traverse a common stripline cavity (i.e., the space between two reference planes) create crosstalk. |
|
5.5.5.2 |
The crosstalk voltage induced in a victim circuit equals the rate of change of current in the aggressor times the mutual inductance, L M , shared between the two circuits. |
|
6.1 |
The big advantage of single-ended signaling is that it requires only one wire per signal. |
|
6.1 |
Single-ended signaling falls prey to disturbances in the reference voltage. |
|
6.1 |
Single-ended signaling is susceptible to ground bounce. |
|
6.1 |
Single-ended signaling requires a low-impedance common reference connection. |
|
6.2 |
Two-wire signaling renders a system immune to disturbances in distribution of global reference voltages. |
|
6.2 |
Two-wire signaling counteracts any type of interfering noise that affects both wires equally. |
|
6.2 |
Two-wire signaling counteracts ground bounce (also called simultaneous switching noise) within a receiver. |
|
6.2 |
Two-wire signaling counteracts ground shifts in connectors. |
|
6.2 |
Two-wire signaling works when there is no significant stray returning signal current. |
|
6.3 |
Differential signaling delivers equal but opposite AC voltages and currents on two wires. |
|
6.3 |
Assuming the layout is symmetrical, any AC currents induced in the reference system by one wire are counteracted by equal and opposite signals induced by the complementary wire. |
|
6.3 |
Differential pcb traces need not be tightly coupled to be effective. |
|
6.3 |
Differential signaling markedly reduces radiated emissions. |
|
6.4 |
Differential and common-mode signals are used to describe the voltages and currents on a two-wire transmission system. |
|
6.4 |
Odd-mode and even-mode signals are yet another way to describe the voltages and currents on a two-wire transmission system. |
|
6.4 |
Differential receivers cancel common-mode noise. |
|
6.5 |
Microstrips support slightly different propagation velocities for the differential and common modes. The impact of this difference is not very great. |
|
6.6 |
Common-mode balance is the ratio of common-mode to differential-mode signal amplitudes. |
|
6.7 |
Don't violate the common-mode input range specification for a receiver (not even briefly ). |
|
6.8 |
An imbalanced circuit can translate part of a perfectly good differential signal into a common-mode signal, or vice versa. |
|
6.9 |
Differential impedance is the impedance measured between two conductors when they are driven in the differential mode. |
|
6.9 |
Odd-mode impedance is the impedance measured on either of two conductors when they are driven with opposite signals in the differential mode. |
|
6.9 |
The value of differential-mode impedance is twice the value of odd-mode impedance. |
|
6.9 |
The differential impedance of two matched, uncoupled transmission lines is double the impedance of either line alone. |
|
6.9 |
The odd-mode impedance of two matched, uncoupled transmission lines equals the impedance of either line alone. |
|
6.9 |
Coupling between two parallel pcb traces decreases both differential and odd-mode impedances. |
|
6.9 |
Common-mode impedance is the impedance measured on two wires in parallel when they are driven together. |
|
6.9 |
Even-mode impedance is the impedance measured on either of two wires when they are driven with identical signals in the common mode. |
|
6.9 |
The value of common-mode impedance is half the value of even-mode impedance. |
|
6.9.3 |
Aside from the complications introduced by unbalanced modes, differential transmission lines behave pretty much like single-ended ones. |
|
6.10.2 |
Differential traces can be pushed really, really close together. If you do so, compute a new trace width to compensate for the fact that the differential impedance goes down for closely spaced pairs. |
|
6.10.2 |
Widely spaced (i.e., loosely-coupled) pairs are not subject to picky, difficult-to-implement spacing and width requirements. |
|
6.10.2 |
The most important determiner of skin-effect loss is the trace width. |
|
6.10.2 |
An interpair trace separation of four times h yields about a 6% effect on impedance, a small enough value in many cases to simply ignore. |
|
6.10.2 |
Matching the elements of each pair to within 1/20 of a risetime limits the common-mode signal contributed by trace skew to less than 2.5% of the single-ended signal amplitude. |
|
6.10.3 |
If you separate elements of a tightly-coupled pair the differential impedance reverts to twice the uncoupled value of Z . |
|
6.10.4 |
Broadside differential trace impedance is maximized by a trace height equal to 25% of the interplane separation. |
|
6.10.4 |
The bottom trace of a broadside-coupled differential pair has some extra delay built in at the endpoints. |
|
6.10.4 |
Avoid broadside-coupled traces unless they are made necessary by routing considerations. |
|
6.11.1 |
Match the differential characteristic impedance of two pcb traces to the differential characteristic impedance of a balanced cable. |
|
6.11.1 |
Make the two pcb traces as symmetrical as possible, with equal impedances to ground. |
|
6.11.2 |
Differential signaling defeats ground bounce. |
|
6.11.3 |
You need not struggle to place ordinary differential digital traces any closer than 0.5 mm (0.020 in.) for any EMI purpose. |
|
6.11.4 |
Subject to the limits of common-mode rejection , ground shifts generated within a connector are totally cancelled within a differential receiver. |
|
6.11.5 |
Differential receivers often have more accurately specified switching thresholds than single-ended receivers. |
|
6.11.5 |
Uncoupled differential traces need not follow the same path; they just need to have the same delay. |
|
6.11.6 |
Tightly coupling a differential pair delivers only a modest improvement in crosstalk. |
|
6.11.8 |
The benefits of differential signaling apply to multidrop configurations. |
|
6.11.9 |
Every long, differential link needs at least one good differential termination and also a reasonable common-mode termination to prevent severe common-mode resonance. |
|
6.11.10 |
Visualize the propagation of a differential signal as a quad of four currents. |
|
6.11.11 |
Chamfering or rounding of differential corners does not eliminate skew. |
|
6.11.12 |
A pair that starts and ends going north has by definition equal numbers of right-hand and left-hand turns. |
|
6.12 |
The twisted-pair cable guarantees low crosstalk by virtue of having a different rate of twist on all the pairs within the same jacket. |
|
6.12 |
Quad cable guarantees low crosstalk by virtue of its unique geometrical alignment. |
|
6.12.1 |
Ribbon cables can use the same twist pitch on every pair because the wires are held in a rigid geometry. |
|
6.12.2 |
Never introduce a metallic connection between any two frames powered by different AC power sources. |
|
6.12.2 |
If you must electrically connect two boxes, make sure that both boxes are served by green-wire grounds connected to the same Earth potential. |
|
6.12.2 |
Differential signaling with unshielded cables does not require a direct ground connection between the two ends of the link. |
|
6.12.3 |
To get the best RF-rejection performance from your cabling, |
|
6.12.3 |
Use a tightly twisted, well-balanced cable. Twisted cables work better than quad cables in this respect. |
|
6.12.3 |
Don't scrimp on connectors. Buy and use connectors designed to go with the cable. |
|
6.12.3 |
Use well-balanced circuitry for both transmitter and receiver. |
|
6.12.4 |
Differential receivers have more accurate switching thresholds than ordinary single-ended logic. |
|
6.13.1 |
Normal operating voltages for LVDS logic are 1.2 ± 0.2 V on each wire. |
|
6.13.2 |
LVDS, like most digital transceivers, is not extraordinarily well balanced. |
|
6.13.3 |
The common-mode noise tolerance for general-purpose LVDS logic is ±925 mV. |
|
6.13.4 |
The high noise margin gives LVDS a built-in natural advantage in combating ringing, overshoot, and crosstalk from like devices. |
|
6.13.5 |
Always provide fast-edged inputs to LVDS logic. |
|
6.13.6 |
LVDS works best with 100- W transmission lines. |
|
6.13.7 |
You need not struggle to place ordinary differential digital traces any closer than 0.5 mm (0.020 in.) for any EMI purpose. |
|
6.13.8 |
Always double-check your final artwork to make sure you've met the specifications for skew. |
|
6.13.9 |
Fail-safe features are permitted by the LVDS standard, but not required . |
|
7 |
Any system that connects from room to room, or from building to building, should use generic building cabling. |
|
7 |
Building-cabling standards are evolving rapidly . If you want the latest information, order the latest standards. |
|
7.1 |
Horizontal cabling is the most widely deployed, highest-volume element of the building-cabling architecture. |
|
7.1 |
New buildings in North America provide two outlets in every work area, with four-pair, 100- W UTP, category 5 or better cabling to both outlets. |
|
7.1 |
Backbone cables are mostly a mix of category 5 cables, multimode fiber (62.5- m m or 50- m m), and some single-mode fiber. |
|
7.1 |
A weird backbone cabling requirement is a sales obstacle to be overcome . A weird horizontal cabling requirement is a wooden stake in the heart of your project. |
|
7.2 |
Don't underestimate the complexity of proper SNR budgeting. |
|
7.6 |
Multi-pair building cables should be installed straight-through with no crossing of the pairs. |
|
7.6 |
When necessary, an external crossover should be implemented in a short, clearly visible section of cabling and boldly labeled. |
|
7.7 |
The materials used to make fire-resistance plenum-rated cables are heavy, stiff, and somewhat more expensive than PVC. |
|
7.8 |
Cable performance must be de-rated to account for operation at the elevated temperatures commonly found in building attics. |
|
8 |
Cabling standards proliferate faster than bunnies. |
|
8.1 |
Compared to category 3 cabling, categories 5e and 6 higher have progressively tighter twists and better plastic insulation with less dielectric loss at high frequencies. The resulting cables pick up less noise and have a superior frequency response. |
|
8.1.2 |
The many possible combinations of surface plating, types of shielding, and dielectric make it difficult to accurately predict the performance of all twisted-pair cables from the basic information provided on a datasheet. |
|
8.1.2 |
The cable model you use for system simulation should either add another 2 dB of fixed, flat loss to the datasheet attenuation or extend the simulated maximum cable length by another 10% to 20%. |
|
8.2 |
Timing jitter is improved when all received amplitudes are independent of past history. |
|
8.2 |
Simple fixed pre-emphasis boosts the maximum operational cable length of a Manchester-coded link by at least 50%. |
|
8.2 |
A more sophisticated adaptive equalizer can extend operation to even greater distances. |
|
8.3.1 |
A complete noise budget takes into account all reflections within a cabling system. |
|
8.3.1 |
Connectors generate reflections that superimpose on the reflections generated by changes in cable impedance. |
|
8.3.2 |
Bidirectional links must tolerate near-end reflections. |
|
8.3.2.1 |
A specification of structural return loss combined with a specification of the mean value of characteristic impedance is used for old category 3 cables. |
|
8.3.2.1 |
A single specification of cable return loss (as measured with the cable terminated in a 100- W load) simultaneously limits both the mean value and local perturbations in cable impedance. |
|
8.3.2.2 |
Structural return noise is modeled as a summation of many noise sources with random amplitudes. |
|
8.3.2.2 |
Structural return noise grows at a rate of 15 dB per decade. |
|
8.3.3 |
A hybrid circuit makes possible bidirectional full-duplex transmission through a single channel. |
|
8.3.3 |
A sufficiently complex adaptive digital filter can compensate for cable roughness and also cable-transition reflections simultaneously. Such a filter is called an adaptive echo cancellation circuit. |
|
8.3.4 |
NEXT is modeled as a summation of many noise sources with random amplitudes. |
|
8.3.4 |
NEXT grows at a rate of 15 dB per decade. |
|
8.3.5 |
Alien crosstalk comes from devices occupying unused pairs within your cable jacket. |
|
8.3.6 |
FEXT is modeled as a single noise source with a random amplitude. |
|
8.3.6 |
FEXT grows at a rate of 20 dB per decade. |
|
8.3.7 |
Within a single jacket there may be one combination of pairs that press up against the limit for pair-to-pair NEXT or ELFEXT, but not all combinations of pairs may do so. |
|
8.3.8 |
The best antidote for RFI is good signal balance. |
|
8.3.8 |
A 27-MHz low-pass filter applied to category-3 horizontal cabling should cut RFI to less than 40 mV in most commercial situations. |
|
8.3.8 |
Categories 5e and 6 cabling pick up less RFI. |
|
8.3.9 |
The key to obtaining good radiated performance is good common-mode balance. |
|
8.3.9 |
Scrambling spreads the spectral power density of the transmitted signal, reducing the peak radiation. |
|
8.4 |
UTP connectors are cheap, and the performance is outstanding. |
|
8.4 |
Systems that tolerate polarity reversal greatly simplify installation. |
|
8.5 |
Even though screened cables are heavily favored in Europe, this author does not recommend their use. |
|
8.6 |
Never use PVC-insulated category 3 cables in an uncooled attic space. |
|
9 |
Think about 150- W STP-A when you need a quick and dirty transceiver for a first product release (or beta-trial). |
|
9.2 |
When 150- W STP-A is used in a unidirectional mode it is not subject to near-end reflections, alien crosstalk, or far-end crosstalk. |
|
9.3 |
Inside a 150- W STP-A cable, the signal on one wire of a pair might arrive ahead of the signal on the other wire. |
|
9.3 |
Pigtail and AC-coupled shields work at audio frequencies, but not at a gigahertz. |
|
9.4 |
Customers will not maintain the shields on an STP system. |
|
9.5 |
The equipment-end connector used with FDDI and Ethernet 150- W STP-A installations is the shielded DB-9. |
|
10 |
The electrical performance of coaxial cable is as good as anything else, but physically, coax is difficult to handle. |
|
10 |
Coaxial cable suffers from an overabundance of standards. |
|
10.1 |
A good coaxial cable presents a nearly uniform impedance at all frequencies above the onset of the skin effect. |
|
10.1 |
Coaxial cables formed from foamed, cellular, or helically-wrapped dielectrics exhibit a faster propagation velocity and less high-frequency loss than their solid-dielectric counterparts. |
|
10.1 |
The step response duration for a coaxial cable scales roughly in proportion to the square of cable length. |
|
10.1.2 |
A characteristic impedance of approximately 50 W minimizes the skin-effect losses in a solid-polyethylene coaxial cable. |
|
10.1.3 |
Wimpy drivers appreciate higher-impedance transmission lines. |
|
10.1.3 |
I consider IBM's selection of 150- W for STP-A a goof. |
|
10.1.3 |
50- W coax is less sensitive than 75- W coax to reflections caused by transceiver taps. |
|
10.2.1 |
Coaxial cables are generally manufactured to much tighter impedance standards than UTP cables. |
|
10.2.3 |
RF susceptibility and radiation in coaxial cables result from imperfections in the shield. |
|
10.2.4 |
If you block the direct path of signal current with an isolating device, such as a transformer, optical isolator , or differential receiver, then you are free, as far as signal integrity is concerned , to disconnect the coax ground from your equipment ground. |
|
10.2.4 |
A common-mode choke can also block the flow of intercabinet ground current. |
|
10.2.4 |
DC-balanced signals are perfectly suited for connection through transformers . |
|
10.3 |
Above 100 MHz, you should always match the characteristic impedance of the connector to the cable. |
|
10.3 |
Contact plating serves to stave off corrosion and eventual failure of the contacts. |
|
10.3 |
If it goes on a boat, a car, a plane, or anything that moves, use threaded connectors. |
|
10.3 |
Crimp-style connectors generally superior to the other types for high-frequency work. |
|
10.3 |
Always specify heat-treated beryllium-copper for critical contact springs. |
|
11 |
The bandwidth-carrying capacity of modern fiber- optic cabling greatly exceeds that of any form of copper cabling, an advantage counterbalanced by the high costs and practical difficulties associated with fiber. |
|
11.1 |
Glass optical fiber is drawn as one continuous thread from a single cylinder of purified glass called a preform . |
|
11.2 |
The key parameter that differentiates fiber in the marketplace is core diameter. |
|
11.3 |
The optical properties of the fiber are determined almost entirely by the coated glass core. |
|
11.3 |
The mechanical properties of the cable are determined almost entirely by the buffer and jacket construction. |
|
11.4 |
The three most popular wavelength windows for glass fiber are (1) 770 nm to 860 nm, (2) 1270 nm to 1355 nm, and (3) 1500 nm to 1600 nm. |
|
11.5 |
The two most popular standard core diameters for multimode glass fiber are 50 m m and 62.5 m m. |
|
11.5 |
A graded-index multimode fiber higher bandwidth than a step-index multimode fiber of the same core diameter and quality. |
|
11.5.1 |
Within a multimode fiber, there exist hundreds of different pathways , or modes of propagation |
|
11.5.1 |
The multiple modes cause a step input to gradually disperse in time as it travels down the fiber. |
|
11.5.1 |
Dispersion in a multimode fiber is divided into modal dispersion and chromatic dispersion. |
|
11.5.1 |
Modal bandwidth is a function of the refractive index profile of the fiber. |
|
11.5.1 |
Chromatic dispersion is a function of the material properties of the glass and also the refractive index profile of the fiber. |
|
11.5.2 |
Carefully grading the profile of the index of refraction greatly improves modal bandwidth. |
|
11.5.3 |
Internationally recognized specifications for 50 and 62.5 m m multimode optical fibers are provided by IEC 793-2. |
|
11.5.4 |
Fifty-micron multimode fiber has a generally higher bandwidth and less attenuation than 62.5- m m multimode fiber. These advantages are counterbalanced by the fact that some common LED sources can't couple efficiently into 50- m m core. |
|
11.5.5.1 |
Dispersion calculations determine the extent of risetime degradation and estimate the impact that degradation will have on signal reception . |
|
11.5.5.2 |
An attenuation budget allocates attenuation among the long continuous runs of fiber cabling, the short fiber jumpers , and the connectors in a typical installation. |
|
11.5.6 |
Fiber-optic transmission systems commonly divide the jitter budget into deterministic jitter and random jitter . |
|
11.5.7 |
Fiber cabling may be immune to crosstalk and RFI, but your fiber-optic receiver is not. |
|
11.5.8 |
Never look into the end of a fiber. |
|
11.5.9 |
The use of laser-diodes on multimode fiber depends on subtle, undocumented, and unspecified features of the multimode fiber. |
|
11.5.10 |
A VCSEL shines perpendicular to its top surface, just like a surface-emitting LED. |
|
11.5.11 |
No one has yet designed a satisfactory, easy to install, inexpensive fiber-optic connector. |
|
11.5.11 |
Optics work well for intersystem connections, but I've not yet seen a cost-effective optical backplane. |
|
11.6.1 |
Single-mode fiber does not suffer from modal dispersion, differential mode delay, modal noise, or mode partition noise. |
|
11.6.1 |
The most important optical parameters for a single-mode fiber are the operating wavelength, the attenuation in dB/km, and the chromatic dispersion. |
|
12 |
Clock signals, because they are so fast, so heavily loaded, and so important for system timing, are subject to special requirements. |
|
12.1 |
DLL or PLL technology can produce arbitrary, precise, intentional clock skew where and when you need it. |
|
12.2 |
Timing margin measures the slack , or excess time, remaining in each clock cycle. |
|
12.2 |
Lowering the clock frequency fixes setup problems, but not hold problems. |
|
12.2 |
Clock skew affects operating speed as much as any other propagation delay. |
|
12.3 |
The performance of a clock tree structure depends heavily on the input-to-output uncertainty of the clock repeaters. |
|
12.3 |
Keeping the clock repeater isolated in its own package is a good idea. |
|
12.3.1 |
A skew-compensated clock repeater architecture does nothing to combat uncertainty in the overall input-to-output delay. |
|
12.3.1 |
Actively compensated clock repeaters are highly susceptible to power supply noise. |
|
12.3.2 |
A zero-delay clock buffer directly controls the input-to-output uncertainty. |
|
12.3.3 |
What you really want is low skew as defined at the points of usage . |
|
12.4 |
Given similar dielectrics, signals propagate faster on a microstrip layer than on a stripline layer. For best speed matching, don't mix the two types. |
|
12.5 |
For low skew, use the same clock drivers everywhere, source-terminate every driver, and use the same length line with the same impedance and the same loading on every trace. |
|
12.6 |
The spread between V IL and V IH creates an uncertainty in the exact moment at which a clock receiver will switch. |
|
12.7 |
Resistive loading attenuates the output of a digital driver, but does not change its rise (or fall) time. |
|
12.8.3 |
Delay elements are built from three basic building blocks: transmission lines, logic gates, and passive lumped circuits. |
|
12.8.3 |
A fixed delay cannot cancel variations in board fabrication or active component delay. |
|
12.8.3 |
An adjustable delay compensates for actual delays, not just nominal delays, elsewhere in the circuit. |
|
12.8.3 |
Whatever form of delay you choose, incorporate its uncertainty in delay into your timing margin calculations. |
|
12.8.5 |
Avoid long, coupled switchbacks. |
|
12.9 |
A single driver can service two or more source-terminated lines only under limited conditions. |
|
12.9.1 |
A slow driver can damp the ringing on a hairball network, but it may need to be too slow for your circuit. |
|
12.9.1 |
Appropriately placed attenuating networks can damp all the oscillatory modes at the expense of shrinking the received signal. |
|
12.9.1 |
A weak termination can help reduce, but totally cure, overshoot and ringing. |
|
12.9.1 |
Test all combinations of maximum and minimum load capacitance and line length. |
|
12.9.1 |
Eventually, someone will inherit your hairball design and try to figure out what you did. Keep it simple. |
|
12.9.2 |
Hidden within every split-tee network is an unconstrained resonance. |
|
12.10 |
Five things reduce the reflection from an isolated, lumped-element capacitive load: slow the risetime, lower the capacitance, lower the characteristic impedance of the trace, isolate the load with a big resistor, or compensate for the capacitance by modulating the trace width. |
|
12.10.1 |
Rules for good daisy-chaining ”Uniformly space the loads, with a spacing whose delay is small compared to the signal rise and fall time, and terminate the structure with a resistance that matches the effective impedance of the loaded structure you've built, not just the impedance of the raw trace you started with. |
|
12.11 |
PLL-based clock generators require a stable, low-jitter reference clock. |
|
12.11.1.2 |
Any sort of resonance in a PLL, even a tiny one, spells disaster for a highly cascaded system. |
|
12.11.1.3 |
The variance of the tracking error in a PLL circuit represents all the power in the input reference signal that falls above the tracking range of the PLL. |
|
12.11.1.4 |
A large ratio between the reference clock frequency and the PLL output frequency requires a very stable VCO. |
|
12.11.1.5 |
Jitter in the output of a PLL comes from internal sources plus noise coupled from the power system and noise propagated from the reference input. |
|
12.11.1.6 |
The point of separating jitter into random and deterministic components is to avoid overly stringent specifications for deterministic jitter. |
|
12.11.2.1 |
The noise properties of a PLL are characterized by the intrinsic internal jitter, the power supply sensitivity, and a jitter transfer function. |
|
12.11.2.2 |
You can calculate the variance of jitter using a spectrum analyzer. |
|
12.12 |
Filters designed for wideband operation are built from a cascade of multiple sections, each section scaled to provide coverage in successively higher frequency bands. |
|
12.12.1 |
Observing the noise between V CC and ground always returns useful information. |
|
12.12.2 |
A power-supply filter does not eliminate noise ”it merely copies junk from one circuit node to another, eliminating the difference between them. |
|
12.13.1 |
A modulated clock can never be used as the reference clock input to any advanced data communication transceiver. |
|
12.13.2 |
A scrambled clock spreads the clock emissions without modulating the mean clock frequency. |
|
12.14 |
Reduced-voltage clock signaling saves power and cuts EMI at the expense of noise susceptibility. |
|
12.15 |
The physical means of providing extra crosstalk protection are simple; the logistical means are complex. |
|
12.16 |
On a short line, if a range of series termination values will work, the biggest value minimizes the transmitted current and therefore the emissions. |
|
13.1 |
If by using a simulator you can save one design spin on one circuit board, the simulator pays for itself. |
|
13.2 |
Signal-integrity simulations may be performed in what-if mode or post-processing mode. |
|
13.2 |
Tool sets are highly differentiated according to their degree of software integration . |
|
13.2 |
Automated tools can be as dangerous as they are powerful and easy to use. |
|
13.3.2 |
All signal-integrity time-domain analysis tools use simulation techniques pioneered by SPICE. |
|
13.3.2 |
Especially on circuits containing inductive spikes or hard corners in the I-V curves, SPICE may fail to converge. |
|
13.3.2 |
Some versions of SPICE have a lower limit on the smallest permissible step size. |
|
13.3.2 |
Check your documentation to make sure TOL and REFTOL are set properly for your application. |
|
13.3.2 |
If your parameter extraction efforts fail to properly account for all the significant parasitic elements in a circuit, SPICE results will be incorrect. |
|
13.3.3 |
The SPICE lossless transmission-line model is computationally efficient. |
|
13.3.3 |
For typical pcb traces up to 25 cm (10 in.) long, at risetimes of 1 ns or slower, a lossless transmission-line model serves adequately well. Higher speeds and greater distances require the use of a transmission-line model that accounts for the skin effect and dielectric-loss . |
|
13.3.3 |
Lossy transmission-line models take a lot longer to run. |
|
13.3.4 |
When you first start working with any simulator, begin by setting up some simple, low-frequency test circuits for which you can predict the response by hand calculations. |
|
13.4.5 |
IBIS is an international standard for the electrical specification of chip drivers and receivers. |
|
13.4.5 |
IBIS specifies how to record the various parameters of a chip driver or receiver, but it does not specify what to do with them. |
|
13.4.5 |
IBIS is the best, most comprehensive, and genuinely useful piece of signal-integrity technology to come along in a great while. |
|
13.4.5 |
We need our chip vendors to provide IBIS model files for every part they make. |
|
13.4.5 |
At the time of publication, the IBIS committee maintained work-in-progress copies of its latest draft standards at the Electronic Design Automation (EDA) and Electronic Computer-Aided Design (ECAD) one-stop standards resource: http://www.eda.org/pub/ibis . |
|
13.6 |
Specify circuit behavior under conditions similar to the actual conditions present in your application. |
|
13.7 |
IBIS simulators don't yet properly compute SSO noise. |
|
13.8.1 |
Real live EMI problems are much too complex for even the best software tools. |
|
13.9 |
If your system design depends on the natural power-plane capacitance, compare the roundtrip delay across your board to your clock period ”you could be headed for resonance problems. |
Fundamentals
- Impedance of Linear, Time-Invariant, Lumped-Element Circuits
- Power Ratios
- Rules of Scaling
- The Concept of Resonance
- Extra for Experts: Maximal Linear System Response to a Digital Input
Transmission Line Parameters
- Transmission Line Parameters
- Telegraphers Equations
- Derivation of Telegraphers Equations
- Ideal Transmission Line
- DC Resistance
- DC Conductance
- Skin Effect
- Skin-Effect Inductance
- Modeling Internal Impedance
- Concentric-Ring Skin-Effect Model
- Proximity Effect
- Surface Roughness
- Dielectric Effects
- Impedance in Series with the Return Path
- Slow-Wave Mode On-Chip
Performance Regions
- Performance Regions
- Signal Propagation Model
- Hierarchy of Regions
- Necessary Mathematics: Input Impedance and Transfer Function
- Lumped-Element Region
- RC Region
- LC Region (Constant-Loss Region)
- Skin-Effect Region
- Dielectric Loss Region
- Waveguide Dispersion Region
- Summary of Breakpoints Between Regions
- Equivalence Principle for Transmission Media
- Scaling Copper Transmission Media
- Scaling Multimode Fiber-Optic Cables
- Linear Equalization: Long Backplane Trace Example
- Adaptive Equalization: Accelerant Networks Transceiver
Frequency-Domain Modeling
- Frequency-Domain Modeling
- Going Nonlinear
- Approximations to the Fourier Transform
- Discrete Time Mapping
- Other Limitations of the FFT
- Normalizing the Output of an FFT Routine
- Useful Fourier Transform-Pairs
- Effect of Inadequate Sampling Rate
- Implementation of Frequency-Domain Simulation
- Embellishments
- Checking the Output of Your FFT Routine
Pcb (printed-circuit board) Traces
- Pcb (printed-circuit board) Traces
- Pcb Signal Propagation
- Limits to Attainable Distance
- Pcb Noise and Interference
- Pcb Connectors
- Modeling Vias
- The Future of On-Chip Interconnections
Differential Signaling
- Differential Signaling
- Single-Ended Circuits
- Two-Wire Circuits
- Differential Signaling
- Differential and Common-Mode Voltages and Currents
- Differential and Common-Mode Velocity
- Common-Mode Balance
- Common-Mode Range
- Differential to Common-Mode Conversion
- Differential Impedance
- Pcb Configurations
- Pcb Applications
- Intercabinet Applications
- LVDS Signaling
Generic Building-Cabling Standards
- Generic Building-Cabling Standards
- Generic Cabling Architecture
- SNR Budgeting
- Glossary of Cabling Terms
- Preferred Cable Combinations
- FAQ: Building-Cabling Practices
- Crossover Wiring
- Plenum-Rated Cables
- Laying Cables in an Uncooled Attic Space
- FAQ: Older Cable Types
100-Ohm Balanced Twisted-Pair Cabling
- 100-Ohm Balanced Twisted-Pair Cabling
- UTP Signal Propagation
- UTP Transmission Example: 10BASE-T
- UTP Noise and Interference
- UTP Connectors
- Issues with Screening
- Category-3 UTP at Elevated Temperature
150-Ohm STP-A Cabling
- 150-Ohm STP-A Cabling
- 150- W STP-A Signal Propagation
- 150- W STP-A Noise and Interference
- 150- W STP-A: Skew
- 150- W STP-A: Radiation and Safety
- 150- W STP-A: Comparison with UTP
- 150- W STP-A Connectors
Coaxial Cabling
- Coaxial Cabling
- Coaxial Signal Propagation
- Coaxial Cable Noise and Interference
- Coaxial Cable Connectors
Fiber-Optic Cabling
- Fiber-Optic Cabling
- Making Glass Fiber
- Finished Core Specifications
- Cabling the Fiber
- Wavelengths of Operation
- Multimode Glass Fiber-Optic Cabling
- Single-Mode Fiber-Optic Cabling
Clock Distribution
- Clock Distribution
- Extra Fries, Please
- Arithmetic of Clock Skew
- Clock Repeaters
- Stripline vs. Microstrip Delay
- Importance of Terminating Clock Lines
- Effect of Clock Receiver Thresholds
- Effect of Split Termination
- Intentional Delay Adjustments
- Driving Multiple Loads with Source Termination
- Daisy-Chain Clock Distribution
- The Jitters
- Power Supply Filtering for Clock Sources, Repeaters, and PLL Circuits
- Intentional Clock Modulation
- Reduced-Voltage Signaling
- Controlling Crosstalk on Clock Lines
- Reducing Emissions
Time-Domain Simulation Tools and Methods
- Ringing in a New Era
- Signal Integrity Simulation Process
- The Underlying Simulation Engine
- IBIS (I/O Buffer Information Specification)
- IBIS: History and Future Direction
- IBIS: Issues with Interpolation
- IBIS: Issues with SSO Noise
- Nature of EMC Work
- Power and Ground Resonance
Points to Remember
Appendix A. Building a Signal Integrity Department
Appendix B. Calculation of Loss Slope
Appendix C. Two-Port Analysis
- Appendix C. Two-Port Analysis
- Simple Cases Involving Transmission Lines
- Fully Configured Transmission Line
- Complicated Configurations
Appendix D. Accuracy of Pi Model
Appendix E. erf( )
Notes
EAN: N/A
Pages: 163
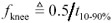 Hz, is a crude estimate of the highest frequency content within a particular digital signal.
Hz, is a crude estimate of the highest frequency content within a particular digital signal.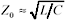
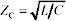 and
and 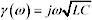 .
. equal to the weighted average, on a volumetric basis, of the permittivities of the constituent materials:
equal to the weighted average, on a volumetric basis, of the permittivities of the constituent materials: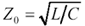 eliminates reflections within the LC band while also providing a relatively flat propagation function within the RC band.
eliminates reflections within the LC band while also providing a relatively flat propagation function within the RC band.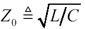 .
.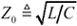 .
.