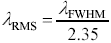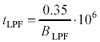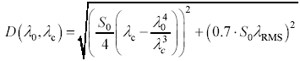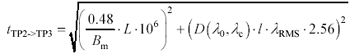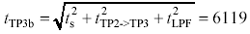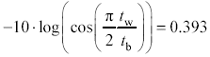Multimode Glass Fiber-Optic Cabling

Most glass fibers are manufactured with a standard cladding diameter of 125 m m and a polymer coating of 250 m m. They all look the same to the naked eye. They all flex the same amount. [93] Yet, their performance varies tremendously. The optical differences have to do with subtle variations in the index of refraction of the core and with the purity and clarity of the glass.

[93] Yes, glass is flexible. When you push on a window, the glass flexes slightly. Thinner glass flexes more, in inverse proportion to the square of its thickness . Glass that is only 125 m m thick, like an optical fiber, becomes quite flexible.

The two most popular standard core diameters for multimode glass fiber are 50 m m and 62.5 m m. Both cores operate as multimode cables for all transmission wavelengths within the wavelength range of 700 nm to 1600 nm. Larger core diameters are rarely used for high-speed applications because they admit too much modal dispersion (see Section 11.5.1). The core and cladding diameter specifications are usually given together, written as a pair like this: 62.5/125 m m.

Figure 11.8 illustrates the construction of a standard 62.5/125 m m graded-index multimode glass fiber core. The nominal index of refraction [94] of both core and cladding is approximately 1.5 with subtle variations in the central core region. The subtle variations in the index of refraction give fiber its unique properties.

[94] The velocity of propagation of light in a clear material is inversely proportional to the index of refraction. When light encounters a change in the index of refraction, the rays of light are bent or reflected.

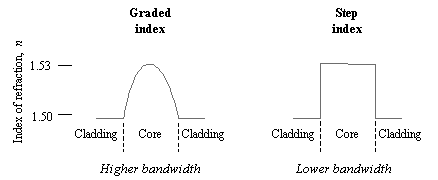
The term graded index refers to the shape of the refractive index profile in the core area of the fiber. [95] Multimode fibers used in modern LAN applications have an inverted- parabolic refractive index profile (shown in Figure 11.8). This type of graded-index profile produces a very high-bandwidth fiber. Older multimode fibers have a stepped refractive index profile (Figure 11.9). The step-index profile is easier (and cheaper) to make, but does not produce as high a bandwidth, as explained in Section 11.5.2, "Why Is Graded-Index Fiber Better than Step-Index?".

[95] The index of refraction for a perfect graded-index core is circularly symmetric, being a function only of the radial distance from the center of the core. Looking at a cross-section of the core, if you draw a horizontal line across the center of the core and plot the refractive index as a function of position along this line, you will have measured the refractive index profile for the fiber.

Figure 11.8. Construction of a graded-index glass fiber core (62.5/125 m m MMF).

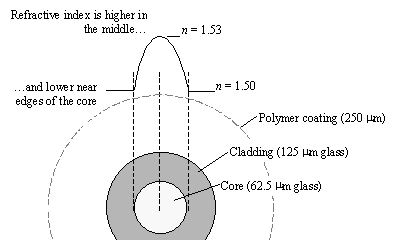
Figure 11.9. Refractive index profiles for two styles of multimode fiber.

POINTS TO REMEMBER

• The two most popular standard core diameters for multimode glass fiber are 50 m m and 62.5 m m.
• A graded-index multimode fiber higher bandwidth than a step-index multimode fiber of the same core diameter and quality.

 

11.5.1 Multimode Signal Propagation

The first violinist in a concert production emits sound waves in all directions. These different directions of sound propagation, or acoustic modes, scatter off the walls, ceiling, and floor, eventually reaching your ears. In a well-designed symphony hall, the various acoustic modes will all (hopefully) arrive closely grouped in time. That makes the music sound live, but not too reverberating. If the same concert were presented in a larger symphony hall, say, the New Orleans Superdome, the multiple disparate echoes would make it very difficult to properly interpret the music. [96]

[96] Unless you are listening to grunge music, which might be improved by the echo effect (anything would probably improve grunge music).

A similar principle applies to fiber-optic signal propagation. Within a multimode fiber, there exist hundreds of different pathways , or modes of propagation. Some go straight down the center of the core, some bounce back and forth off the cladding walls, and others spiral around the center of the core as they make their way down the cable. What you need to understand about modal propagation in fibers is this: The modes don't interact very much as they travel. Each mode proceeds separately at its own pace, with a unique value of attenuation and delay .

What is the effect of this multimodal structure on a propagating signal? The primary effect is that a step transition in the transmitted power becomes gradually dispersed in time as its travels down the fiber.

Figure 11.10 illustrates the dispersion effect. This figure shows a broad-beam transmitting source on the left, like an LED. The source couples optical power into all the conducting modes of the fiber. [97] Such a launch condition is called an overfilled launch .

[97] An LED does not couple equal amounts of power into each mode; however, all modes are used.

Figure 11.10. Each mode within a fiber propagates at a slightly different speed.

The independent propagating modes (only three are shown) appear temporally aligned near the source. As the modes progress, they each experience different values of attenuation and delay. Even though the modal delays in modern graded-index multimode fibers are matched to within better than 1 part in 1,000, the difference in arrival times at the end of a long fiber can become quite noticeable.

The receiver has no way to distinguish the arriving modes. It simply sums the power in all the arriving modal signals. On a rising edge, this summing action causes the receiver output to begin rising when the first mode arrives. The received waveform doesn't stop rising until after the arrival of the slowest, latest mode. If the spread in arrival times exceeds the natural risetime of the optical source, the incoming edge will be noticeably dispersed, or stretched out. The same effect happens on falling edges. The longer the fiber, the greater the modal separation, and the greater the rise/fall time dispersion.

Sufficiently large amounts of dispersion cause the tail of one edge to overlap with the beginning of the next . This type of eye pattern distortion degrades the receiver noise margin and must be included in the optical power budget . The power budget, and the way it accounts for dispersion, are the subjects of Section 11.5.5.2, "Multimode Attenuation Budget."

Specifications for dispersion in a multimode fiber are broken down into two categories, modal dispersion and chromatic dispersion . The modal and chromatic dispersion specifications separately account for dispersion due to the patterns of propagation of light within the fiber and the variations in refractive index with wavelength respectively.

Let's take a quantitative look at modal dispersion. [98] A multimode fiber illuminated with only one wavelength of light (presumably this comes from a perfect LED having a zero spectral width) displays only its modal dispersion t m . The various rays bang around inside the fiber, emerging with a total dispersion proportional to the length of the fiber.

[98] Here I use a ray-tracing analogy to describe the propagation of modes. This analogy, while not perfect, is a useful way to mentally visualize the modes. For a better analogy, you may recall from the study of ordinary differential equations Bessel's solution for the behavior of the surface of a round drum. The drum vibrates in a number of modes. Each mode is characterized by a number of concentric rings and a number of undulations as a function of angular position. The modes in a fiber-optic cable better resemble drumhead patterns than individual rays. For further study, see [90].

The modal dispersion of a fiber may be estimated given its modal bandwidth and length:

Equation 11.1

 

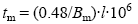
where	t m is the 10% to 90% modal dispersion risetime, in ps,
	B m is the 6-dB modal bandwidth of the fiber (FWHM bandwidth), in MHz-km, and
	l is the length of the fiber, in km.

NOTE:

The term B m represents the 6-dB modal bandwidth of a 1-km fiber. In other words, it is the frequency in MHz at which the electrically detected signal amplitude from a sinusoidal optical source would be 6 dB below the detected signal amplitude at low frequencies. At this 6-dB electrical loss frequency, the received optical peak-to-peak power is down only by half (a 3-dB optical power penalty). The optical detector changes optical power to electrical voltage , changing a 3-dB optical power loss into a 6-dB electrical signal loss. The 6-dB loss point is the traditional specification method used for modal bandwidth.

Modal bandwidth is improved by manipulating the refractive index profile of the fiber as it is manufactured to create a structure that propagates all possible modes at more nearly the same speed. It is also improved by using a smaller core, which tends to restrict the number of possible modes.

Next let's examine chromatic dispersion. A fiber illuminated with a practical LED having a finite spectral width displays not only modal dispersion t m , but also a degree of chromatic dispersion t c , due to the fact that different wavelengths of light travel at different speeds in glass. As the spectral width of the source increases , so does the chromatic dispersion.

The chromatic dispersion of a fiber may be estimated given its chromatic dispersion constant , length, and the spectral width of the source:

Equation 11.2

 

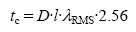
where	t c is the 10% to 90% chromatic dispersion risetime, in ps,
	D is the chromatic dispersion constant, in ps/nm-km,
	l is the length of the fiber, in km,
	l RMS is the RMS spectral width of the source, in nm (the RMS spectral width is the standard deviation of the curve of power density versus wavelength), and
	2.56 is a constant that converts standard-deviation-type pulse width measurements to 10% to 90% measurements.

NOTE:

The term l RMS represents the RMS spectral width, in units of nanometers, of the source. It is defined as the standard deviation of a plot of source power versus wavelength. The RMS width is one traditional specification method used for spectral width (that is, you spread out the light using a diffraction grating or prism, then you put it into an electrical detector to convert power to voltage, then you plot the detector voltage versus wavelength, then you determine the standard deviation of that plot). Had the optical source been characterized by a FWHM spectral width (defined at the -3dB power, or -6dB electrical, points), the term l RMS would be replaced with l FWHM , and the conversion factor to 10% to 90% risetime would be changed to 1.09.

LED operation at 850 nm suffers markedly from chromatic dispersion. LED operation at 1300 nm suffers less, because 1300 nm lies near a natural null in the chromatic dispersion of glass. For LED-based systems, which have a source spectral width on the order of 150 nm, there is therefore a natural advantage to operation at 1300 nm.

A laser-based source produces a much narrower spectral width than an LED. Most laser sources are therefore less susceptible to chromatic dispersion at all wavelengths, although they may be affected by other peculiar factors (see Section 11.5.9, "Multimode Fiber with Laser Source").

Careful analysis reveals two primary factors that contribute to chromatic dispersion:

1. Variations in the refractive index of the glass as a function of wavelength (material dispersion), and
2. Variations in the penetration depth of each wavelength into the core/cladding interface ( waveguide dispersion).

The first factor was discovered by Newton. Light travels at different speeds in glass, depending on its wavelength. Prisms used for spectrographic analysis work on this principle.

In an ideal fiber, the refractive index would not vary with wavelength, so that all wavelengths of light would travel at the same speed. In a practical fiber variations in the refractive index always occur. These variations arise due in part to the nature of amorphous silicon, and in part to the presence of trace impurities.

The second factor arises because different wavelengths of light tend to penetrate , or bleed over, to different depths within the core-cladding interface. Any change in the relative mix of signal power carried in the core versus cladding portions of the fiber affects the overall effective index of refraction experienced by waves at that particular wavelength and mode number; therefore, the speed of propagation varies with wavelength [90] .

A natural null in chromatic dispersion occurs in glass near 1300 nm. Careful management of the index-of-refraction profile can modify the position and depth of this null. A fiber in which the dispersion curve has been intentionally changed is called a dispersion-shifted fiber, Dispersion-shifted fibers can be produced with a null anywhere in the range of 1300 to 1600 nm (Figure 11.11). Typical values for dispersion in the first window (800 nm) are on the order of 85 ps/nm-km. Shifting the dispersion null to the center of the third window improves operation in that window at the expense of worse performance in the second window.

Figure 11.11. The dispersion curve may be shifted, or flattened, by manipulating the index-of-refraction profile within the core.

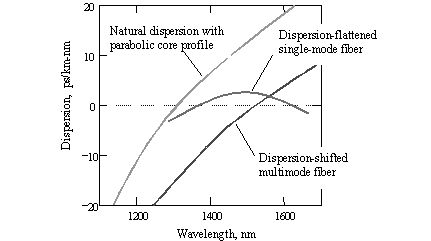
In the case of single-mode fiber intended for use only in the second or third window, sophisticated manipulation of the index-of-refraction profile within the core can produce a dispersion-flattened fiber, which has a low value of dispersion across a wide range of wavelengths within those windows .

Chromatic dispersion in typical installations is improved by the use of the 1300-nm operating wavelength (as opposed to 850 nm), by the use of narrow spectral-width sources like VCSEL laser diodes (as opposed to LEDs), and by limiting the link to short distances.

Modal and chromatic dispersion combine like this to produce the overall fiber dispersion t f :

Equation 11.3

 

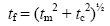
POINTS TO REMEMBER

• Within a multimode fiber, there exist hundreds of different pathways, or modes of propagation.
• The multiple modes cause a step input to gradually disperse in time as it travels down the fiber.
• Dispersion in a multimode fiber is divided into modal dispersion and chromatic dispersion.
• Modal bandwidth is a function of the refractive index profile of the fiber.
• Chromatic dispersion is a function of the material properties of the glass and also the refractive index profile of the fiber.

 

11.5.2 Why Is Graded-Index Fiber Better than Step-Index?

The core/cladding interface in a step-index fiber acts like an almost-perfect mirror. According to Snell's law [91] , waves internal to a step-index fiber that graze the core/cladding interface at a sufficiently shallow angle will experience total internal reflection. No power is lost. Such waves may bounce back and forth between the cladding walls as many times as necessary as they travel down the fiber. This bouncing effect describes the essence of multimode fiber operation.

In the simple ray-tracing diagram (Figure 11.12), those modes that travel straight down the fiber experience the fewest reflections, have the straightest path , and therefore arrive with the least delay. Those modes that travel at the greatest angle with respect to the central core experience the greatest delay. [99]

[99] Ray-tracing is an imperfect description of how modes actually propagate in a circular fiber, but it's the best we can do without having to solve a bunch of Bessel's equations.

Figure 11.12. A reflecting ray takes a longer path than the straight ray.

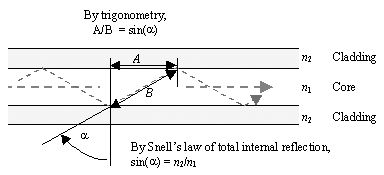
The maximum dispersion that can occur in a step-index fiber has to do with the ratio of transit times between the slowest and fastest modes in the fiber. Here's where Snell's law comes in. It constrains the maximum angle at which light can propagate in the fiber. Using Snell's law you can predict that the maximum ratio of transit times, based on the ray-tracing analogy, equals n 2 / n 1 , where n 2 and n 1 represent the index of refraction in the cladding and core areas respectively.

For a typical step-index, the quantity n 2 / n 1 equals approximately 1.01, or 1% greater than unity. In a 100-m cable with a natural delay of 400 ns, the step-index dispersion (worst-case difference in delays) would therefore be 1% of 400 ns, or 4 ns.

If you take a careful look at the ray-tracing picture, you may spot an easy way to improve this situation. Notice how the reflecting ray spends a lot of time near the edges of the fiber, but relatively little in the core. The central, straight ray is the other way around. It spends its time in the middle. If you artificially increase the index of refraction exactly in the center of the core, the central ray slows down, but it doesn't much affect the reflecting ray . This effect helps to balance the delay between modes. It also turns out that slightly reducing the index of refraction near the edges of the core speeds up the most broadly reflecting rays. A careful job of shaping the index profile can theoretically improve modal dispersion by orders of magnitude. The resulting fiber, which has gradual, controlled variations of the index of refraction within the core region, is called a graded-index fiber.

POINT TO REMEMBER

• Carefully grading the profile of the index of refraction greatly improves modal bandwidth.

 

11.5.3 Standards for Multimode Fiber

Internationally recognized specifications for 50 and 62.5 m m multimode optical fibers are provided by IEC 793-2 [86] . If you are planning to design systems that will be installed using existing in-building cabling, you should familiarize yourself with IEC 793-2. For each standard core diameter, IEC 793-2 identifies a large number of standard modal bandwidth and attenuation categories for operation at either 850 nm or 1300 nm. These are the two most popular wavelengths available for fiber-optic system design. Transceiver components for a third major wavelength range, at 1550 nm, are recently becoming widely available.

In the early days of fiber development, a fiber would be heavily optimized for use at 850 nm or for use at 1300 nm, but not both. Today the situation has changed. Today most fibers are specified for use at either wavelength. Fibers specified for use at either 850 or 1300 nm are called dual-window fibers.

System designers can call out from Table 11.1 minimum standard bandwidth categories for dual-window fibers that suit their application and can expect that users will be able to purchase and install these cables. Should the user change systems, possibly to a system that operates at a different wavelength, the dual-window property will protect the investment in the installed cabling.

Specifications for dual-window attenuation or bandwidth are usually written with a slash, like this: 62.5/125 m m 160/500 MHz-km. The first two figures specify a 62.5 m m core with a 125 m m cladding. The next two figures give the modal bandwidth, in MHz-km, at operating wavelengths of 850 nm and 1300 nm respectively.

If you are looking for "safe" values that will cover a larger percentage of the installed base, you can take a tip from the IEEE 802.3z Gigabit Ethernet committee. Based on customer surveys conducted in 1997, the committee picked minimum bandwidth figures of 160/500 MHz-km for 62.5/125 m m fiber and 400/400 MHz-km for 50/125 m m fiber (highlighted in bold below). If you design your system to work with these values, you will find a large installed base of cable already in the field that can support your application.

Specifications for fiber performance continue to evolve . For example, ISO 11801 standards stipulate a slightly higher bandwidth for 62.5/125 m m fiber of 200/500 MHz-km. In 1998, one representative from the predominant major manufacturer of 50/125 m m fiber cores indicated that they commonly ship a 500/500 MHz-km product. In 1999, presentations to the 802.3 committee regarding 10-Gigabit Ethernet suggested that 50/125 m m fiber with a bandwidth of 2500 MHz-km may be possible.

Table 11.1. Standard Attenuation and Modal Bandwidth Specifications for Dual Wavelength Fiber, Adapted from IEC 793-3, with Additions

Fibertype	Alb(62.5/125 m m)		A1a(50/125 m m)		Units
Transmission wavelength	850	1300	850	1300	nm
Attenuation categories (max)	3.5	1.5	2.7	1.0	dB/km
	3.2	0.9	2.5	0.8
	3.0	0.7	2.4	0.6
Modal bandwidth categories	160	200	200	400	MHz-km
	160	500	200	600
	200	200	400	400
	200	400	400	600
	200 [1]	500 [1]	400	800
	200	600	400	1000
	250	1000	400	1200
	300	800	400	1500
			500 [2]	500 [2]
			600	1000

[1] NOTE(1) ”specified in the ISO/IEC 11801 generic cabling standard, but not part of IEC 793-3.

[2] NOTE(2) ”A common specification, according to the predominant manufacturer of 50/125 m m fiber, but not part of IEC 793-3.

Can you use these new cables in your design? That depends on your application. A sufficiently compelling application could cause a customer to rip out old wiring and install something new. More likely, though, you will need to work on the old, installed base of cabling.

You will occasionally hear the term numerical aperture used to describe fiber. This term refers to the light-gathering ability of the fiber. All other things being equal, a fiber with a greater numerical aperture will generally accept more light from an LED source. Mathematically, the value of numerical aperture is a function of the refractive index profile, roughly equal to .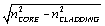

You will rarely have to specify the numerical aperture, because it is already specified in an international standard, IEC 793-2 (1992) [86] . Section A of IEC 793-2 calls out mechanical dimensions and numerical aperture values for several standard sizes of graded-index multimode fiber cores. Designers of high-speed systems need only ask for multimode optical fiber from IEC 793-2, category A1a (50 m m) or A1b (62.5 m m).

POINT TO REMEMBER

• Internationally recognized specifications for 50 and 62.5 m m multimode optical fibers are provided by IEC 793-2.

 

11.5.4 What Considerations Govern the Use of 50-micron Fiber?

Fifty-micron multimode fiber is generally available with a higher bandwidth and better transmission characteristics than 62.5- m m multimode fiber. This natural advantage is tempered by the fact that many data transmission products don't couple as well into 50- m m fiber as into 62.5- m m fiber. The coupling issue has to do with the construction of LED transmitting sources.

LED transmitters, which are normally used with multimode fiber (62.5 or 50 m m), produce divergent light beams. These transmitters may be classified into two broad categories: surface emitters and edge emitters .

A surface-emitting LED produces a beam spot generally large enough to overfill the front end of a 62.5- m m fiber (Figure 11.13). The beam power is calibrated to produce the correct transmitted light power when coupled to a 62.5- m m fiber. When the same transmitter is used with a 50- m m core, the smaller core intersects a smaller fraction of the transmitter's projected beam. It therefore picks up less power than the 62.5- m m core. A 50- m m fiber coupled to a surface-emitting LED suffers a power budget loss of approximately 2 dB to 5 dB, depending on the beam geometry.

Figure 11.13. Surface-emitting and edge-emitting LED beam widths.

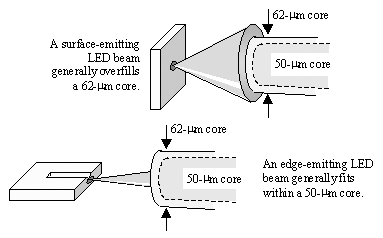
An edge-emitting LED produces a beam spot that generally fits within either a 62.5- m m or 50- m m core. It couples approximately the same power into either fiber type. There is no 50- m m penalty associated with edge-emitting LED transmitters. The edge-emitting LED produces a superior beam, but, like many things in life, you gotta pay for it. Edge-emitting sources are generally more expensive than surface-emitting sources.

To better support the use of cheap 62.5- m m sources, many customers in North America install 62.5- m m fiber. [100]

[100] The 50-mm versus 62.5-mm issue is part of a larger marketing war that rages constantly among cabling manufacturers.

POINT TO REMEMBER

• Fifty-micron multimode fiber has a generally higher bandwidth and less attenuation than 62.5- m m multimode fiber. These advantages are counterbalanced by the fact that some common LED sources can't couple efficiently into 50- m m core.

 

11.5.5 Multimode Optical Performance Budget

The optical performance budget for a fiber-optic link is a list of all known factors that affect link performance. It adds up the worst case for each effect and verifies that the system will still function under worst-case conditions.

The performance budget is one of the first places customers look when they want to lay blame for a system that doesn't work. If the customer's fiber meets the stated attenuation budget, but your system doesn't work, then in the mind of the customer any problems that arise will begin to seem like your fault . This issue cuts both ways. If you can prove that the customer's fiber doesn't meet its specification, you get to point the fickle finger of fate squarely at the cable installer. Make sure you have a performance budget and that it is accurate. [101]

[101] I'm not kidding about this. Some customers will initiate legal action against your company for a failure to perform.

A good optical performance budget should address three main areas: dispersion, attenuation, and jitter. Template budgets for all three areas appear in the following sections. These templates follow the same pattern used for the development of FDDI, Ethernet, and Fibre-Channel LAN standards.

These templates were developed for glass multimode fiber links operating at distances between 100 m and 1 km, and at speeds between 100 Mb/s and 1000 Mb/s. If your application is significantly different from that, you should spend some time carefully checking each of the assumptions used in these models to see which might not apply.

This brief chapter cannot possible hold enough information to satisfy the needs of an optical component designer. That is not my intent. Rather, I merely hope to define the most commonly used terms, show the relations between the various parameters, and prepare you as a digital designer to read an optical specification sheet, understand the terms used, and properly apply a fiber-optic transceiver in your next system design. Towards that end, let's look first at the causes of dispersion in fiber data links.

11.5.5.1 Multimode Dispersion Budget

An optically perfect, crisp rising-edge input to the front end of a long fiber emerges at the other end somewhat smaller and with a degraded risetime. The purpose of dispersion calculations is to determine the extent of risetime degradation and to estimate the impact that degradation will have on signal reception . I'm going to review the dispersion calculations very carefully, because they are riddled with assumptions that must be understood in order for the calculations to apply. You will need the following basic information (Table 11.2) to compute dispersion.

Table 11.2. Parameters Required for Dispersion Calculations

Name	Meaning	Units
t s	Source rise/fall time	ps
t b	Source baud interval	ps
t w	Clock window at receiver	ps
B m	Fiber modal band width	MHz-km
l	Fiber length	km
l RMS	Source RMS spectral width	nm
D	Fiber chromatic dispersion constant	ps/nm-km
If you don't have access to D , you will need the next three parameters:
l	Zero-dispersion wavelength	nm
S	Dispersion slope	ps/nm 2 -km
l c	Source center wavelength	nm

Figure 11.14 depicts four test points used to define dispersion. Point TP1 represents the electrical interface just prior to the LED or laser driver. This interface is commonly a differential low-level signaling interface. The choice of signal levels is arbitrary. [102]

[102] Although ECL (or PECL) was for a long time the best choice, in 1999 it began being supplanted by LVDS and other low-voltage signaling schemes.

Figure 11.14. Block diagram showing critical points for jitter analysis.

Point TP2 represents the optical signal entering the fiber-optic cable plant. This is commonly defined at a point on the far side of the transmitter connector if the transmitter is directly connectorized or on the far side of the first connection placed at the end of the transmitter pigtail if the transmitter is a pigtail type. In some extraordinary circumstances ”for example, when a special form of patch cord is required at the driver ”point TP2 is defined at the end of a suitable patch cord. [103]

[103] That is the approach taken in Gigabit Ethernet.

Point TP3 represents the optical signal at the conclusion of the fiber-optic cable plant at the point where it enters the receiver. The receiver comprises an optically sensitive diode receiver (PIN diode or APD diode), a low-pass filter [104] (to cut down the level of white noise from the diode), and a limiting amplifier (a comparator).

[104] Most receivers comprise a chain of several limiting-amplifier stages, each with a finite bandwidth. The number of stages of amplification required to boost the signal to a fully saturated level is a function of the incoming signal amplitude. The low-pass filter in this diagram represents the composite bandwidth of those stages which, at the minimum signal amplitude, operate in the linear mode. The comparator then represents the operation of the remaining stages, which operate in a fully saturated mode. A well-designed receiver heavily restricts the bandwidth of the first stage, but leaves the others wide open , so that the effective receiver bandwidth of the resulting amplifier chain does not vary with signal amplitude.

Point TP3b represents a circuit internal to the receiver. This circuit is generally not available for direct testing, although its characteristic may be determined by indirect means. This node is after the low-pass filter but before the limiting amplifier.

Point TP4 represents the electrical interface just after the limiting amplifier (comparator) in the receiver but before the sampling circuit (flip-flop). This interface is commonly a differential low-level signaling interface.

The specific problem addressed by the dispersion budget involves the degree of intersymbol interference present at point TP3b. The precise amount of intersymbol interference at point TP3b varies as a function of the optical risetime at TP2, the effects of the fiber as measured at TP3, and the effects of the low-pass filter inside the receiver. The intersymbol interference reduces the usable signal amplitude at point TP3b, rendering the system more susceptible to internal noise generated within the optical receiver. If the usable signal amplitude is sufficiently reduced, bit errors may result.

The purpose of the dispersion budget is to allocate some permissible degree of dispersion among the source, fiber, and receiver in a way that will provably limit the worst-case intersymbol interference at TP3b .

Dispersion calculations are generally made using the following assumptions:

• The source risetime (or fall time, whichever is worse) at TP2 is known independent of other factors.
• The fiber performs a linear, time-invariant operation on the transmitted signal.
• The receiver detection diode is both linear and instantaneous in its operation, converting received optical power to an electrical voltage.
• The low-pass filter performs a linear, time-invariant operation on the transmitted signal.

Under these assumptions, we may conclude that the complete signal at point TP3b, and thus the intersymbol interference, may be completely predicted by convolving together

1. The source signal at TP2,
2. The impulse response of the fiber,
3. The gain of the diode, and
4. The impulse response of the low-pass filter.

Given a sufficiently powerful calculation tool, you could convolve together these waveforms and directly compute the intersymbol distortion. There is, however, a generally accepted shortcut that can save you a lot of time in the calculations. It is based on some additional assumptions, which I should be very careful to state clearly. This shortcut-calculation procedure does not work in all systems, only in those which conform to the following:

• The source signal rising edge is monotonic (so is the falling edge).
• The step response of the fiber is monotonic.
• The step response of the low-pass filter is monotonic.
• All rising and falling edge waveforms are Gaussian.

In fiber-optic systems, the aforementioned signal conditions are generally true, and under those conditions the following approximation describes the risetime at point TP3b.

Equation 11.4

 

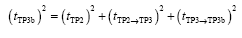
where	t TP3b is the 10% to 90% risetime at point TP3b,
	t TP2 is the 10% to 90% risetime at point TP2,
	t TP2 TP3 is the 10% to 90% risetime of the fiber, and
	t TP3 TP3b is the 10% to 90% risetime of the low-pass filter.

We'll use equation [11.4] to estimate the signal risetime, and from that the intersymbol interference, at TP3b.

I'd like to draw your attention for a moment to the use of the Gaussian assumption in equation [11.4]. The Gaussian assumption is also used to translate the signal risetime at TP3b into an equivalent amount of intersymbol interference. It is crucial that you understand that this assumption, while fairly reasonable for most fiber-optic systems, does not apply to copper-based transmission systems. In the copper -based world you are faced with a skin-effect impulse response which has long, significant, non-Gaussian tails . In a copper system, you really need to explicitly convolve together the whole system response to accurately predict what intersymbol interference will develop. In the fiber case, we can take the shortcut of making the Gaussian assumption.

Equation [11.4] is derived by first recognizing the relation between the risetime of a Gaussian step response and the area under the corresponding impulse response (see [88] , and Figure 11.15). [105] The 10% to 90% points on the step response correspond in time to the 10% and 90% cumulative area points on the impulse response. That is, 80% of the area under the impulse response should lie between the 10% and 90% points on the step-response curve. If we knew the standard deviation s of the impulse response waveform, and if the pulse were truly Gaussian, then the exact width which encompasses 80% of the area should equal ±1.28 s .

[105] I should point out that the concept of RMS risetime makes sense only for signals that are monotonic, that is, lacking any form of ringing or other non-monotonic behavior.

Figure 11.15. Relation of step response to impulse response (for a positive, integrable impulse response with finite variance).

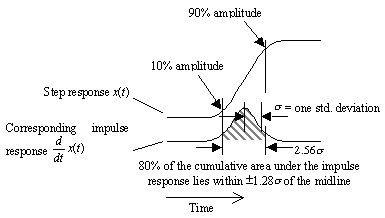
For every Gaussian waveform there exists this basic equivalence between risetime of the step response and the standard deviation of the corresponding impulse response: The 10% to 90% risetime equals 2.56 times the standard deviation.

If the risetime is proportional to the standard deviation, then the risetime squared should be proportional to the standard deviation squared (variance) of the impulse response. That's the form in which equation [11.4] may begin to make sense to you. It's a theorem about the variance of little impulses. It says, "When convolving impulse functions, their variances add."

The proof of this familiar fact appears in every elementary textbook on the subject of probability theory. You'll find that in probability theory this theorem applies to the determination of the variance of a sum of random variables , whereas here we look to determine the variance of a convolved chain of impulse responses, but it's the same theorem either way. Variances add.

To operate equation [11.4] you will need to know the risetimes of the component pieces. The risetime of the source t TP2 is easy: That's the 10% to 90% risetime called out on the datasheet for the optical driver.

The 10% to 90% risetime of the fiber is a little more involved, being a function of two types of fiber bandwidth: modal and chromatic. For a typical multimode fiber application the overall risetime is closely approximated by

Equation 11.5

 

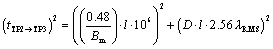
where	the assumed impulse of the fiber is approximately Gaussian,
	t TP2 TP3 is the 10% to 90% risetime contributed by the fiber, ps,
	B m is the 6-dB (electrical) modal bandwidth of the fiber (FWHM bandwidth), in MHz-km,
	l is the length of the fiber, km,
	D is the chromatic dispersion constant, ps/nm-km,
	l RMS is the RMS spectral width of the source, nm (the RMS spectral width is the standard deviation of the curve of power density versus wavelength), and
	2.56 is a constant that converts standard-deviation-type pulse width measurements to 10% to 90% measurements.

The value of the chromatic dispersion constant D varies with wavelength. For some fibers, you will find a chart that directly shows D as a function of wavelength. Other fibers are specified in terms of a zero-dispersion wavelength and dispersion slope from which you are expected to estimate D , like this:

Equation 11.6

 

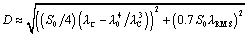
where	D is the chromatic dispersion constant, in ps/nm-km,
	S is the dispersion slope parameter at wavelength l , in ps/(nm 2 -km),
	l c is the source center wavelength, in nm,
	l is the zero-dispersion wavelength, in nm, and
	l RMS is the RMS spectral width of the source, in nm.

Equation [11.6] is the dispersion formula accepted for use by the IEEE 802.3z Gigabit Ethernet committee in 1998. At the time it was generally acknowledged by the working members of that committee to be the most accurate closed-form approximation available for the estimation of chromatic dispersion.

Equation [11.6] is appropriate for use with normal or dispersion-shifted fiber. It properly estimates the high-order dispersion effects that happen with wide-spectral-width sources near the dispersion null. It also works with narrow-spectral-width sources. It works in both 850 and 1300 nm windows. This formula is not appropriate for use with dispersion-flattened single-mode fiber.

The 10% to 90% risetime of the low-pass filter is a function of the bandwidth of low-pass filter and the various preamplifier circuits internal to the receiver. These bandwidths are typically specified in terms of a 3-dB roll-off frequency. For a typical multipole, critically damped roll-off, most receiver filters will have a risetime reasonably approximated by

Equation 11.7

 

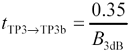
where	the equation assumes the impulse of the filter is not quite as good as a Gaussian response, [106]
	t TP3 TP3b is the 10% to 90% risetime of the low-pass filter, s, and
	B 3dB is the frequency (Hz) at which the filter gain falls 3 dB short of the gain at DC.

[106] A perfect Gaussian filter would have a risetime equal to 0.338/BW 3dB .

Now you have all the numbers necessary to apply equation [11.4].

Given t TP3b , and assuming the overall response is Gaussian, you may use the following general expressions to represent the cumulative effect of source, fiber, and receiver filter, where the variable s equals the standard deviation of the cumulative impulse response, equal to t TP3b /2.56. The following expression takes into account the shape of the received waveform, but does not model the bulk transport delay of the fiber.

Equation 11.8

 

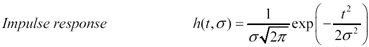
Equation 11.9

 

Equation 11.10

 

where	h ( t ) is the system impulse response, as a function of time t ,
	g ( t ) is the system step response, as a function of time t .
	The function erf 2 () is defined in Appendix E,
	H ( w ) is the transfer function from TP2 TP3b, as a function of frequency,
	w is the frequency of operation, rad/sec, and
	s is the RMS pulse width of the impulse response, equal in this case to t TP3b /2.56, in units of seconds (NOTE: t TP3b was previously computed in units of ps ”here you must translate it to units of sec).

You may recognize the function H ( w ) as a linear-phase transfer function, meaning that the step response will have equal length, symmetrical tails on the leading and trailing edges. This transfer function will not much affect the positions of the zero crossings of the received waveform, but it will create ISI whenever the tails from one transition have not been given sufficient time to dissipate before the center of the next bit.

Procedures for modeling the overall system response, given the function H ( w ), appear in Chapter 4, "Frequency-Domain Modeling."

From the risetime T TP3b you can predict the worst degradation in received amplitude likely to occur under any conditions due to intersymbol interference (ISI). This requires that you know the worst-case data pattern for intersymbol interference. That pattern, for any system with monotonic rising and falling step edges, is a single 1 preceded and followed by a long run of zeros (or the complement of this pattern).

Equation 11.11

 

From knowledge of the system step response we may write an expression for the exact waveform y BAD ( t ) expected under the worst-case-ISI condition, namely, a single step going high at time t followed by an opposite step at time t + t B , where the constant t B represents the data link baud interval:

Equation 11.12

 

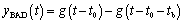
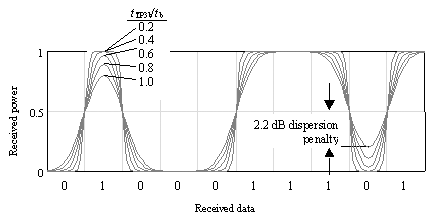
Figure 11.16 shows examples of various dispersion-induced ISI waveforms calculated in this manner. What counts is the degree to which y BAD (t) exceeds the switching threshold at the moment your sampling circuit makes its decision. In order to estimate the degrading effect of dispersion, separate from all other factors, one assumes the received waveform is of unit size, the sample is taken precisely halfway between the two step transitions at time t + t b /2, and the threshold is adjusted precisely midline (at 1/2). Under those conditions the sampled amplitude (above the threshold) would be

Figure 11.16. Received optical signal with varying amounts of Gaussian dispersion.

Equation 11.13

 

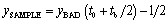
Plug in the definition of y BAD [11.12].

Equation 11.14

 

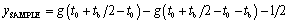
Simplify terms.

Equation 11.15

 

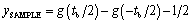
Adapt the definition of g ( t ) from [11.9].

Equation 11.16

 

Apply the definition of s .

Equation 11.17

 

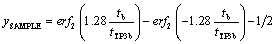
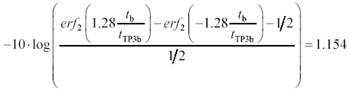
Equation [11.17] is usually converted to a dB penalty, expressing the ratio of two quantities : first, the sampled amplitude above the threshold in [11.17] assuming dispersion T TP3b , and second, the nominal sampled amplitude that would have been received had there been zero dispersion (1/2 in this case).

The traditional approach for all penalty calculations is to express them as a received optical power penalty, using 10 times the logarithm of the received power ratio, recognizing that the received optical power is converted linearly by the optical receiver into a received voltage. An optical power penalty of 3 dB implies that the received voltage has been cut in half.

Equation 11.18

 

The above expression for the dispersion penalty p D is plotted against the parameter t TP3b /t b in Figure 11.17, where t TP3b is the total 10% to 90% dispersion in seconds and t b is the baud interval in seconds (or both in ps). The dispersion penalty represents the loss of eye opening expected in the received data due to the interaction of one bit with the next. The ratio t TP3b /t b defines the dispersion in units of unit intervals where one unit interval is understood to be one baud interval.

Figure 11.17. Dispersion penalty in dB versus the degree of dispersion, 10% to 90%, measured in units of bit intervals.

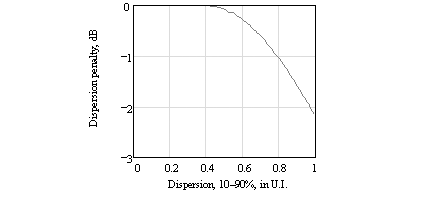
In many systems a limit is placed on the worst-case magnitude of the dispersion penalty. Typical dispersion limits are set at 2 or perhaps 3 dB. With 3 dB of dispersion penalty, the eye at top dead center is half closed by intersymbol interference. Beyond 3 dB of dispersion, the system performance becomes extremely sensitive to dispersive effects, as the received eye pattern begins to completely close.

Any fiber-optic data link that conveys an acceptable amount of power to the receiver, but for which the degree of intersymbol interference (dispersion) prohibits reliable communication, is called a dispersion-limited link. Increasing the transmitter power in such a link will not improve performance. On the other hand, a link with an acceptable degree of intersymbol interference (dispersion), but which is otherwise limited by the total amount of received power, is called an attenuation-limited link.

After consideration of the dispersion penalty, any jitter or uncertainty in position of the clock further degrades the received amplitude at the precise moment of sampling. The additional degradation as a function of clock position could be computed by using an equation like [11.13] to compare the worst-case sampled power at one edge of the clock window ( A 2 in Figure 11.18) to the sampled power at the center of the clock window ( A 1 ). Alternately, you may assume that the received signal under worst-case conditions takes on the appearance of a half-sinusoid within each baud interval, leading to the following more commonly-used approximation for what is called the clock window penalty :

Equation 11.19

 

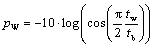
where	t w is the full width of the clock uncertainty window (assumed to be symmetrically located about the baud center as in Figure 11.18),
	t b is the full width of each baud interval, and
	p W is the clock window penalty, in decibel units of received optical power, evaluated at the edges of the clock window.

Figure 11.18. The received amplitude at the edges of the clock window is never as great as in the center of the eye.

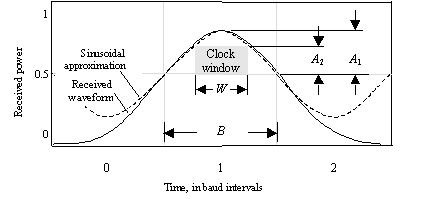
The preceding assumption is a pessimistic assumption. It always overestimates the clock window penalty. The clock window penalty may be improved only by narrowing the window of clock uncertainty through the use of better clock-recovery circuitry .

To the extent that there is any duty-cycle distortion present in the transmitted signal, we must adjust the dispersion calculations. Duty-cycle distortion is caused by asymmetries in the turn-on and turn -off time of the optical source. It is a specification of the optical source. In cases where the duty-cycle distortion is significant it is customary to take the minimum ON or OFF duration permitted by the duty-cycle specification and use that value for t b in the calculation of the dispersion and clock window penalties. For example, in FDDI the transmitter duty-cycle distortion is specified as no more than 1.00 ns peak-to-peak. That would mean that the worst-case baud duration must lie within the range of 8.00 ns +/ “ 0.50 ns, for a minimum of 7.50 ns. The value 7.50 ns should be used in all FDDI dispersion calculations.

The parameters p D and p W comprise the output of the dispersion-estimation process. These values feed into the signal attenuation budget discussed in the next section.

Example Calculation of FDDI Dispersion Penalties

NOTE: The following values represent typical datasheet parameters. These values do not reflect the worst-case parameters permitted by the FDDI standard.

Specifications
Source rise/fall time	t s =3500	ps,10 “90%
Source baud interval(min)	t b =7500	ps
Clock window at receiver	t w =2000	ps
Fiber modal band width	B m =500	MHz-km
Fiber length	L =2	km
Source FWHM spectral width	l FWHM =140	nm
Source RMS spectral width		nm
Zero-dispersion wave length	l 0,min =1300 l 0,max =1350	nm
Dispersion slope	S =0.11	ps/nm 2 -km
Source center wavelength	l c,min =1320 l c,max =1360	nm
LPF bandwidth	B LPF =87.5	MHz

Derived Quantities
LPF risetime		ps 10 “90%
Dispersion constant		ps/nm-km
Fiber risetime		ps 10 “90%
Overall risetime		ps 10 “90%
Dispersion penalty p D		dB optical
Clock window penalty p W		dB optical

POINT TO REMEMBER

• Dispersion calculations determine the extent of risetime degradation and estimate the impact that degradation will have on signal reception.

 

11.5.5.2 Multimode Attenuation Budget

The purpose of the attenuation budget (also called the power budget) is to allocate some permissible degree of attenuation among the long fiber cabling, the short fiber jumpers , and the connectors in a way that will provably limit the worst-case minimum received signal at TP3 .

A proper attenuation budget takes the form shown in Table 11.3. The budget begins by listing the output power available from the transmitter and the input power required at the receiver. The difference between these two entries is the optical power budget , or available power budget , in the optical link. The other entries represent various attenuating factors present within the link. These entries are appropriate for evaluating a multimode glass-fiber link with an LED transmitter. (See also Section 11.5.9, "Multimode Fiber with Laser Source," and Section 11.6.1, "Single-mode Signal Propagation.")

The sum of all attenuating factors should (hopefully) be less than the available power budget. The power margin represents the excess power budget remaining after subtraction of all known deleterious effects.

The values in Table 11.3 represent typical datasheet parameters for FDDI components. These values do not necessarily reflect the worst-case parameters permitted by the FDDI standard.

Table 11.3. Multimode LED Optical Power Budget

Line	Item	Value	Total	Units
1	TX power (min)	“20		dBmW
2	Guaranteed RX sensitivity	“31		dBmW
3	Power budget (line 1 less line 2)		11	dB
4	Cable losses	4.0		dB
5	Connector and splice losses	2.0		dB
6	Dispersion penalty p D	1.2		dB
7	Clock window penalty p W	0.4		dB
8	Extinction ratio penalty	0.0		dB
9	Other penalties	n/a		dB
10	Total losses and penalties (sum of lines 4 “8)		7.6	dB
11	Power margin (line 3 less line 9)		3.4	dB

NOTES

TX power. The guaranteed worst-case (minimum) output power specified under the worst combination of power supply voltage, temperature and aging conditions.

RX sensitivity. A level above which all receivers are guaranteed to operate with an acceptable BER, under worst-case conditions, including local self-generated crosstalk from any nearby transmitter circuits within the same package, VCC noise, etc.

Cable losses. Typically 2 to 10dB per kilometer for glass fiber. This example assumes 2 km at 2dB/km.

Connector and splice losses. Remember to provide for several connections so you can have patch panels and jumper cables. This example budget permits four connectors at 0.5 dB each.

Dispersion penalty. See "Example Calculation of FDDI Dispersion Penalties." In some systems the receiver input sensitivity is defined under a specific test condition which imposes a worst-case signal with artificially generated dispersion. In such a system, if the test conditions accurately reflect the real-life worst-case dispersion, you could assume that the dispersion penalty has already been accounted for within the receiver sensitivity specification.

Clock window penalty. See "Example Calculation of FDDI Dispersion Penalties."

Extinction ratio penalty. For systems that transmit equal numbers of ones and zeros (that's the normal case for a fiber-optic link), the peak-to-peak transmitted power is nominally twice the average transmitted power. The peak-to-peak power is what activates the receiver; the average power is what we read on a power meter during product testing or installation. If the transmitter does not go completely dark during the transmission of a logical zero, the peak-to-peak power will be somewhat smaller than twice the average power. The difference between the actual peak-to-peak power and the nominal value is expressed here as the extinction ratio penalty . The extinction ratio E expresses the ratio between the zero -state power and the one -state power, while the extinction ratio penalty in optical dB equals 10log((1- E )/(1+ E )). The extinction ratio penalty for LED transmitters is often negligible.

Other penalties. Other penalties apply to laser-based links. See Section 11.5.9, "Multimode Fiber with Laser Source."

Power margin. The purpose of margin is to cover your posterior in case you forgot anything or were overly optimistic about any parameters. A 3-dB margin is highly desirable. Any finished system that incorporates a 3-dB optical margin will prove very robust in the field. Technicians will routinely be able to exceed its guaranteed link distances, eating into the margin without fear of catastrophic link failure. A big margin keeps your customers smiling. [107]

[107] In the initial stages of product planning, you should insist on an even greater margin (perhaps 6 dB). As your design progresses and you learn more about the behavior of optical components, there will be many heated arguments about the budget, and the margin will always diminish.

POINT TO REMEMBER

• An attenuation budget allocates attenuation among the long continuous runs of fiber cabling, the short fiber jumpers, and the connectors in a typical installation.

 

11.5.6 Jitter

Every practical communication system is affected by jitter . Simply put, jitter is the amount by which each individual rising or falling edge deviates from its ideal temporal position.

A large amount of jitter, something large enough to push individual data transitions into the clock window, will obviously cause bit errors. A smaller amount of jitter, while not enough to cause dramatic errors, can still induce system failure if it interferes with the operation of the PLL-based clock recovery subsystem.

In fiber-optic transmission systems it is common to divide the measured jitter into two components, the deterministic jitter and the random jitter . The deterministic jitter represents the misplacement of received edges due to any repeatable, data-dependent phenomenon . The most important property of deterministic jitter is that it is bounded . That is, there is a certain amount of it, and it never gets any worse.

One common measurement procedure for deterministic jitter uses a repeating data waveform that includes a variety of different run-lengths, both high and low. An ideal waveform would be something like this: [10101111000011110000 10101111000011110000...]. This waveform includes a rapid 1010... pattern, and also some slower 11110000... patterns. Such a repeating waveform must be observed , averaged over many repetitions to eliminate random effects, and analyzed to determine the precise position of each zero crossing . Such an analysis reveals the worst-case peak-to-peak deterministic jitter. Frequent causes of deterministic jitter include asymmetry in the rise/fall time of the optical source, the non-ideal modal step response of the fiber, and phase nonlinearity in the receiver filter.

The random jitter is then calculated as the difference between the observed total jitter and the measured deterministic jitter. The reason we bother separating these two effects is that total jitter is usually measured at some nominal working point on the jitter histogram. A comfortable probability-of-occurrence level for jitter measurement in high-speed systems is about p =10 “6 . In other words, the measurement gives us a jitter number and tells us that the jitter will exceed this number only one time out of 10 6 . That's nice, but it isn't what we need to know. What we usually seek is a jitter number that will be exceeded only one time in, say, 10 12 . To calculate that number, we can extrapolate the random jitter component, assuming it follows a Gaussian probability distribution from 10 “6 to 10 “12 , and then add back in the deterministic jitter to estimate the total jitter at a probability level of 10 “12 . Extrapolation of the random jitter distribution is done according to the chart in Figure 11.19.

Figure 11.19. The extrapolated random jitter magnitude at a probability-of-error level of 10 “12 exceeds the measured value at 10 “06 by a factor of 7/4.8 = 1.46.

POINT TO REMEMBER

• Fiber-optic transmission systems commonly divide the jitter budget into deterministic jitter and random jitter .

 

11.5.7 Multimode Fiber-Optic Noise and Interference

Fiber-optic links use photons , not electrons. As a result, fiber-optic cables emit zero electromagnetic energy within the FCC or EN regulated bands. Fiber-optic links operate free from interference due to radio waves, power-line noise, lightning, and all other electromagnetic effects. Fiber links may run near power lines and other communication lines without fear of interference. They may be bundled tightly together without limit. Fiber is perfect for communication between buildings .

Any noise problems you encounter with fiber will come from the receiver circuitry itself, from local crosstalk within the receiver package or from the printed-circuit board or power system to which the receiver connects.

The self-noise generated within a high-speed fiber-optic receiver is true, wide-bandwidth white Gaussian noise (WGN). It's caused partly by thermal noise and partly by quantization noise (shot noise) within the detection diode and preamplifier circuits. In a good receiver this is the primary source of noise. Cooling down a receiver usually improves its sensitivity.

A low-pass filter in series with the detector output reduces the level of noise, but at the expense of additional signal dispersion. Most receivers attempt to set the low-pass filter cutoff somewhere around 0.7 /t b , where t b is the baud interval. Extremely high-speed receivers, because they cannot afford the dispersion that comes with low-pass filtering, have to operate in the presence of high levels of noise. Such receivers are generally less sensitive than lower-speed receivers.

Direct electrical crosstalk between the local optical transmitter and the optical receiver is another factor that can affect performance. This crosstalk is exaggerated when the local transmitter and receiver are located close together. The worst case for local crosstalk happens when the receive fiber in a two-fiber system is disconnected or when the far end is powered off. In either case the receiver sees no light. When that happens, the receiver's AGC circuit usually turns up the receiver gain as high as it will go, straining to hear any faint signals on the incoming fiber. If the gain goes up too high, the receiver picks up electrical crosstalk from the local transmitter. I've seen protocols that come alive and start talking to themselves when that happens, thinking there is a "live" device at the other end.

Appropriate solutions to the local crosstalk problems include

1. Limiting the maximum receiver gain,
2. Implementing a minimum-signal-level detection circuit to shut off the receiver in the event of a dark input, or
3. Reducing the crosstalk.

Regarding emissions, even though fiber itself doesn't radiate, beware of the fiber-optic transmitter. The transmitter is a fairly high-powered device mounted near a hole in your cabinet shielding. Potentially high levels of direct radiation can emanate from the optical transmitter.

POINT TO REMEMBER

• Fiber cabling may be immune to crosstalk and RFI, but your fiber-optic receiver is not.

 

11.5.8 Multimode Fiber Safety

One thing you should never do with a fiber is look into the end of it. Most fiber communication systems use infrared light. The human eye cannot see it, but it is very, very bright. The surface brightness over the tiny active cross section of the fiber can rival that of an oven heating element on full broil. While it is true that there are no internationally recognized safety guidelines for multimode LED emitters, it is still never a good idea to look directly into the end of a fiber.

This author will always remember the warning he found posted near the transmit port of one early fiber-optic transmitter: "Do not look into this orifice with your remaining good eye."

POINT TO REMEMBER

• Never look into the end of a fiber.

 

11.5.9 Multimode Fiber with Laser Source

LAN standards for ATM, Fibre Channel, and Ethernet all advocate the use of laser-diode transmitters on multimode fiber. These standards attain speeds of approximately 1 Gb/s, at distances ranging up to several hundred meters .

Laser-diodes enjoy many advantages over LED sources. Good laser-diodes have a faster risetime, a greater switching frequency, and a higher optical power. Laser-diodes also possess a narrower spectral width, which dramatically reduces chromatic dispersion. As far as traditional measures of optical performance are concerned , laser-diodes appear better in every respect.

The major areas where laser-diodes are not better than LED sources include cost and modal effects. The cost issue is, for the moment, pretty glaring. Some manufacturers hope that VCSEL technology (see Section 11.5.10, "VCSEL diodes") will reduce the cost of their laser optics, but it will probably still never achieve the rock-bottom pricing of a simple surface-emitting LED.

The modal effects are very complex and deserve serious discussion. The problem here is that laser-diodes depend on some subtle, undocumented, and unspecified features of multimode fiber. That's not a good situation.

Multimode fibers are designed for illumination with a broad-beamed source like an LED. The LED induces an overfilled launch condition , meaning that all modes of propagation in the fiber are filled with light. [108] If the fiber manufacturer has done the job correctly, the propagation delays for most of the modes will be closely grouped. Notice that I did not say all the modes, but rather most of the modes. Since there are so many modes, and the power splits among them, no individual mode carries very much power. If there are a few bad modes with radically different delays, it really doesn't hurt anything. When confronted with all the modal power that does arrive together, the receiver will never notice a tiny amount of errant , bad -mode power that arrives late (or early).

[108] An overfilled launch condition does not carry equal amounts of power in each mode; however, all modes are used.

This description may give you an idea about how to improve the bandwidth performance of multimode fiber. What if you could craft a source that broadcast power only into the really good modes, the ones with the best natural speed-matching? Such a miracle source might then enjoy the benefits of reduced modal dispersion. That would be wonderful, but no one knows how to do it reliably and cheaply with practical fibers.

What the industry does know how to do is how to craft a source that concentrates most of its power into a few very bad modes. This kind of source emphasizes the worst-than-worst case modal dispersion problems of those bad modes. A fiber illuminated with this kind of bad -mode source may exhibit modal dispersion in excess of the normal specified limits.

One way to create a really bad -mode source is to aim a very narrow-beam laser-diode straight down the center of a multimode fiber. This couples power into only a few modes of propagation, mostly centered in the middle of the fiber. It turns out that the manufacturing processes used to create multimode fiber often leave imperfections in the dead center in the fiber that are highlighted by this type of source.

Precisely this scenario happened to the Gigabit Ethernet committee in 1997. The committee at that time was trying to develop a multiuse 1300 nm source that would work on either single-mode fiber or multimode fiber. The SMF requirements mandated that the narrow-beam laser-diode source be very accurately centered. When this source was coupled to MMF, the pinpoint accuracy of the source constituted an almost perfect bad -mode source, with anomalous results. Eventually, the committee found ways around this difficulty [93] .

Another problem with the use of laser-diodes on MMF relates to the modal structure of a laser source. Laser-diodes radiate fewer optical modes than do LED sources. For the purposes of this discussion you may imagine one mode as a beam of light shining in a particular direction with a particular wavelength. [109] Contrary to popular belief, laser-diodes do not produce a single beam of light at one wavelength. A laser-diode actually radiates in hundreds of modes at once, at a multitude of wavelengths, spewing out the front facet of the laser at a variety of angles. There are a lot of modes, but not an infinite number. The modal structure is discrete and measurable.

[109] Here I use a ray-tracing analogy for the modes. This analogy, while not perfect, is a useful way to mentally visualize the radiated modes.

An LED, on the other hand, operates more like a light bulb. It emits light over a continuous range of wavelengths, with an even and continuous spatial distribution.

The modal structure of the laser-diode wouldn't really matter if all the modes were always present, all the time, with consistent amplitudes, but that isn't how they work. Some modes carry a lot of power, some very little. Also, sometimes the amplitude of one specific mode pops out of whack suddenly. This happens particularly in connectors if an air gap is present between the coupled fibers. It also happens in the fiber itself if the fiber is wiggled. When the amplitude of one mode changes, if you happen to have too many eggs in that one modal basket , the received signal glitches unpredictably. The term for this effect is modal noise . An LED is not subject to a modal noise penalty, because it uses a continuum of modes. With an LED source, the loss of any one particular mode is too insignificant to affect the remaining power in the optical signal.

As the laser-diode turns on, the distribution of modes changes. In the early stages of oscillation it may emit at one wavelength and later at a slightly different one. Also, the spatial distribution changes. If the fiber propagation delay for the early modes and the late modes are slightly different, you get another strange type of signal distortion. The term for this effect is mode partition noise . Gigabit Ethernet was among the first standards to incorporate mode partition noise to its power budget [93] . An LED source is not subject to a mode partition noise penalty.

The third weird effect associated with lasers is reflected input noise (RIN). A laser-diode depends on an intricate set of mirrored facets to do its work. When other mirrors are introduced into the system, it can mess up the lasing action. In a multimode system there may be connector facets downstream that reflect significant amounts of noise back to the source. With an LED source these reflections cause no difficulties. With a laser-diode source the reflections cause sporadic shifts in the laser's operation. These shifts and clicks have been characterized and a measurements procedure crafted to measure the susceptibility of a laser to reflections. The test procedure is specified in ANSI X3.230-1994 annex A, subclause A.5. [110]

[110] IEEE 802.3z: "For use with MMF applications the polarization rotator referenced in the ANSI X3.230-1994 should be omitted, and the single-mode fiber should be replaced with a multimode fiber."

My advice to you about the use of laser-diodes on MMF is simple: It can be done, but it can be dangerous. If you must go down this road, copy the specifications from an existing standard (preferably one that ships in high volumes ).

POINT TO REMEMBER

• The use of laser-diodes on multimode fiber depends on subtle, undocumented, and unspecified features of the multimode fiber.

 

11.5.10 VCSEL Diodes

The term VCSEL stands for vertical- cavity surface-emitting laser . The key difference between this form of laser-diode and any other (like a typical CD-ROM laser) is the orientation of the emitted beam. In an edge-emitting laser the beam comes out the side of the die; in a VCSEL it comes out the top surface. The VCSEL beam orientation is the same as that used in an ordinary surface-emitting LED.

The edge-emitting configuration was the first approach used to make successful laser-diodes. With this approach, the diodes must be diced apart, polished, and tested to weed out the bad components (Figure 11.20). A certain amount of effort is wasted polishing and handling parts that turn out ultimately to be dead lasers.

Figure 11.20. Edge-emitting diodes must be diced, polished, and individually tested to see if they are any good.

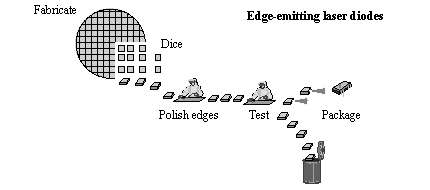
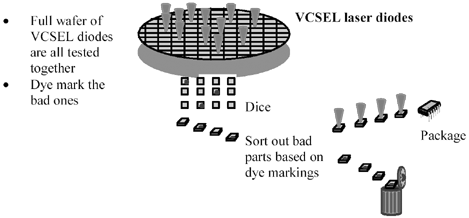
The surface-emitting configuration eliminates some of the waste, because the diodes can be tested en masse as they sit on the wafer, prior to dicing (Figure 11.21). After testing, the diodes are dye marked to record the results of the test, and the parts may then be diced and packaged in the ordinary way. The difference in fabrication process is responsible for the claims of lower cost associated with VCSEL technology.

Figure 11.21. The VCSEL fabrication process eliminates the polish-and-test cycle.

POINT TO REMEMBER

• A VCSEL shines perpendicular to its top surface, just like a surface-emitting LED.

 

11.5.11 Multimode Fiber-Optic Connectors

There continues a high degree of thrash in the fiber-optic connector arena, mostly because no one has yet designed a satisfactory, easy to install, inexpensive fiber-optic connector. Nor is anyone expected to do so in the near future.

Fortunately, if your customer's building site has the wrong connectors, you can always cut the fiber and put on different ones. Normal installation practices for fiber almost always leave a large loop of fiber at either end of a connection for just this purpose. That leaves you free to select just about any connector you want for your system. The three fiber-optic connectors most prevalent in building backbone wiring appear in Table 11.4, and Figure 11.22.

Figure 11.22. Widely-installed fiber-optic connectors.

Of the three connector styles in Table 11.4, the duplex SC has the smallest footprint. [111] It is a duplex connector, meaning that both transmit and receive fibers are terminated in a single keyed plastic connector assembly. At the time the Gigabit Ethernet LAN standard was finalized, ISO 11801 had designated the duplex SC as the connector of choice for future facilities designs. The duplex SC was selected as the only connector authorized for use with that standard. This is an excellent choice for all high-speed systems.

[111] Footprint is the amount of pcb space, or front panel space, consumed by the connector.

The ST connector remains popular in older Ethernet installation on 10 and 100 Mb/s equipment. Two ST connectors are required per port, one for the transmit circuitry and one for the receive circuitry.

Table 11.4. Common Fiber-Optic Connectors

Duplex SC (also called duplex SCFOC/2.5)	EIA/TIA 604-3 , and IEC 874-14	ISO-preferred connector for future facilities designs
ST (also called BFOC/2.5)	EIA/TIA 604-2	Separate connectors for TX and RX
FDDI fiber-MIC	ISO/IEC 9314-3	Unique to FDDI equipment

The FDDI MIC was developed exclusively for FDDI. The advent of 100 and 1000 Mb/s Ethernet backbone standards may obliterate the FDDI MIC.

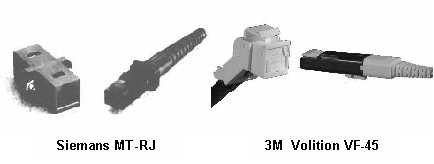
Upcoming new fiber-optic connectors will focus on ease of assembly and reduced footprint. It's not clear which will turn out to be the better connector, but two connectors that show promise are the 3M Volition VF-45 (standardized by ANSI/TIA/EIA 604-7) and the Siemans MT-RJ series (see Figure 11.23).

Figure 11.23. New fiber-optic connectors are about the size of an RJ-45 data plug.

You have probably heard speculation about the use of optics for backplane connections. Someday that will become commonplace. Optical backplanes certainly have an EMC advantage, and it's true that the pricing is coming down. Unfortunately, the cost of a transceiver pair is still stratospheric compared to the cost of one pin on a Euro-connector. Within a system, optics just can't compete economically. Carefully check the pricing and availability for both transceivers and optical connectors before you proceed with any optical backplane architecture. Optics work well for intersystem connections, but I've not yet seen a cost-effective optical backplane.

POINTS TO REMEMBER

• No one has yet designed a satisfactory, easy to install, inexpensive fiber-optic connector.
• Optics work well for intersystem connections, but I've not yet seen a cost-effective optical backplane.