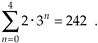Appendix B. Closed Form of a Geometric Series
In the literature of digital signal processing, we often encounter geometric series expressions like
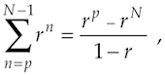
or
Equation B-2
Unfortunately, many authors make a statement like "and we know that," and drop Eqs. (B-1) or (B-2) on the unsuspecting reader who's expected to accept these expressions on faith. Assuming that you don't have a Ph.D. in mathematics, you may wonder exactly what arithmetic sleight of hand allows us to arrive at Eqs. (B-1) or (B-2). To answer this question, let's consider a general expression for a geometric series such as
Equation B-3
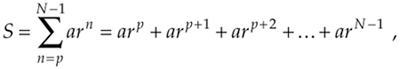
where n, N, and p are integers and a and r are any constants. Multiplying Eq. (B-3) by r, gives us
Equation B-4
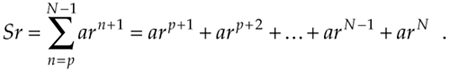
Subtracting Eq. (B-4) from Eq. (B-3) gives the expression
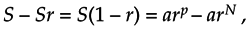
or
Equation B-5
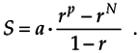
So here's what we're after. The closed form of the series is
Equation B-6
(By closed form, we mean taking an infinite series and converting it to a simpler mathematical form without the summation.) When a = 1, Eq. (B-6) validates Eq. (B-1). We can quickly verify Eq. (B-6) with an example. Letting N = 5, p = 0, a = 2, and r = 3, for example, we can create the following list:
|
n |
arn = 2 · 3n |
|---|---|
|
0 |
2 · 30 = 2 |
|
1 |
2 · 31 = 6 |
|
2 |
2 · 32 = 18 |
|
3 |
2 · 33 = 54 |
|
4 |
2 · 34 = 162 |
|
The sum of this column is |
Plugging our example N, p, a, and r values into Eq. (B-6),
Equation B-7
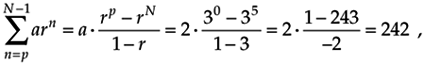
which equals the sum of the rightmost column in the list above.
As a final step, the terms of our earlier Eq. (B-2) are in the form of Eq. (B-6) as p = 0, a = 1, and r = e–j2pm/N.[
] So plugging those terms from Eq. (B-2) into Eq. (B-6) gives us
[
Equation B-8
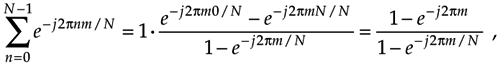
confirming Eq. (B-2).
URL http://proquest.safaribooksonline.com/0131089897/app02
 |
Amazon |  |
