3.11 Discovering Computing Abilities
|
3.11 Discovering Computing Abilities
How do we find reaction-diffusion or excitable media to fulfill our "computational dreams" in very real wet-ware? There is not much choice at the moment. Regarding oscillating chemical reactions, there are dozens of them and most reactions look quite similar, hence almost everybody mainly experiments with BelousovZhabotinsky media. The advantage of such ubiquity is the chance to verify each other's experiments. At the molecular level, the situation is not as good: We can fabricate molecular arrays, but there exist almost no reports on any feasible computing experiments, either with "classical" waves or with mobile self-localizations.
Which problems can be solved in what types of nonlinear media? Should we fabricate these media from scratch or could we instead search for already existing species in nature? In one of the author's papers (Adamatzky 2001), the reader can find a study of which behavioral parameters of a medium's local elements are essential when classifying morphological, dynamic, and computational aspects of excitable lattices. In this chapter, we would rather provide an example demonstrating an impressive potential arising from very simple parameterization.
A threshold of excitation seems to be the most suitable parameter in the case of excitable media. Unfortunately, knowing the excitation threshold does not give us a complete description of space-time excitation dynamics. To discover richer regimes of the dynamics and thereby find new computing abilities, we can "upgrade" the excitation threshold to an excitation interval. In a lattice with local transition rules and an eight-cell neighborhood, a cell is excited if the number of excited neighbors belongs to a certain interval [φ1,φ2] 1 ≤ φ1 ≤ φ2 ≤ 8. Using excitation intervals, we classify the lattice excitation regimes and select those suitable ("suitability" being decided based on our previous experience and intuition) for wave-based computing. As we see in figure 3.9, the evolution of excitable lattice from senseless quasi-chaotic activity patterns to the formation of protowaves and then proper labyrinthine-like structures is guided mainly by the upper boundary of the excitation interval [φ1,φ2]. When the boundary φ2 is increased, the computation capabilities of the medium are changed from chaos to a wave dynamic that implements image processing (contouring, segmentation, filtration) then to a quasiparticle dynamic that supports universal computation (via colliding localizations; see previous sections on universal computing) then to the solution of computational geometry problems (shortest path, spanning tree) (Adamatzky 2001).
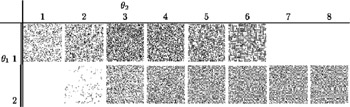
Figure 3.9: Morphology of interval classification.
We would like to stress that the excitation interval discussed in the previous section may be analogous to (1) Langton's ƛ parameter (Langton 1990), which opened a new field of research in complex systems and emergence of computation; (2) temperature in the lattice swarm models of hot sand (Adamatzky 2000a; an abstract temperature determines transitions between various types of two-dimensional patterns); and (3) laboratory experiments with a nonstirred Belousov-Zhabotinsky reaction (Masia et al. 2001); where the increasing temperature evokes mode transitions from periodic oscillations to quasi-periodic oscillations to chaos.
|