Synthesis Building Blocks: Virtual Analog Techniques
Early analog instruments were based on what could be easily accomplished with electrical circuitry . Surprisingly, that hasn't made the way they work any less relevant in the digital realm.
First, of course, there's the desirability of analog sounds, which have had a profound impact on the course of music over the past few decades. Analog techniques are essential for reproducing those familiar sounds, but they're important for other sound production tasks as well.
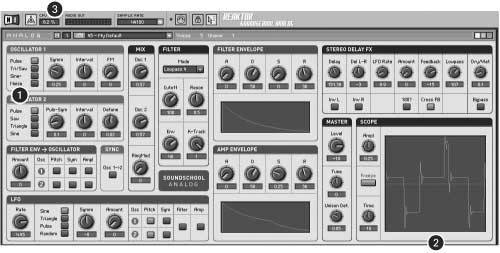
The simple waveforms, filters, and modulators of analog synthesis make excellent basic building blocks for sound production in general. Just as a child's building blocks set tends to incorporate simple, geometric forms like cubes and cylinders , analog synthesis gives us basic geometric sounds (like the sine and square wave) that can be combined into more complex constructions. As a result, you'll find the same elements, concepts, and interfaces from vintage analog synthesis on sophisticated digital synths. You'll use analog-style envelopes, filters, and modulation on instruments that use sampled audio as wave sources instead of analog-style waveforms like sine, saw, and square waves. Most instruments follow the basic structure shown in Figure 9.19 .
Figure 9.19. The basic signal routing structure of virtual analog instruments is identical to the vast majority of synths, including digital synths. Even though the analog-style oscillators may be replaced with other signal sources and synthesis techniques, many of the basic modulation, filters, and effects will be similar.

Just reading about synthesis is a fairly fruitless endeavor, since it's the sound result in which we're interested. For that reason, in the discussion on the following pages you'll want to follow along with Native Instruments' Reaktor SoundSchool Analog, included on the DVD. (See the sidebar, "Hands-on: SoundSchool Analog.")
| | Disclaimersimulated analog sound: Virtual analog synthesizers can reproduce the basic interface, working method, and sound of their analog forbearers. Behind the scenes, though, they use digital algorithms to replicate the function of analog circuitry, so technically they're not analog. Synthesis pioneer Bob Moog claimed that even the virtual analog instruments that bear his official endorsement don't sound as good as his true-analog hardware. You can let your own ears be the judge, but be aware that for the purpose of this section, although the techniques are basically the same as they would be on real analog equipment, the sound is digitally generated. |
Basic Waveshapes and Timbre
Harmonics in sound and the basic waveshapes were discussed in Chapter 1, but now we'll use these principles in music making.
Analog circuitry easily generates simple waveforms via basic, repeating electrical patterns. The simplest circuits, for instance, simply turn voltage on and off, like a repeating light switch, producing a signal called a pulse wave (after the on and off pulses created) or a square wave (describing what the signal looks like on an oscilloscope).
The different waveshapes produced can be either listened to or used as modulators (for altering other signals). When a wave comes from an audio oscillator, the different waveshapes have different harmonic content, giving each of them a different sound. Oscillators in a synth can almost always be switched, so that a single oscillator can produce any of several different waveshapes. (On vintage analog oscillators, this switch was typically in the form of a dial for selecting different waveforms. Now that switch is an onscreen setting, often in a pop-up menu.)
|
As discussed in Chapter 1, real-world instruments all produce sounds containing sound energy at different frequencies. A sound vibration at a particular frequency is called a partial. The words "harmonic" and "overtone" have similar meanings, but the frequency of a harmonic is always a whole-number multiple of the frequency of the fundamental , which is the frequency we hear as the base pitch of the note. For example, a fundamental at 100 Hz would have harmonics at 200 Hz, 300 Hz, and so on.
The relative strengths of the various partials contribute to what we hear as timbre (tone color ). Different waveshapes have different combinations of harmonics, from the sine wave, which contains only a fundamental tone with no overtones, to the sawtooth wave, which contains all the overtones (although higher overtones are progressively weaker). White noise (which is also available as a waveform on many oscillators, although some synths have a separate noise source instead) contains not only all of the harmonically related overtones, but all of the possible partialsin theory, an infinite number. A noise signal contains sound energy at all frequencies. Oscillators of these wave types can be combined with one another, or filtered, so that you hear different harmonic content for additional timbral possibilities. Let's begin by taking a look at (and listening to) the waveshapes most often used in analog synthesis.
|
Sine wave
Sine waves ( Figure 9.22 ) are the most basic of the basic waveshapes: they're pure tones, free of overtones. As a result, their sound is plain vanilla . Since we're more accustomed to hearing sounds with partials at various frequencies, sine waves sound quite dull on their own. They're often used as test tones for calibrating audio equipment and software. Some acoustic instruments make sounds that resemble a sine wave, like the tone of a glockenspiel, a flute playing without vibrato, and other pure-tone instruments.
Figure 9.22. The sine wave is a pure tone, and sounds very muted.
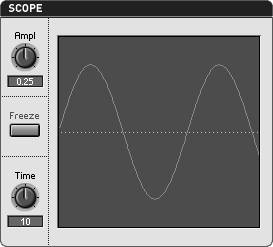
| | Sine wave Harmonic content: No overtones What it sounds like: Plain, similar to a flute without vibrato or breath noise; you've heard it in test tones Why it's useful: Can be used in combination with other sine waves (or other signals) to form more complex tones Try it: SoundSchool preset 28Basic Sine |
|
Although they're not as useful alone, used in combination with other waves, sine waves are essential to synthesis. They're the basis, for instance, of both additive synthesis and FM synthesis, both of which utilize sine waves to form more complex sounds.
Triangle wave
Triangle waves ( Figure 9.23 ) are similar to sine waves, but do not have a rounded shape. As a result, they add harmonic content (higher overtones) along the lines of a square wave. The harmonics in a triangle wave are much weaker than those in a square wave, however.
Figure 9.23. The triangle wave has a mellow sound with a few weak harmonics.
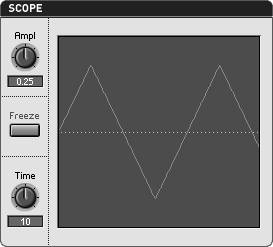
| | Triangle wave Harmonic content: Odd harmonics; higher harmonics are very weak What it sounds like: Muted, like the sine wave, but with slightly more color Why it's useful: Used for basses and leads; combined with other waves Try it: SoundSchool preset 29Basic Tri |
Sawtooth wave
Sawtooth waves ( Figure 9.24 ) don't just look like the teeth on a saw. Because of the rich set of harmonics, they sound buzzy and "saw-like" as well. They're rich enough in sound that many people use them as is for bass sounds. They're also the classic basis for subtractive synthesis, so-named because filters are used to remove some of the harmonic content from a sound. Sawtooth waves are often compared to the sound of a trumpet : just listen to a high-pitched sawtooth wave and add a lot of imagination .
Figure 9.24. Sawtooth wave (note the slight rough edge at the corners; a mathematically perfect waveform will have precise corners, but that isn't always desirable).
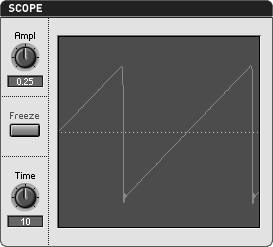
| | Sawtooth wave Harmonic content: All harmonics; higher harmonics are progressively weaker What it sounds like: Rich, fat, and buzzy; the classic analog synth bass sound Why it's useful: Rich and strident on their own, saw waves can be filtered to produce subtler timbres with less harmonic content Try it: SoundSchool preset 27Basic saw |
|
Square wave
Like a sawtooth wave, the square wave's upper harmonics (see Figure 9.26 ) are relatively strong. Unlike the sawtooth wave, however, the square wave has only odd-numbered harmonics, giving it a full, rich, but slightly hollow sound. It lacks the buzz of the sawtooth's even-numbered harmonics. In its middle range, you'll find a square wave sounds reminiscent of a clarinet played without vibrato.
Figure 9.26. Square wave (left); if the width of the wave narrows (right), the wave is called a pulse wave and has different timbral qualities (it contains all harmonics, but less energy at the fundamental).
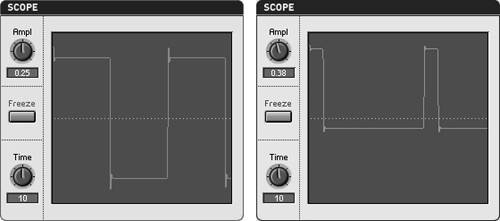
| | Square wave Harmonic content: Odd harmonics; higher harmonics are relatively strong (as with a sawtooth wave) rather than falling off rapidly in energy (as with a triangle wave) What it sounds like: Rich but rounded, hollow; very similar to the sound of a clarinet playing without vibrato Why it's useful: Used for basses and leads; combined with other waves Try it: SoundSchool preset 26Basic Square |
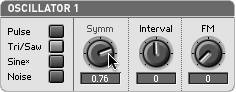
Preset 26 in SoundSchool is the most common, basic square wave. True to its name, the dimensions of the wave are square: the signal spends as much time in the "up" position as in the "down" position. If we call the "up" position a pulse, the width of each pulse is equal to the width between pulses. SoundSchool, like many analog synths, lets you adjust the width of the pulse. Hold down a note and try increasing the value of the Symm knob. The width of the pulse will gradually get narrower, and you'll hear a thinner sound. (With a narrow pulse width, the sound is similar to the Hohner Clavinet, the electric keyboard popularized by Stevie Wonder.) As you narrow the pulse, the energy of the fundamental decreases, and the wave acquires more energy in the high harmonics. A narrow pulse has all of the harmonics, not just odd harmonics as with a square wave.
SoundSchool calls the parameter for the width of the pulse "symmetry," but most synths refer to it as pulse width or duty cycle . Some allow continuous adjustment, whereas others require you to choose from preconfigured widths. Later in this chapter, you'll use this parameter in modulation, which is naturally called pulse width modulation .
| | Narrow pulse waves versus square waves: All square waves are pulse waves, but not all pulse waves are square waves. As the width of a square wave narrows (so it's no longer square), it is called a pulse wave. Narrow pulse waves have different harmonic content than square waves. Harmonic content: All harmonics (instead of only odd) Fundamental: Less energy than a square wave High harmonics: More energy than a square wave |
Envelopes
At its core , a keyboard is nothing more than a row of buttonsvelocity-sensitive buttons , to be sure, but essentially the performer is limited to switching notes on and off. A simple electronic sound, like the beep on your microwave oven, fits into that basic design: it turns on, you hear sound; it turns off, the sound stops. But the sounds of instruments we love have always been more complex.
An acoustic piano, for instance, also has a keyboard that acts like a series of buttons: press one, and a lever strikes a string. Release the key, and a damper ends the sound. The keyboard of a 9- foot Steinway concert grand is still an on/off device: the sound is anything but. There's the sharp, striking attack as you hit a note, shifting harmonic content after the note is struck; the gradual, dying sound as that sound fades away; and with the release of the note, the tapered damping as a felt damper deadens the vibration of the string.
In short, acoustic instruments produce sounds that change over time. Synthesis needs a way of shaping sounds that's more sophisticated than a binary on/off system. From early analog synthesis to the latest software synths, electronic instruments have used envelopes to model changes over time.
What's an envelope?
An envelope (see Figure 9.27 ) is a representation of how a parameter of a sound, such as loudness, changes over time. In synthesis, envelopes are created by envelope generators, which are dedicated modules in the synth that output control signals. The control signals are routed to whatever is being controlled. The envelope generator makes no sound; it simply controls something else. Imagine you're conducting a cellist with your hand, raising your hand to coax more volume from the player and lowering it when you want the player to back off. Your hand is the equivalent of the control signal from the envelope generator: it produces an envelope (the shape of the volume you want over time), which the player translates into the volume of the sound (the actual amplitude).
Figure 9.27. What we refer to as a synthesizer envelope is simply a change in the level of a control signal over time. This is usually charted as an X/Y graph with time as the x-axis and control level as the y-axis. If the envelope shown were used to control amplitude, the synth's sound would quickly get louder and then somewhat softerthe attack portion of the note (1), then sustain at a fixed level (2), then fall back to silence (3), as shown here both in an envelope (left) and the waveform it approximates (right).
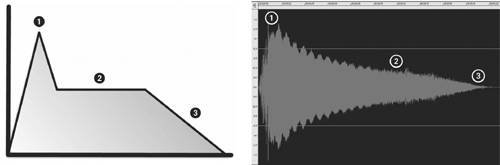
The most common use of an envelope generator is to control amplitude, but many synths give you other options as well. Unless you're using modular software, you'll usually choose a prerouted destination for the envelope generator to control, then adjust the shape of the envelope. SoundSchool Analog has a typical envelope generator configuration: one for amplitude and one for the filter. The filter envelope can also be routed to pitch, symmetry, or the amplitude of either or both of the oscillators. Each of these routings can be used for different effects.
Envelope shapes
Theoretically, an envelope can be any shape you want, but many synths, inspired by early analog designs, use a standard four-parameter envelope design. For this design, you can thank Columbia-Princeton Electronic Music Center head Vladimir Ussachevsky, who suggested to Bob Moog that a four-parameter system would be the best way to generate note shapes similar to those made by real instruments, from violins to drums. Many musicians have agreed, and the system has remained popular.
On the original Moog Modular, the four settings were labeled T1, T2, T3, and sustain. Today they are called attack, decay, sustain, and release, or ADSR. You'll see those four knobs on the SoundSchool Analog, with a graph of the resulting envelope below them. The Moog labels are helpful in that three of the settings (T1, T2, and T3, or attack, decay, and release) control the amount of time that passes between one envelope level and another when you press or release a key, whereas the sustain parameter controls the level at which the envelope stays for as long as you hold down the key. Figure 9.28 shows what happens when you press a key and the synth's envelope generator responds.
Figure 9.28. This image shows what happens when you press a key down on your synth in relation to your synth's envelope generator controls.

You press a key.
The level rises from zero to the maximum level over a span of time (the attack time).
The level falls (or "decays") to the sustain level over a span of time (the decay time).
The level stays at the sustain level for as long as you hold down the key.
You release the key.
The level falls back to zero over a span of time (the release time).
Each of the points along the envelope is called a breakpoint . Some digital synths allow you to design more complex envelopes by adding breakpoints wherever you like. You can use a multisegment envelope to create sophisticated rhythms . For everyday playing, though, ADSR envelopes are versatile enough to cover many of the sound designs you'll need.
Hands-on: ADSR and amplitude
| |
Let's try working with these different knobs and see how they impact sound. Load SoundSchool preset 26Basic Square. Notice that the Amp Envelope is simply a big blue rectangle: this is an on/off envelope. The attack is 0 (instantaneous) and the sustain is 1 (full level). The decay knob happens to be set to 57, but that's irrelevant, because the decay setting affects the sound only when the sustain level is lowered . Try pressing and releasing a key. The sound turns on, then turns off; its amplitude goes from zero to maximum almost instantly and returns to zero almost instantly. Looking at the sustain knob, you can see why: the sustain amplitude is at its maximum level, 1. Press a key and, while you're holding it down, adjust the sustain knob. The amplitude of the waveform changes, just as it would with a conventional volume knob.
| | Tip: To keep your square waves from overlapping one another, set Voices (at the top of the screen) to 1, converting SoundSchool into a monophonic synth. Otherwise, notes sounding at the same time will interfere with one another on the Scope. |
Set the sustain knob to about .3 and the decay knob to about 50, and you'll create a basic decay/sustain envelope. As you play notes now, each note will decay from its full amplitude to the sustain amplitude and continue sounding until you release the key. You can see this happening by watching the Scope: the amplitude of the note (the height of the square wave) decreases from the time you press down the key until the sustain level is reached as you hold down the key.
| | Mario, anyone ? If this walkthrough gives you flashbacks to video games , it's because Nintendo's original console and Game Boy used basic square waves and noise oscillators. If you like the effect, try reducing the sample rate and using an LFO for vibrato (see the discussion of LFOs later in the chapter). |
The notes still begin and end abruptly because the attack and release knobs are set to 0. (The A, D, and R knobs in SoundSchool display time in ms; as you'll see if you turn on tips and mouse over the knob: 0=1 ms, 20=10 ms, 40=100 ms, and so on. The value 0 isn't actually 0, but 1 ms is close enough to zero to sound instantaneous to our ears.)
If you increase the attack knob to 30, the beginnings of the notes will sound less sharp and more "rounded" as the sound gradually increases to maximum amplitude with each note you strike. For a gradual attack, try a value of 50 or more. That sounds a little strange with this plain square wave, so return the knob to a more realistic setting of 20. At that level, the note still has a fairly sharp attack, but there's an audible beginning to the sound.
Just as the attack knob determines how quickly the sound rises to maximum amplitude after you hit a key, the release knob determines how long it takes for the sound to return to zero after you let go of the key. (This is potentially confusing terminology because musicians commonly call this property the "decay" of a pitch, but in synthesis terms, release happens after you let go of a key.) Try increasing the knob to 40 or more, and you'll hear the note continue to sound after you release the key. Decrease it to 25 for a subtler effect.
You've just created a typical envelope for pitched instruments that sustain pitch, like woodwinds. But what about sounds like percussion, which have fast, brief attacks? They can be modeled with ADSR envelopes, but the envelopes will have a different shape.
Open preset 30Basic Noise. Initially, pressing a key sounds like turning on static on your TV. To make this sound more like a snare drum, you'll need to mimic what happens when you hit a snare: there's a brief initial attack, then the sound quickly delays. Decrease the sustain value to 0 (to mimic the drum's damping effect) and the decay to 40 to create a steep envelope. (For slightly more realistic attack and release, try values of A=8 and R=40.) The sound is primitive, but reminiscent of a snare hit, and is a result of manipulating simple noise with a basic amplitude envelope. (If it sounds familiar, this effect was used in early video games for drums; you'll create richer-sounding percussion later when using filters.)
To see how envelopes are used in practice, you can browse through some of the presets included with SoundSchool; see Table 9.1 .
Table 9.1. Common Amplitude Envelope Types
| Although their timbre is unmistakably analog and electronic, these sounds are reminiscent of common instruments because of their envelopes. (These are classic envelope shapes for these kinds of instruments, so expect to see similar envelopes on other synths.) | |
| | 35Basic Sub Bass has a sharp attack, which you'd expect of a plucked electric bass. |
| | 72Gittar also has a sharp attack, but decays quickly like an acoustic guitar or harp. |
| | 56Sreng sounds vaguely like a bowed cello, due to a gentler attack. Adjust it to make it slightly more realistic: increase the attack to 40 and nudge the release to 45. |
| | 75Tomm mimics the sound of a drum by eliminating the sustain entirely and decaying quickly. Try small adjustments to the D knob on the Amp Envelope to make this tom more muted (shorter decay) or more resonant (longer decay). |
|
Mixing Oscillators
So far, we've been using only one oscillator at a time, limiting us to basic waveform choicessquare, triangle, sawtooth, sine, and noise. That's fine for some sound designs, but fairly vanilla.
By mixing oscillators, you can create richer sounds. SoundSchool provides two oscillators that can be mixed together in the Mix section ( Figure 9.30 ). Many synths have four oscillators or even more, but the two included in SoundSchool allow for most basic techniques.
Figure 9.30. Adjust the balance of SoundSchool's two oscillators in the Mix section.
The simplest way to use two oscillators is by detuning one from the other. When two identical or similar waveforms are not perfectly in tune with one another, natural phasing produces a more complex waveform. Let's watch and hear it.
Hands-on: Mixing and detuning
| |
Load preset 28Basic Sine. Look at the Mix section: by default on this preset, you hear only SoundSchool's second oscillator. The Osc 2 volume is turned all the way up (1), but Osc 1 is set to silent (0). While holding down a note, turn up the volume for Osc 2. Since both are sine waves and are set to the same frequency, the two waveforms will overlap exactly, so you'll get the same sound but twice as loud. (If that doesn't happen, the two oscillators are out of sync: check the Sync Osc 1 > 2 check box and then uncheck it again to place them back in phase.)
With the two oscillators in phase, one waveform is added to the other, as shown in Figure 9.31 . (Watch the amplitude of the wave on the Scope.) If they're detuned, the waveforms will move in and out of phase. Adjust the detune knob on Oscillator 2 and listen while watching the Scope. As the waveform shifts out of phase, it produces a pulsing sound as it cancels out the sound from Oscillator 1.
Figure 9.31. With two identical sine waves in phase, combining two oscillators just produces a wave with twice the original amplitude (left). When one wave is detuned from another, the result is a new waveform that changes over time. Try combining two sawtooth waves and adjusting the detune and Symm/Puls-Sym knobs to create a richer sound (right).
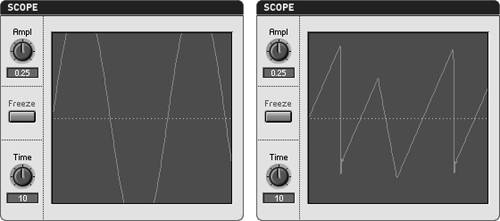
With sine waves, this effect isn't very interesting or useful. Load preset 26Basic Square. (Both oscillators are set to square waves in this patch.) Increase the level of Mix > Osc 2 to 1 (turning it all the way up). Now hold down a note and gradually turn the detune knob down for Oscillator 2, just slightly below 0 in the 00.03 range. Huge difference, right? We've gone from a boring electronic sound to a lead sound reminiscent of a distorted electric guitar. Let's take a look at what's going on: decrease the amplitude knob on the Scope so you can see the whole dynamic range of the waveform. As the two waves superimpose, they move in and out of phase with one another, alternately adding and cancelling one another out, creating a dynamically shifting range of overtones in the sound. With the Osc 2 mix set to 100%, the effect is similar to pulse width modulation, because the "stair-steps" appear above and below zero amplitude and shift in width. (For true pulse width modulation, you can assign the filter envelope or LFO to the symmetry of a pulse wave.) To adjust the resulting timbre, adjust the Puls-Sym knob of Oscillator 2, and try replacing the waveforms with Pulse and Saw, Tri/Saw and Saw, and so on.
Ring modulation
Other means of combining oscillators can result in some pretty far-out effects. By using the Osc 1 and Osc 2 controls under Mix, you're adding the waveforms. To hear what happens when you multiply waveforms, turn up the Mix > RingMod knob. RingMod, or ring modulation , is sometimes called multiplicative synthesis because it multiplies one signal by another. The net result of multiplying two signals is both the sums and differences of all of the overtones present in the two sources. (For more on ring modulation used as an audio effectwith any signal source, not just these common synthesized waveformssee Chapter 7.)
Theory aside, ring modulation is useful for clanging, sonorous timbres and special effects. Let's try one extreme example. You'll need two waveforms, since ring modulation requires two inputs. Select preset 26Basic Square, and turn up the RingMod knob all the way to 1. Note that you don't have to turn up the Osc 2 knob, because RingMod by definition uses both oscillators. In fact, turn down Mix > Osc1 so you hear only the ring-modulated output ( Figure 9.32 ).
Figure 9.32. For a pure ring-modulated sound, turn up RingMod while turning down the level of the oscillators.
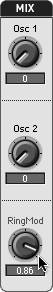
The two signals are the same at the moment, so change the pitch of one. Turn up the Interval knob on Oscillator 2 to 30. Now try hitting a note: you'll hear a variety of partials, the sum and difference pitches. Sounds horrible, right?
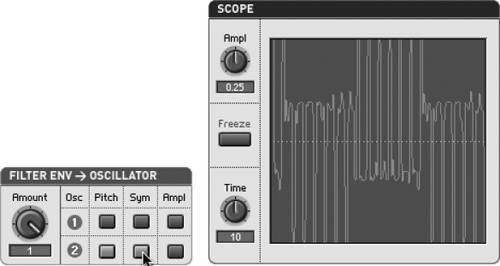
You can make the ring modulation more active by having one of the two signals sweep in pitch. Unlike the amplitude envelope, the filter envelope in SoundSchool can be routed to other parameters. Let's first set up the envelope shape: set the filter envelope's attack to 0, decay to 60, sustain to 0, and release to 30 for a gradual sweep from high to low. Under Filter Env  Oscillator, select the Pitch and Sym check boxes next to Osc 2 ( Figure 9.33 ). By selecting these boxes, you're routing the envelope generator to those parameters. So instead of the pitch and symmetry of the second oscillator staying where you put the knobs, they'll shift along the contour of the envelope as you hold down a note. Next, choose the amount of the modulation by increasing Filter Env
Oscillator, select the Pitch and Sym check boxes next to Osc 2 ( Figure 9.33 ). By selecting these boxes, you're routing the envelope generator to those parameters. So instead of the pitch and symmetry of the second oscillator staying where you put the knobs, they'll shift along the contour of the envelope as you hold down a note. Next, choose the amount of the modulation by increasing Filter Env  Oscillator > Amount to 1. (For a sweep in the reverse direction, from low to high, youd choose 1.)
Oscillator > Amount to 1. (For a sweep in the reverse direction, from low to high, youd choose 1.)
Figure 9.33. You've used envelopes to modulate amplitude; here you modulate the oscillator with the filter envelope using SoundSchool's Filter Env  Oscillator check boxes (left). The ring-modulated result multiplies the two signals, producing the waveform shown at right, while the envelope sweeps oscillator pitch and symmetry downward.
Oscillator check boxes (left). The ring-modulated result multiplies the two signals, producing the waveform shown at right, while the envelope sweeps oscillator pitch and symmetry downward.
Now hold down a note for a wild, sweeping, ring mod pulse wave. You wouldn't want to use this for anything other than a brief special effect, but keeping in mind how simple the ingredients were (two pulse waves and a single envelope), you'll likely agree that the result is more dramatic. (Fans of the British sci-fi hit Doctor Who will immediately recognize this sound as the screaming sound that opened the theme music during the '70s and '80s.)
You can also use subtler ring modulation to add timbral color to a patch. For example, load preset 85Clav. Try playing it with the RingMod knob at 0, then return the knob to the preset's value of 0.2. In this case, ring modulation is simply icing on the cake, turning a somewhat lifeless sound into a more biting one.
Oscillator sync and timbre
Oscillator sync synchronizes the period of two oscillators' waveforms, forcing the "slave" oscillator to have the same fundamental frequency as the "master" oscillator. Unlike what happens when beats are synchronized, you'll hear a timbral, not a rhythmic effect, since you don't hear individual periods of waves. So why would you want to synchronize waveforms? Oscillator sync produces some unique sound design twists that make it useful.
In SoundSchool, with oscillator sync turned on, Oscillator 1 is the master and Oscillator 2 is the slave. If you open a preset like 28Basic Sine, Oscillator 2 will sound by default. Turn sync on, and nothing audible happens. A sine wave operating at a regular frequency can be synced to another sine wave operating at the same frequency. It's the same effect as tuning two oscillators to the same pitch; nothing interesting happens. However, if you raise the frequency of the master oscillator, Oscillator 1, using its Interval knob, the slave oscillator, Oscillator 2, has to change its waveform to maintain the same frequency as the master. It gets only part way through its period before it must start over again ( Figure 9.34 ). The waveform produced will have a unique timbre as a result.
Figure 9.34. Oscillator sync adds another timbral option to your synth. With oscillator sync turned on (1), one waveform will be a slave to the wavelength of another. So a standard sine wave (2), for instance, will have to restart its waveform before it completes a full period (3).
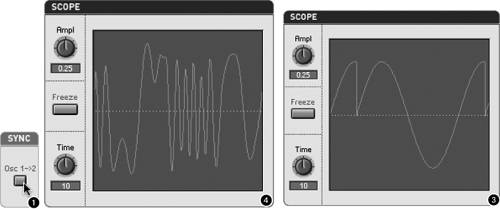
Why is this useful, other than for creating oddly shaped waveforms? If the pitch of your second oscillator is being modulated, synchronizing it to the first oscillator will maintain a prominent fundamental frequency while other overtones change.
The whole point of oscillator sync is to open up new timbral effects, so let's give it a listen. Load preset 40Basic Sync and play a note. The Filter Envelope controls the pitch of Oscillator 2, but the pitch is not just sweeping down from high to low as you'd expect. You hear the sweep, but you also hear a prominent fundamental tone below it. Looking at the Scope, you can see why this is happening: the waveform of Oscillator 2 is locked to the pitch of the pulse wave in Oscillator 1, and the fundamental pitch is preserved. You can change this fundamental pitch by adjusting the Interval knob on Oscillator 1.
By contrast, if you turn off Sync Osc 1 > 2, all you'll hear is the sweep with no fundamental. Watching the Scope, you'll see the width of the fundamental waveform shifting (which indicates why you're perceiving the fundamental pitch changing).
Frequency modulation (FM)
FM synthesis is often described as sounding metallic or bell-like. The reason is that the process has the perceived effect of adding harmonics. As the name implies, FM modulates the frequency of one oscillator with a signal from another oscillator. The reason FM synthesis differs from other forms of FM, such as vibrato, is that the modulating oscillator is in the audio range, the frequency range above 20 Hz. You need at least two oscillators to do FM, although some synths will give you four or more.
Like many virtual analog synths, SoundSchool focuses on subtractive synthesis but adds some basic FM. FM is hard-wired to the most common case: Oscillator 1 modulates Oscillator 2 via the FM knob ( Figure 9.35 ). In basic two-oscillator FM, the wave you hear as the output is called the carrier, whereas the wave modulating the carrier is called (oddly enough) the modulator . In SoundSchool, that makes Oscillator 2 the carrier and Oscillator 1, the oscillator with the FM knob, the modulator.
Figure 9.35. The one lowly FM knob in SoundSchool is the key to some timbres that are very different from other subtractive sounds discussed in this chapter. Oscillator 1 is the FM modulator, so you can turn down the Osc 1 level in the mixer (1). Adjust the harmonic relationship of the two oscillators with Oscillator 2's Interval and Detune knobs (2), and choose how much of an effect you want to have on the waveform of the carrier (that is, on the harmonic spectrum) with the FM knob (3). Watch the Scope (4) to see why you hear additional harmonics in the sound.
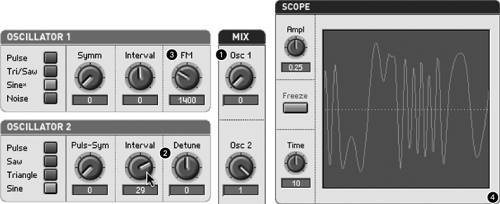
Load preset 39Basic FM for a typical FM patch that uses two sine waves. Notice that the designer opted for a bell-like amplitude envelope, and via a similar filter envelope routed to Oscillator 1, diminishes the FM effect as the pitch sounds. This mimics the decay of overtones in a bell or other resonant metal. (To hear the pure tone throughout, uncheck Filter Env  Oscillator > Ampl.)
Oscillator > Ampl.)
Using this preset as a template, you can experiment with some basic properties of FM:
-
Try changing the Oscillator 2 Interval parameter one increment at a time. As the ratio of the frequencies changes, there's a significant change in timbre.
-
For more complex timbres, adjust the Oscillator 2 detune parameter in conjunction with Interval. With detune set to 0, the two oscillators have a whole-number frequency relationship. Detuning sets up an inharmonic relationship that changes the timbre.
-
The FM knob increases the amount of effect the modulator (Oscillator 1) has on the carrier (Oscillator 2). Using this knob, you can try more radical changes: a higher value will generally sound more metallic and biting, while a lower value will sound more muted and bell-like. (To hear the difference clearly, adjust the control while the pitch is sounding.)
-
Different waveforms will give you different results. Substitute, for instance, two pulse waves for the sine waves.
-
Try additional filter and amplitude modulation as employed in this patch to further shape the tone.
Filters and Subtractive Synthesis
Sculptors work in two fundamental ways: they either start with a big block of stone and chip away, subtracting material until they have the shape they want, or they start with a material like clay, adding material to produce a finished form. Likewise, synth designers can start with a basic sound and add harmonic content or start with a harmonically rich sound and remove harmonics for the desired timbre. To put it more accurately, they can use frequency modulation and additive synthesis to strengthen the energy of certain partials, or they can use subtractive synthesis to weaken the energy of the partials within an existing sound.
| | More FM and subtractive synthesis: For more sound design tips that combine extensive FM synthesis with subtractive synthesis, open the demo tutorials for Ableton Operator, included with the Live demo on the disc. Work through the tutorials to see how classic FM and subtractive techniques can be used to build common sounds. Operator is a four-oscillator synthesizer in which the oscillators can be routed as FM oscillators, virtual analog oscillators, or both. |
To do the latter, subtractive synthesis uses filters, just as we used in Chapter 7. In SoundSchool Analog, you'll see familiar filter shapes: notch , lowpass, bandpass, and highpass . By feeding a sound source rich in harmonic content through this filter, you can adjust the timbre of the sound by weakening partials, most commonly with a lowpass filter.
But if the goal is to produce "richer" sounds, why would we want to weaken the harmonics? Isn't it "the more harmonics, the merrier?" Not necessarily : you might want to use filters for several reasons. First, you can use them to achieve specific timbral effects. You might want to weaken certain harmonics to create a more focused timbre. In addition, by adding resonance to the filter, you can accentuate certain partials while weakening others. Second, by using a filter in conjunction with a filter envelope, you can sweep through different harmonics, shaping sounds over time.
Let's take a look at how classic analog filtering works; then, see how that translates into music making.
Anatomy of a filter
As opposed to some of the more elaborate filters you learned about in Chapter 7, most filters used in synthesis are fairly simple. You'll use the cutoff frequency knob to zoom in on the part of the spectrum you want to attenuate, and then add resonance where you want to emphasize specific frequencies near the cutoff.
SoundSchool Analog offers a typical filter bank with a choice of fixed filter types (which SoundSchool calls "Modes"), Cutoff, and resonance ("Reson"). Separate controls adjust how much effect the envelope has on the filter over time (Env) and how much the cutoff frequency changes depending on where you play your keyboard (K-Track) ( Figure 9.36 ).
Figure 9.36. SoundSchool's filter controls.
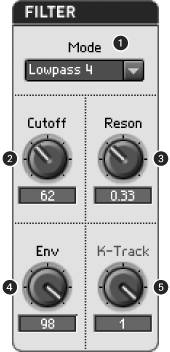
Mode: Selects a specific fixed filter type (lowpass, bandpass, etc.) and slope.
Cutoff: Determines the cutoff frequency for the filter; measure in semitones (60 is middle C).
Reson: Sets the amount of resonance, from no resonance to self-oscillation (1.0).
Env: Determines the extent to which the filter envelope changes the cutoff frequency, in positive or negative semitones.
K-Track: Short for Keyboard Tracking, determines the extent to which the filter cutoff is affected by what note is played.
Mode
Some synths have just one fixed-slope lowpass filter, in which case all you'll see are cutoff and possibly a resonance control. Most will offer you a choice by letting you adjust the filter type (lowpass, bandpass, highpass, and notch), slope (in db/Octave), or both.
In SoundSchool Analog, there is a number after each filter type in the Mode menu. These numbers refer to filters of different slopes: lowpass 1 is 6 dB per octave, lowpass 2 is 12 dB per octave, and so on. (See the explanation of slope in Chapter 7.) To find out which is which, turn on hints (View > Show Hints) and mouse over the Mode menu for a full description.
Cutoff frequency
The cutoff frequency setting is the most important to consider first. In fact, with some synths, if you have a lowpass filter with a cutoff of zero or a highpass filter with a maximum cutoff, you may not hear any sound at all. (SoundSchool's cutoff range is intentionally limited so that doesn't happen.) If you choose a lowpass filter with the cutoff set at the maximum value, for instance, you won't hear much or any filtering until you turn down the knob.
Hands-on: Resonance and self-oscillation
| |
Once you've adjusted the cutoff frequency, you can add a resonant peak just below the cutoff frequency by increasing the resonance parameter. Resonance is the amount of emphasis added around the cutoff frequency and ranges from no resonance (Reson=0, a standard, nonresonant cutoff filter) to maximum resonance (1.0). At maximum resonance, a phenomenon called self-oscillation occurs in some filters. As the filter approaches its highest resonance value or Q, instead of simply emphasizing frequencies around the cutoff, the filter oscillates at the cutoff frequency. Think back to the discussion of Q in Chapter 7: as the resonance filter increases, you're effectively adding a peaking filter around the cutoff frequency.
It's easier to understand resonance and self-oscillation by trying them out. (Warning: turn your speakers down first, since the result can be dangerously loud!)
-
Load preset 29Basic Tri. By default, this patch includes a lowpass filter, but the cutoff frequency is set to the maximum value of 140, so you can't hear it.
-
Reduce the cutoff frequency, and you'll gradually attenuate the harmonic content. Notice what happens: your triangle wave gradually turns into a sine wave as you reduce its upper harmonics.
-
Set Reson to 0.75 and move the cutoff knob around. You'll be able to hear an emphasis at the cutoff frequency. It sounds somewhat like an additional note sounding at that pitch. If you watch the Scope closely with Cutoff set just above its center position, you'll be able to see this frequency. Incidentally, if you've ever heard overtone chanting as practiced by singers of cultures like Tibet and Tuva, the sound is similar. These singers are able to use their mouths or throats as resonant filters.
-
Now try self-oscillation. (Don't forget to turn your speakers down first.) Increase Reson to 1.0 and again move the cutoff knob. The tone at the cutoff frequency is so distorted that the filter literally self-oscillates. If this effect is reminiscent of someone tuning a shortwave radio in an old movie, it's because that tuning circuitry had a tendency to self-oscillate.
Tracking filters to time and pitch
Filter type, cutoff, and resonance are all settings that remain stable in performance, affecting sound equally no matter what notes you play or how short or long the notes may be. To shape the way the filter behaves as a function of time (the length of time a given note has been sounding) or the note played on the keyboard, you'll need to use envelopes and keyboard tracking.
By using an envelope to modulate filter cutoff, you can sweep the cutoff frequency of the filter as the note sounds, making the sound's frequency spectrum morph as it plays for subtle or dramatic effects. In SoundSchool, you can adjust the amount of filter envelope that will be applied to the cutoff by setting the Env knob to either a positive or negative value. Set to positive, the Env setting increases the cutoff frequency as the envelope rises. When the Env control is set to negative, a rising envelope lowers the cutoff frequency. When this knob is set to zero, the envelope has no impact.
Keyboard tracking is significant, too. Normally, the filter cutoff remains at a fixed point regardless of what note you play. (If you're not using key tracking, double-check what the filter sounds like in different registers by playing different notes as you adjust the filter settings.) By adjusting the K-Track knob in SoundSchool, you can determine how closely the cutoff frequency follows the notes you're playing. With the K-Track knob set to 1, the cutoff frequency increases as you play higher notes and decreases when you play lower notes. With K-Track set at zero, the cutoff remains wherever you set it regardless of the note you play. Tracking is sometimes called "following," because with tracking enabled the cutoff frequency " follows " whatever note you've played.
Hands-on: Fine-tune a "brass" timbre with a filter
| |
Analog synthesis has traditionally involved starting with a basic waveform, then fine-tuning it with a filter. You can try this out by creating a textbook brass patch. Okay, so it won't sound much like real brass so much as a retro electronic sound. Most likely you'll use this technique to create distinctive , fat analog electronic sounds or apply similar filtering techniques to digital sounds. If you listen closely, there are some key similarities to real brass that give it that association.
Sawtooth waves are often said to be "brasslike" because they share a similar harmonic profile, so let's start there. Load preset 27Basic Saw, which sets Oscillator 1 to a sawtooth wave (a triangle wave with a Symm value of 1).
Try playing a note in the middle of the keyboard. It sounds vaguely brassy, but it's a little overly electronic and buzzy. It actually has too much energy in the upper part of the frequency spectrum. You can correct this with a slight filtering of that part of the spectrum. Try reducing the filter cutoff to 110. The result is not as buzzy, but now the patch sounds dead and muted. (Notice on the Scope that what you've done is round off the waveform, making it more like a sine wave.) Give it back some buzz by increasing the Filter Reson to 0.3.
Now you have a slightly richer sound, but it's still missing something. You can make this sound like a brass section by creating some artificial chorusing, slightly detuning a second oscillator to throw it out of phase with the first. Set Oscillator 2 to saw (that is, the same waveform as Oscillator 1) and increase its Mix value to 1. Then set detune to 0.15. Now you have a basic brass patch.
| | Still not satisfied? For a slightly different effect, adjust detune, cutoff, and resonance to taste. For fit and finish, tweak the Amp Envelope. (Try a release value of 30.) |
Hands-on: Create a filter sweep
| |
You can use filters to modulate sounds over time, thanks to filter envelopes. Let's start with a sound that's rich harmonically: two detuned sawtooth waves. Load preset 27Basic Saw. Set Oscillator 2 to a saw wave. Set its detune to 0.2 and its mix to 1. Now, let's give it a basic sweep from high to low. On the filter envelope, set attack, sustain, and release to 0, but set decay to 70. With just a decay value, the filter envelope becomes a straight line, dropping from top to bottom. Set the filter cutoff to 50 and the resonance to 80. Hold down a note and listen as the filter sweeps through the harmonic spectrum. (The resonance value makes that sweep more noticeable, and once the filter is at its lowest , you hear two phasing sine waves.)
| | More hands-on filters: Instrument designs often combine amplitude and filter envelopes for useful musical shapes. Try preset 47Bassh for an example. You can create a more pronounced effect by increasing filter envelope decay and filter envelope amount settings. |
You can use this technique for other filter envelope shapes as well. Instead of sweeping high to low, try sweeping low to high by setting the Filter > Env control to a negative value. Or, for a filter that opens up and closes again, try setting a larger value for the filter envelope attack parameter, creating a triangular or "mountain-shaped" envelope. Preset 80OpeningReso makes excellent use of this idea, by modulating the filter with a peak-shaped envelope with a high resonance value. Try different cutoff values to hear what the filter sweep sounds like at different frequencies.
|