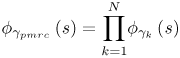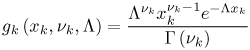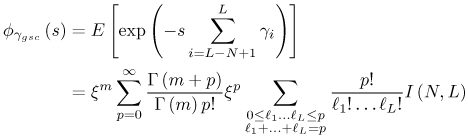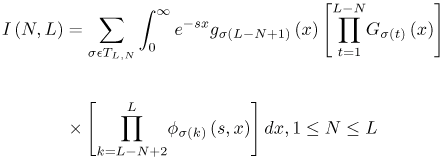Section 6.4. Case Study: Improving Range of UWB Using RAKE Receivers
|
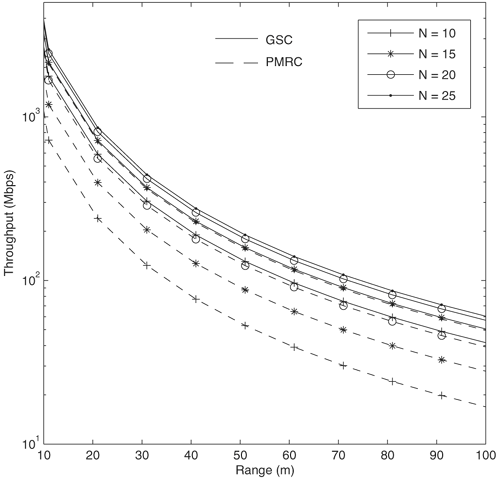
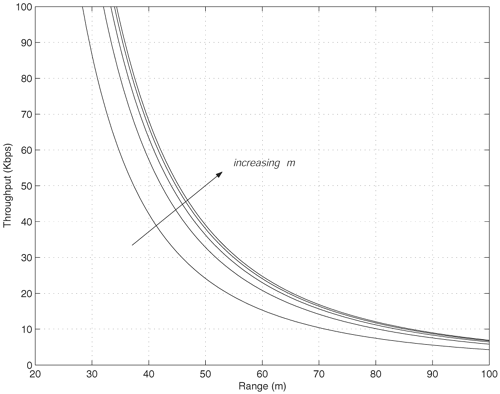
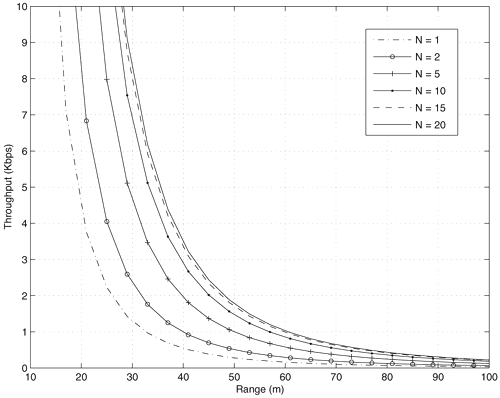
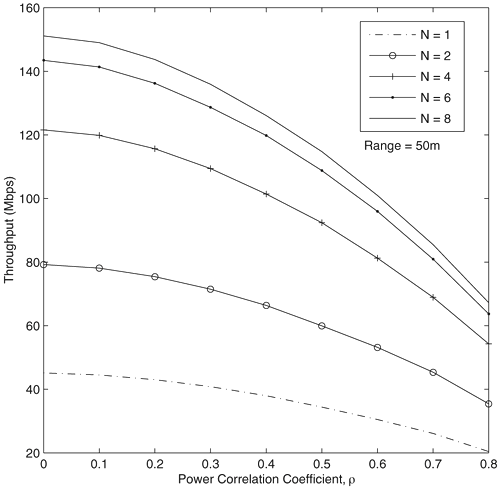
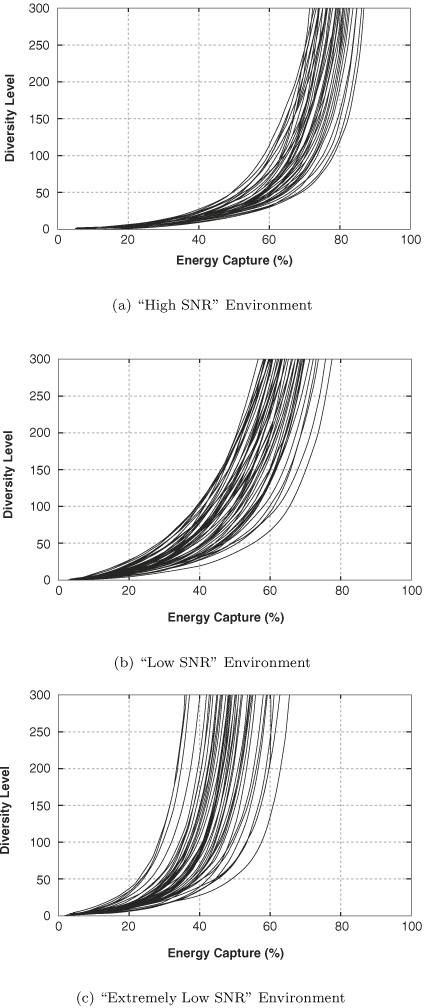
6.4. Case Study: Improving Range of UWB Using RAKE ReceiversThe fine time resolution of multipaths induced by the channel (typically in the order of 100300 picoseconds) can be exploited using a RAKE receiver to capture a significant amount of energy found in the multipath components, and to benefit from multipath diversity gain. Energy capture is important in UWB receiver design, and the number of detectable multipath components could be as large as a few hundred since UWB signals occupy bandwidth greater than 500 MHz or have a fractional bandwidth greater than 0.25. Clearly, classical RAKE receiver design solutions, such as MRC or selection combining, are no longer appropriate from the viewpoint of complexity and performance trade-offs. The realistic trade-off between the energy capture and RAKE diversity level in dense multipath for UWB systems have been quasi-analytically/experimentally presented in [54]. The experimental results are shown in Figure 6.56. Figure 6.56. The Required Diversity Level, L, in a UWB RAKE Receiver as a Function of Percentage Energy Capture for Each of the 49 Received Waveforms in an Office Representing High, Low, and Extremely Low SNR Environments.SOURCE: M. Z. Win and R. A. Scholtz, "On the Energy Capture of Ultrawide Bandwidth Signals in Dense Multipath Environments," IEEE Communications Letters [54]. © IEEE, 1998. Used by permission. In this section, we examine the throughput range trade-off of UWB communication systems that employ two variants of RAKE receiver structures in multipath fading channels: (a) the generalized selection combining receiver (hereafter, referred to as GSC(N,L)) that combines a subset of N resolvable multipaths with the highest instantaneous SNR out of L resolvable multipaths in a similar fashion to MRC; and (b) MRC with finite tap statistics or partial MRC (hereafter, referred to as PMRC(N, L)) that combines N multipaths with the largest mean SNR. Figures 6.57 a and b show the schematic for these receiver structures respectively. Figure 6.57. Hybrid RAKE Receiver Structures.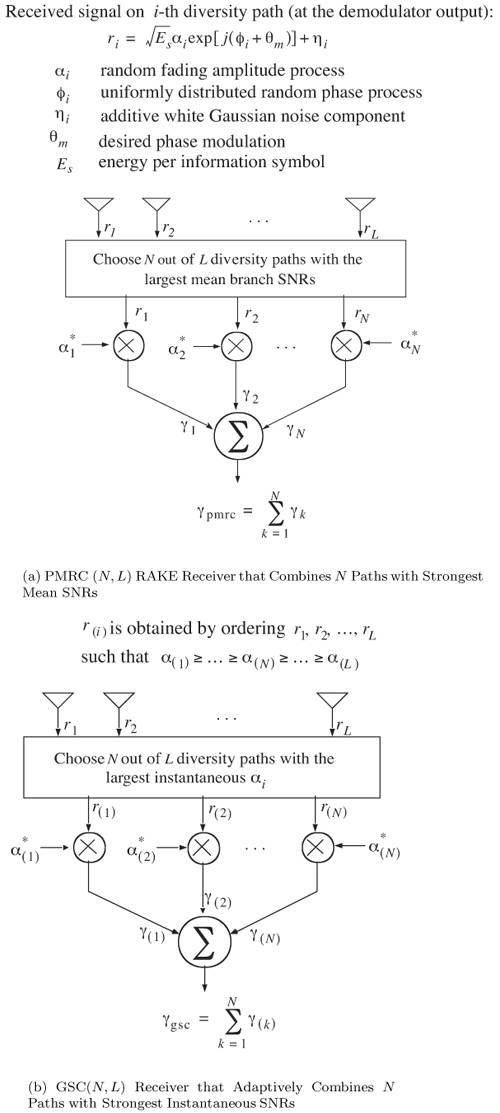 Clearly, PMRC is simpler to implement compared to GSC owing to the simpler selection circuitry complexity (i.e., the need to select N paths with the largest instantaneous SNR in the latter). The motivation for performance analyses of the previous two suboptimal receiver structures is based on the propagation measurements of UWB signals at Virginia Tech [84] that indicates the significant amount of energy that is found in a few dominant multipaths, particularly for the LOS case. For example, approximately 80% of the receiver energy could be captured by combining only 12 dominant multipaths. However, for the NLOS situation, approximately 75% of energy is captured by combining 30 dominant multipaths. In [85], the performance comparisons between GSC and PMRC over Nakagami-m channels have been studied in the context of spread spectrum communications but with only a few multipaths. In [86], the authors resorted to a semianalytical approach for analyzing the average bit error rate (ABER) performance of GSC(N,L) and PMRC(N, L) at a fixed distance. In contrast, we now examine the throughput range performance of UWB for two different reduced-complexity receiver structures over generalized fading channels using exact and approximate ABER expressions (rather than the semianalytical approach discussed in [86]). We assume a UWB system operating in a Rayleigh or a Nakagami-m fading environment and present analytical expressions for the throughput as a function of the distance for the GSC(N,L) and PMRC(N, L) receiver structures. The bit error probability for an M-ary PAM system is given by Equation 6.153 where M is the alphabet size and c = log 2M denotes the number of information bits carried by each symbol. The instantaneous SNR/bit is g = (Eb/N0)a2 where Eb denotes received energy per bit, No is noise spectral density, and a denotes random fading amplitude. The average SNR per bit at the receiver input can be computed as Equation 6.154 where n denotes the path loss exponent, Ls is the system loss factor not related to propagation (Ls The noise spectral density is N0 = kTF where k is Boltzman's constant, T is system temperature, and F denotes the noise figure. From (6.155) it is apparent that for fixed transmit power, throughput is a function of dn. The average SNR per bit required to evaluate (6.155) for a given bit error rate performance can be found by averaging (6.153) over the probability distribution function (PDF) of GSC(N,L) or PMRC(N, L). 6.4.1. GSC(N,L) with Independent but Nonidentically Distributed Fading StatisticsExact Analysis of GSC(N,L)Using the moment-generating function (MGF) approach, we obtain Equation 6.156 where fg(.) denotes the MGF of the GSC(N,L) output SNR, ggsc. The MGF expressions for the GSC(N,L) output SNR in a myriad of fading environments has been developed in [87,88], namely Equation 6.157 where Equation 6.158 while SL is the set of all permutations of integers [1, 2, . . ., L], and s Approximate Analysis of GSC(N,L)The throughput range performance of UWB systems employing GSC receivers can be analyzed using the previous equations. However, as the number of resolvable multipath components increases, it becomes computationally intensive to evaluate (6.156) along with (6.157), and turns out to be impractical for the case L 6.4.2. PMRC(N, L) with Independent but Nonidentically Distributed Fading StatisticsIt is not difficult to show that the MGF of PRMC (N, L) output SNR may be computed as Equation 6.159 where fgk (.) denotes the MGF of the kth strongest (mean SNR) diversity path. Hence, the ABER performance of PMRC may be analyzed using (6.156) and (6.159), and the maximum achievable throughput can be easily computed as before. 6.4.3. GSC(N,L) with Equally Correlated Nakagami-m Fading StatisticsIn order to gain significantly from the use of diversity, there must be a sufficient level of statistical independence in the fading of the received signal in different diversity paths. In UWB communication systems with closely received resolvable multipath components, an assumption of independent multipath signals may lead to grossly overestimated diversity gains. As such, a study of the effect of multipath correlation on the performance of a UWB communication system is important. In this section, we will provide analytical expressions to evaluate the ABER performance of the GSC(N,L) receiver structure in equally correlated Nakagami-m multipath fading channels. Let g(1), . . ., g(L) denote the instantaneous SNRs in ascending order, namely g(1)< g(2)< . . . < g(L). Using the property of exchangeability, the joint PDF of the ordered set of instantaneous SNR can be expressed as Equation 6.160 The joint PDF of the ordered instantaneous SNRs in a Nakagami-m channel can be obtained using Equation 21 of [90] and (6.160) as Equation 6.161 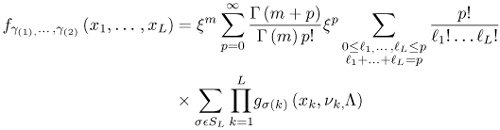 where m Equation 6.162 It is apparent from (6.161) that we can compute the MGF fggsc (.) in a similar fashion to GSC with i.n.d fading statistics [88], namely Equation 6.163 where I(., .) is defined as Equation 6.164 where Equation 6.165 Equation 6.166 where The results discussed in this section use the assumptions in Table 6.2. Figure 6.58 compares the accuracy of "approximate ABER analysis" against the "exact ABER analysis" using (6.157) for a GSC(N, 12) receiver with i.n.d diversity branches in Rayleigh fading channels. It is evident that the throughput curves corresponding to approximate ABER match closely with the exact throughput curves. Readers are referred to [92] for further details.
Figure 6.58. Throughput Performance of a GSC (N, 12) Receiver that Adaptively Combines N | ||||||||||||||||||
|
EAN: 2147483647
Pages: 110
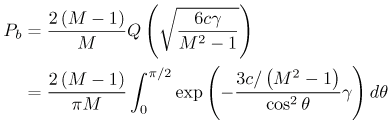
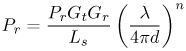
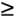 1),
1), 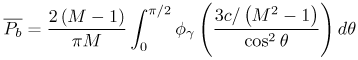
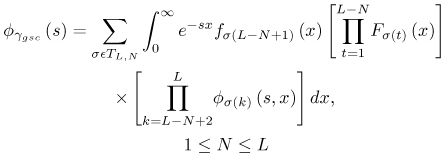
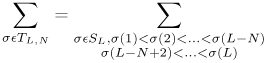
 (
(