UNIFORM DISTRIBUTION

If we consider each die as an independent random variable, then a uniform probability distribution will be the result and will take the shape shown in Figure 16.8.

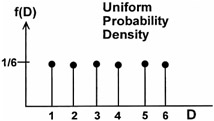
Figure 16.8: Uniform probability density for a die.

With mean value of single die:

Variance about mean:

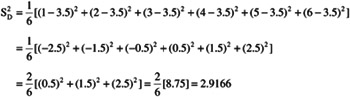
Standard deviation: S _D = 1.708

The uniform distribution may be represented in general mathematical notation along with the constraints as:

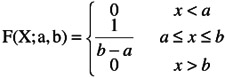
and the shape looks like Figure 16.9.

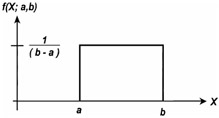
Figure 16.9: A generic uniform distribution.

When checking for the probability density function of a uniform distribution one should check the following two properties:

1. Positive: f {X; a,b) ‰ O

2. Unit area:

   

Note  

Height of p.d.f. is equal to the reciprocal of the base. Figure 16.10 shows the comparison of the uniform distribution and the cumulative density function.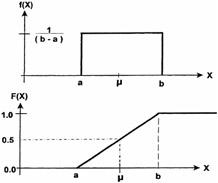

Figure 16.10: A comparison of the uniform distribution and its C.D.F.

The mathematical notation of the c.d.f. with the constraints is:

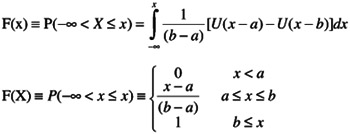
Mean:

Variance:

The reader will notice that in the uniform distribution, (1) median = mean, and (2) there is no mode.

Sound level in a room is found to be uniformly distributed between 80 and 95 dBA. Occupational Safety and Health Administration (OSHA) regulations set a maximum safe level of 90 dBA for an 8- hour workday . See Figure 16.11.

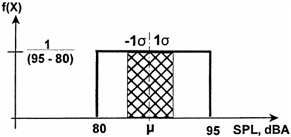
Figure 16.11: The sound level in a room.

Find:

1. The probability density function, f(x) for this noise

2. The probability of exceeding the 90 dBA standard

3. The mean and standard deviation

4. The level range within ± 1 ƒ of the mean

5. The probability of being in this ± 1 ƒ about the mean

Solutions:

1. f(X) = 1/15; 80 ‰ x ‰ 95

2. Probability of exceeding 90 dBA:

   P(> 90) = 1 - P(x ‰ 90) = 1 - F(x = 90)

   

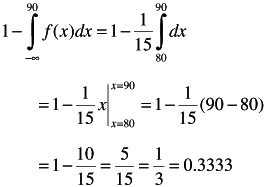
3. Mean:

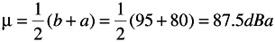
   Standard Deviation:

   

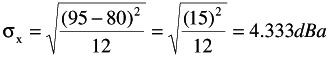
4. One-sigma range about mean: ( ¼ - 1 ƒ ) ‰ x ‰ ( ¼ + 1 ƒ )

   83.2 ‰ x ‰ 91.8

5. Probability of SPL being in this one-sigma range