6.5 Real options
6.5 Real options
The term real option is used to mean three different things [12]: A calculation method based on or derived from the Black-Scholes[10] equation used in derivatives trading [13], as a means of deriving risk assessments from market-traded securities and commodities [14], and a tool for framing decisions [15].
The original concept of real option valuation is derived from its financial counterpart, where an option represents a right, but not an obligation, to buy or sell something at a predefined price on or before a certain date. In the financial markets, options are contracts to buy or sell some asset, such as a stock, some commodity, or foreign exchange. In real options, the assets are cash flows instead of financial instruments.
For example, a company might have a contract specifying the right to buy 1 million euros at the price of US $1 per euro up to 1 year from now. The company might pay $50,000 now for such a contract. If at any time the price of the euro rises above $1.05 the company could exercise the option and pocket the difference.
The value of the option arises from the risk asymmetry it purports. Notice that in the example above, the losses the company might incur are limited to $50,000, while the gains are theoretically unlimited. Whatever the exchange rate between the euro and the dollar, the company will still pay $1 for each euro, but only if it benefits from it.
Conceptually, a project with a development phase and a commercialization phase could be considered as an option because the cost of the development gives us the opportunity, but not the obligation, to commercialize the results. The connection between project valuation and option pricing is made (see Figure 6.16) by mapping the project characteristics onto option parameters and then using the Black-Scholes formula for calculating the project value.
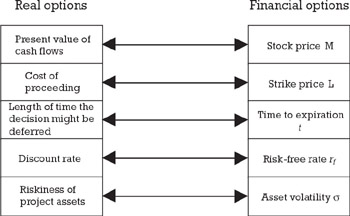
Figure 6.16: Mapping between real and financial options parameters. (After: [16].)

where
V = value of call option on a risk asset = project valuation
L = strike price = cost of proceeding
t = time to expiration = time at which the decision to proceed must be made
rf = risk-free interest rate = discount rate
M = current price of the asset = present value of project-generated revenues
σ = standard deviation of asset's rate of return = volatility of the generated revenues
FN()= cumulative probability of a normally distributed variable e = 2.71
Although the computation of the Black-Scholes formula is straightforward, one must be aware of the assumptions behind it before valuating a project. First, the risk that the formula captures refers to the risk in the returns and not to internal project risks such as staff turnover, lack of funding, or technical difficulties; this still needs to be handled separately [17] with conventional decision trees. The second warning concerns the variability of the generated revenues. In financial options, the variability is obtained from a portfolio of similar assets that are used as a proxy, but in the case of new-product development, such a proxy does not exist or data on it is not readily available. Using the formula outside its domain of application can lead to the making of poor decisions.
As a tool for framing decisions, real options have been used to advocate for building more flexibility into systems [15] and as a justification for not building into systems features that are not needed today [13].
[10]Fisher Black, Myron Scholes, and Robert Merton received the 1977 Nobel Prize in economics for their work on the pricing of derivatives. Their theory and the various forms of their formula are widely used in the trading industry
EAN: 2147483647
Pages: 81