Tool 19: Box Plot

AKA

Box and Whisker Plot

Classification

Analyzing/Trending (AT)

Tool description

The box plot illustrates a distribution of data showing location and spread of values, skewness, and possible outliers (extremely high or low scores). Although a box plot is less detailed than a histogram, it is quite useful in that it will display extreme variations in the data plotted.

Typical application

• To identify outliers in a data set.

• To compare groups of data for significant differences in patterns.

• To check for improvement in data after a process change has been made.

Problem-solving phase

	Select and define problem or opportunity
→	Identify and analyze causes or potential change
	Develop and plan possible solutions or change
	Implement and evaluate solution or change
→	Measure and report solution or change results
	Recognize and reward team efforts

Typically used by

1	Research/statistics
	Creativity/innovation
3	Engineering
	Project management
2	Manufacturing
	Marketing/sales
	Administration/documentation
	Servicing/support
	Customer/quality metrics
	Change management

links to other tools

before

• Data collection strategy

• Stem and Leaf Display

• Observation

• Dot Diagram

• Sampling Methods

after

• Variance Analysis

• Problem analysis

• Process analysis

• Potential problem analysis (PPA)

• What-If Analysis

Notes and key points

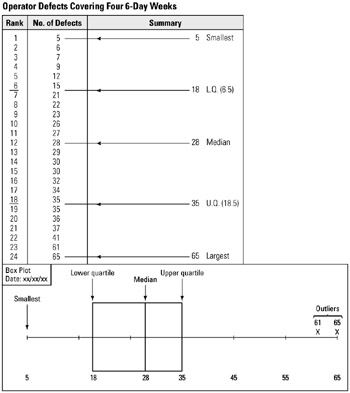
Use the follwing equation to calculate ranks for quartiles:

• For this example:

• – Median rank=

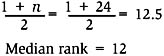
• – Rank of lower quartile (L.Q.)=

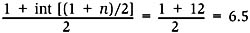
• – Rank of upper quartile (U.Q.)= (n + 1) − L.Q. = (24 + 1) − 6.5 = 18.5

• To identify outliers use the following:

• – Check for larger data values:

  

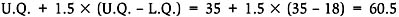
• – Therefore defect scores 61 and 65 are outliers at the high end of the distribution.

• – Check for smaller data values:

  

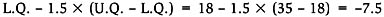
• – The lowest data value = 5; therefore there is no outlier at the low end of the distribution.

Step-by-step procedure

• STEP 1 Data is collected and identified by recording the collection period, data source, and exact description of what is supposed to be measured. See example Operator Defects Covering Four 6-Day Weeks.

• STEP 2 A table of rank-ordered data is constructed and median, lower and upper quartiles calculated. See notes and key points for the calculations used in this example.

• STEP 3 A box plot is constructed as shown. Ensure that outliers are identified and marked.

• STEP 4 Check all data values, box plot dimensions and outlier values, date the plot, and make final notes on the pattern or variation of the data.

Example of tool application