| Critical path tasks that overrun their most-of-the-time estimates cause project time overruns. Tasks that are not on the critical path can overrun their most-of-the-time estimates without affecting a project's time if the overrun is less than the slack between this noncritical path task and its critical path successor. However, if a noncritical path task overruns by an amount greater than its slack, it can cause a project time overrun (that is, if a task D is greater than the slack between it and the following critical path task, it can cause trouble). So far we have only considered project time overruns caused by the time overruns of critical path tasks. Noncritical path tasks can cause just as much over-run as critical path tasks if their D exceeds their slack. Noncritical path tasks provide a contribution to the risk factor if the difference between their D and their slack (D - slack), multiplied by their overrun likelihood, is greater than the D times the likelihood of the parallel task on the critical path. In this case, the contribution of the noncritical path tasks replaces the contribution of its parallel critical path task in the risk factor contribution table. Refer back to Figure 7-4 and see Figure 9-2, which is an enlargement of a small portion of Figure 7-4. Plumbing and furnace and ducts are parallel tasks. Plumbing is on the critical path. The furnace and ducts is 0.25 days shorter than plumbing. It has a 0.25 slack. The 2.5 days most-of-the-time task time of plumbing directly contributes to the critical path time. If the worst-case task time for plumbing is three days, D is 3 - 2.5, which is 0.5 days. If the overrun likelihood for plumbing is 10 percent, plumbing's contribution to the risk factor is 0.5 x 0.1,which is 0.05 days very small. If the worst case duration for the furnace and ducts is 3.5 days, the D for furnace and ducts is 3.5 - 2.25,or 1.25 days. This combines with an overrun likelihood for furnace and ducts of 20 percent. For furnace and ducts, most of the time plus slack is 2.25 + 0.25, or 2.5 days. (Most of the time + slack for a noncritical path is always equal to the critical path tasks most-of-the-time duration.) Delta (D) - slack is 1.25 - 0.25, or one day. The contribution to the risk factor for furnace and ducts is D - slack x likelihood, 1.0 x 0.2, or 0.2 days. Because 0.2 days is much bigger than 0.05 days, the 0.2 days contribution is used on the risk factor contribution table instead of 0.05.
Figure 9-2. 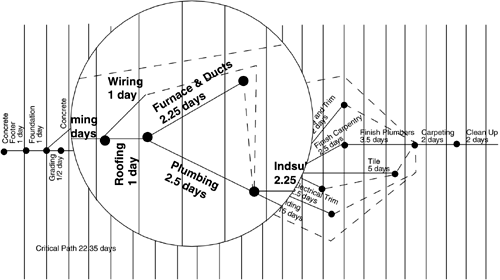
Although these numbers are small, the difference between them is considerable. This same relationship could show up if the tasks were 22.5 days and 25 days, respectively. In this case, the contribution difference would be between half a day and two days. The difference this makes in the risk factor is considerable. Yet, we are looking at only relatively small Ds for both tasks. Some very large, worst-case overruns and worst-case likelihoods can be hidden from analysis if we do risk factor calculations only for critical path tasks. Because of this possibility, every task leader must conduct a contribution analysis for his or her task and produce a risk analysis worksheet (refer to Figure 9-1). The task leader also must note on the worksheet the slack in days (or weeks) between his or her task and the subsequent critical path task. The critical path arrow diagram that the project manager has prepared and distributed is very useful for task leaders at this time. It clearly shows the slack. The project manager now must ask the task leader of the critical path task and the task leader of the parallel task that has slack to compare their contribution calculations if the parallel task has a D that is greater than its slack. The contribution of the critical path task to the risk factor is D x Y; The contribution of the parallel noncritical path task to the risk factor is (D - slack) x Y; whichever number is larger is used on the risk factor contribution table. This still does not take care of all of the overrun threats. Sometimes a critical path task is paralleled by a sequence of tasks that is not on the critical path and ends in slack. A simple example of a two-task parallel stream is shown in Figure 9-3. In this figure, Task A is on the critical path and has a duration of 31 days. It is followed by Task D on the critical path. There is no slack found between A and D. Any task time overrun by Task A will need to be absorbed by the risk factor at the end of the critical path string. Task A has a D of three days and a likelihood of overrun of 10 percent. It has made a contribution of 3 x 0.1 = .3 day to the risk factor.
Figure 9-3. 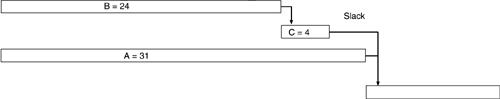
Task B is a parallel task not on the critical path that is the predecessor of Task C. This creates a string of tasks that must end before Task D can begin. Task D is dependent on completion of both Tasks A and C. There are three days of slack that apply to the B-C stream before Task D starts. Task B has a duration of 24 days. Task B's task leader reports that the task has a D of seven days and a likelihood of 30 percent. Task C has a duration of four days, a D of one day, and a likelihood of 40 percent. The worst case impact of Task A on project time could be three days (DA).Tasks B and C have a combined D (DB + DC) of 7+1 = 8 days). If both tasks overran at their maximum, they would not make an eight-day impact on the project finish date, and they would make only an 8 days - 3 days slack, or five day-later impact on the project finish date. These five days would have to be absorbed by the downstream risk factor. However, the team has not calculated a risk factor contribution that takes this into account. Therefore, the project manager guides the task leaders of Task B and C in calculating a risk factor contribution to substitute for Task A's contribution. Because Task B has the much larger D for the string, use Task B's likelihood of overrun (30 percent), to calculate the new contribution. The contribution is 5 x 0.3 = 1.5 days. Because a string of critical path tasks is paralleled by a string of noncritical path tasks that end in slack, the calculations become more complicated, but the principles remain the same. This analysis is more difficult to describe than it is to perform. It is some-thing that a project manager must understand, however. The real danger is that a task like Task B in the first example is buried in a string of tasks that parallel the critical path and end with what appears to be generous slack. This can lead to ignoring Task B as a risk danger. However, Task B may have a large D and a large likelihood, and be capable of greatly overrunning the available slack. Therefore, every task should be evaluated in terms of D x Y during risk analysis. Any task leader who sees that his or her task has a potential for a larger risk contribution than the critical path task must talk this problem over with the project manager. Together, they can conduct an examination of the parallel risks and adjust the risk factor as necessary. One terrible surprise that can impact a project's execution is to discover that an unnoticed task not on the critical path produces a project delay whose possibility has not been accounted for. The key to completing good risk analysis is to look for a D (or a string of Ds) for noncritical path tasks leading up to slack that is much greater than the slack. In risk analysis, the project manager and team always must keep their wits and judgment about them. It may take two or three closely spaced meetings to cover all risk factor considerations. When the review is finished, the Gantt chart should be revised and distributed to team members, with the risk factors appropriately inserted. At this point, it is time to print out all the task notes for distribution to the team members. |