Section 14.3. Large-Scale Optical Switches

14.3. Large-Scale Optical Switches

Large-scale optical switches can be achieved either by implementing large-scale star couplers or by cascading 2 x 2 or even 1 x 2 multiplexers. However, the implementation of star couplers larger than 2 x 2 is expensive, owing to difficulties in the manufacturing process. Cascading small switches is a practical method to expand a switch. This method of switch expansion can make a desired- size switch quickly and at lower expense. However, some factors affect the overall performance and cost of an integrated switch, as follows :

• Path loss. Large-scale switches have different combinations of switch elements; therefore, a signal may experience different amounts of losses on different paths. Hence, the number of switch elements cascaded on a certain path might affect the overall performance of the switch.

• Number of crossovers. Large optical switches can be manufactured on a single substrate by integrating multiple switch elements. In an integrated optical system, a connection between two switch elements is achieved by one layer of a waveguide . When paths of two waveguides cross each other, the level of cross talk increases on both paths. As a result, the number of crossovers in an optical switch must be minimized.

• Blocking. As discussed in Section 13.1, a switch is wide-sense nonblocking if any input port can be connected to any unused output port without requiring a path to be rerouted; is rearrangably nonblocking if, for connecting any input port to an unused output port, a rearrangement of other paths is required.

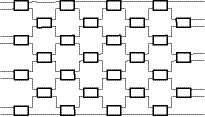
There are several proposed topologies for large-scale switching networks. Two of the more practical ones are crossbar and the Spanke-Bene network architectures.

14.3.1. Crossbar Switching Network

The architecture of an optical crossbar is not quite the same as the one in Chapter 13, as indicated in Figure 14.4. An optical crossbar is wide-sense nonblocking. The fundamental difference in the architecture of the optical crossbar and traditional crossbars is the existence of different and variable-length paths from any input to any output. This feature was created in the structure of the optical crossbar to minimize the crossover of interconnections. An optical crossbar is made up by 2 x 2 switches. Denoting the number of switches a signal faces to reach an output port from an input port by , the bounds for can be investigated from the figure as

Equation 14.1

Figure 14.4. A 4 x 4 wide-sense nonblocking optical crossbar without waveguide crossovers, using basic 2 x 2 switch elements

where n is the number of inputs or outputs of the crossbar. In an n x n crossbar, the number of required 2 x 2 switches is n ² . The 16 2 x 2 switches in the Figure 14.4 crossbar are structured in an appropriate way. The main issue with the crossbar structure is its cost, which is a function of n ² . But a great advantage of such an architecture is that the switch can be manufactured without any crossovers.

14.3.2. Spanke-Bene Switching Network

Figure 14.5 shows a rearrangably nonblocking switch called a Spanke-Bene switching network . This network is designed with identical 2 x 2 switch elements, and there are no crossover paths. This switching network is also known as n -stage planar architecture. Denoting the number of switches a signal faces to reach an output port from an input port by , we can derive the bounds for by

Equation 14.2

Figure 14.5. An 8 x 8 Spanke-Bene switching network with rearrangeably nonblocking structure without requiring any interconnection crossover

This inequity can be verified from Figure 14.5, which shows multiple paths between each input/output pair. Thus, the longest possible path in the network is n , and the shortest possible path is n/ 2. This arrangement is made to achieve a moderately low blocking. Thus, there are n stages ( columns ) and switch elements in an n x n switching network.