Performing Statistical Tests
Problem
You're engaged in hypothesis testing and you'd like to learn about Excel's support for conducting statistical tests .
Solution
Excel provides support for conducting standard z-tests, t-Tests, and F-tests. Read the following discussion to learn more.
Discussion
The built-in function ZTEST computes the one-tailed z-test using the normal distribution. The syntax for this function is =ZTEST(cell ref, m, s) where cell ref is a cell reference containing an input dataset, m is the value being tested, and s is the population standard deviation. If s is not known, it can be omitted, in which case the sample's standard deviation will be computed and used in the z-test calculation.
You can also compute two-tailed z-tests as follows: =2 * MIN(ZTEST(cell ref, m, s), 1 - ZTEST(cell ref, m, s)).
The function TTEST computes the one- or two-tailed t-test using Student's t-distribution. Further, TTEST allows you the option of performing paired, two sample with equal variance, or two sample with unequal variance tests. The syntax for TTEST is =TTEST(cell ref 1, cell ref 2, tails, type), where cell ref 1 and cell ref 2 are cell references to the two datasets under consideration and tails is the number of tails to consider for the test (1 or 2). type is a value of 1, 2, or 3, indicating the test type: paired, two sample equal variance, or two sample unequal variance, respectively.
FTEST computes the two-tailed F-test for two datasets. The syntax for FTEST is =FTEST(cell ref 1, cell ref 2), where cell ref 1 and cell ref 2 are cell references to the two datasets under consideration.
All three of these test functions have corresponding inverse functions (namely, NORMSINV, TINV, and FINV) that are useful when computing critical values for comparison. NORMSINV and TINV were discussed in Recipe 5.3. Recipe 8.7 makes use of FTEST and FINV while performing an F-test in the context of regression analysis.
The Analysis ToolPak also provides several tools for conducting statistical tests. These tools include:
- F-Test Two-Sample for Variances
- t-Test Paired Two-Sample for Means
- t-Test Two-Sample Assuming Equal Variances
- t-Test Two-Sample Assuming Unequal Variances
- z-Test Two-Sample for Means
To access these tools, select Tools  Data Analysis from the main menu bar to open the Data Analysis dialog box. This is the same Data Analysis dialog box shown earlier in Figure 5-13. Youll find each of the statistical test tools listed in this dialog box. They all work pretty much the same way: select a tool to open the tool's dialog box, select input cell ranges, specify your hypothesis, and select your output options.
Data Analysis from the main menu bar to open the Data Analysis dialog box. This is the same Data Analysis dialog box shown earlier in Figure 5-13. Youll find each of the statistical test tools listed in this dialog box. They all work pretty much the same way: select a tool to open the tool's dialog box, select input cell ranges, specify your hypothesis, and select your output options.
I'll show an example using the t-Test Two-Sample Assuming Unequal Variances tool. Figure 5-16 shows a portion of a spreadsheet containing measured weights of North American bullfrogs sampled from two southern states.
Each state's sample contains 60 data points. An example hypothesis to test here (a null hypothesis) is that there's no difference between the mean weights of bullfrogs from Louisiana and Alabama.
To conduct this test, select t-Test: Two-Sample Assuming Unequal Variances from the Data Analysis dialog box discussed earlier to open the t-Test dialog box shown in Figure 5-17.
Figure 5-16. Bullfrog weight data
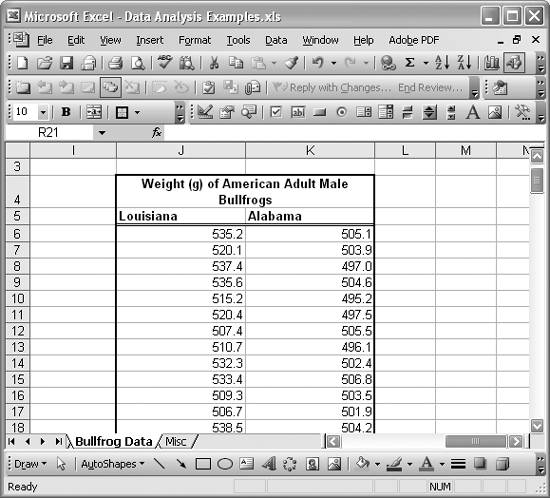
Figure 5-17. t-Test dialog box
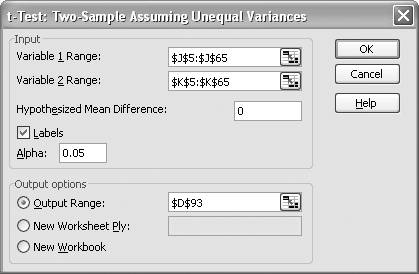
In the Variable 1 Range and Variable 2 Range fields, type (or select from the spreadsheet) the cell ranges containing the dataset corresponding to Louisiana and Alabama, respectively. In this example, I included the column labels in these input ranges, so I checked the Labels checkbox.
|
The hypothesis is that there is no difference between the means; therefore, enter a value of 0 in the Hypothesized Mean Difference field. In this example, I left the Alpha field (confidence field) at the default 5%; but you can change that as well. Finally, choose your output options. In this case, I chose to have the output on the same spreadsheet, starting at cell D93. Press OK; the results are shown in Figure 5-18.
Figure 5-18. t-Test results
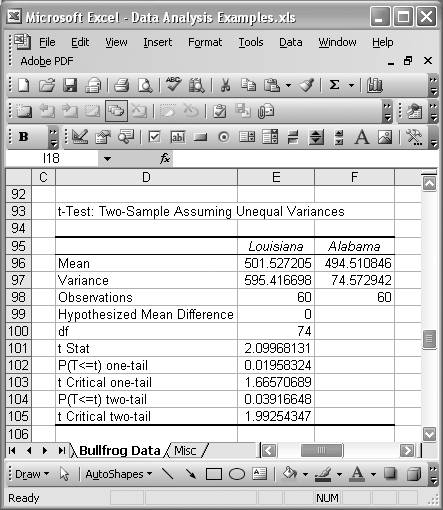
These results include computed means and variances for the two input datasets, along with other information such as the number of observations, the hypothesized mean difference, and the degrees of freedom. The t-statistic is also reported, along with both the one-tailed and two-tailed probabilities and critical values. In this case, the t-statistic is 2.1, which is greater than the two-tailed t-critical value, thus indicating there is a statistically significant difference in means between the two datasets. You can also see that the P-value is less than the significance level (Alpha value of 5% in this case), which also indicates rejection of the null hypothesis.
The other statistical test tools available in the Analysis ToolPak generate results that look very much like the ones shown in Figure 5-18.
See Also
To learn more about the Analysis ToolPak statistical tests, read the Excel help topic entitled "About statistical analysis tools." Refer to any standard text that covers statistical tests, or tests of significance, to learn more about these and other statistical tests.
Using Excel
- Introduction
- Navigating the Interface
- Entering Data
- Setting Cell Data Types
- Selecting More Than a Single Cell
- Entering Formulas
- Exploring the R1C1 Cell Reference Style
- Referring to More Than a Single Cell
- Understanding Operator Precedence
- Using Exponents in Formulas
- Exploring Functions
- Formatting Your Spreadsheets
- Defining Custom Format Styles
- Leveraging Copy, Cut, Paste, and Paste Special
- Using Cell Names (Like Programming Variables)
- Validating Data
- Taking Advantage of Macros
- Adding Comments and Equation Notes
- Getting Help
Getting Acquainted with Visual Basic for Applications
- Introduction
- Navigating the VBA Editor
- Writing Functions and Subroutines
- Working with Data Types
- Defining Variables
- Defining Constants
- Using Arrays
- Commenting Code
- Spanning Long Statements over Multiple Lines
- Using Conditional Statements
- Using Loops
- Debugging VBA Code
- Exploring VBAs Built-in Functions
- Exploring Excel Objects
- Creating Your Own Objects in VBA
- VBA Help
Collecting and Cleaning Up Data
- Introduction
- Importing Data from Text Files
- Importing Data from Delimited Text Files
- Importing Data Using Drag-and-Drop
- Importing Data from Access Databases
- Importing Data from Web Pages
- Parsing Data
- Removing Weird Characters from Imported Text
- Converting Units
- Sorting Data
- Filtering Data
- Looking Up Values in Tables
- Retrieving Data from XML Files
Charting
- Introduction
- Creating Simple Charts
- Exploring Chart Styles
- Formatting Charts
- Customizing Chart Axes
- Setting Log or Semilog Scales
- Using Multiple Axes
- Changing the Type of an Existing Chart
- Combining Chart Types
- Building 3D Surface Plots
- Preparing Contour Plots
- Annotating Charts
- Saving Custom Chart Types
- Copying Charts to Word
- Recipe 4-14. Displaying Error Bars
Statistical Analysis
- Introduction
- Computing Summary Statistics
- Plotting Frequency Distributions
- Calculating Confidence Intervals
- Correlating Data
- Ranking and Percentiles
- Performing Statistical Tests
- Conducting ANOVA
- Generating Random Numbers
- Sampling Data
Time Series Analysis
- Introduction
- Plotting Time Series Data
- Adding Trendlines
- Computing Moving Averages
- Smoothing Data Using Weighted Averages
- Centering Data
- Detrending a Time Series
- Estimating Seasonal Indices
- Deseasonalization of a Time Series
- Forecasting
- Applying Discrete Fourier Transforms
Mathematical Functions
- Introduction
- Using Summation Functions
- Delving into Division
- Mastering Multiplication
- Exploring Exponential and Logarithmic Functions
- Using Trigonometry Functions
- Seeing Signs
- Getting to the Root of Things
- Rounding and Truncating Numbers
- Converting Between Number Systems
- Manipulating Matrices
- Building Support for Vectors
- Using Spreadsheet Functions in VBA Code
- Dealing with Complex Numbers
Curve Fitting and Regression
- Introduction
- Performing Linear Curve Fitting Using Excel Charts
- Constructing Your Own Linear Fit Using Spreadsheet Functions
- Using a Single Spreadsheet Function for Linear Curve Fitting
- Performing Multiple Linear Regression
- Generating Nonlinear Curve Fits Using Excel Charts
- Fitting Nonlinear Curves Using Solver
- Assessing Goodness of Fit
- Computing Confidence Intervals
Solving Equations
- Introduction
- Finding Roots Graphically
- Solving Nonlinear Equations Iteratively
- Automating Tedious Problems with VBA
- Solving Linear Systems
- Tackling Nonlinear Systems of Equations
- Using Classical Methods for Solving Equations
Numerical Integration and Differentiation
- Introduction
- Integrating a Definite Integral
- Implementing the Trapezoidal Rule in VBA
- Computing the Center of an Area Using Numerical Integration
- Calculating the Second Moment of an Area
- Dealing with Double Integrals
- Numerical Differentiation
Solving Ordinary Differential Equations
- Introduction
- Solving First-Order Initial Value Problems
- Applying the Runge-Kutta Method to Second-Order Initial Value Problems
- Tackling Coupled Equations
- Shooting Boundary Value Problems
Solving Partial Differential Equations
- Introduction
- Leveraging Excel to Directly Solve Finite Difference Equations
- Recruiting Solver to Iteratively Solve Finite Difference Equations
- Solving Initial Value Problems
- Using Excel to Help Solve Problems Formulated Using the Finite Element Method
Performing Optimization Analyses in Excel
- Introduction
- Using Excel for Traditional Linear Programming
- Exploring Resource Allocation Optimization Problems
- Getting More Realistic Results with Integer Constraints
- Tackling Troublesome Problems
- Optimizing Engineering Design Problems
- Understanding Solver Reports
- Programming a Genetic Algorithm for Optimization
Introduction to Financial Calculations
- Introduction
- Computing Present Value
- Calculating Future Value
- Figuring Out Required Rate of Return
- Doubling Your Money
- Determining Monthly Payments
- Considering Cash Flow Alternatives
- Achieving a Certain Future Value
- Assessing Net Present Worth
- Estimating Rate of Return
- Solving Inverse Problems
- Figuring a Break-Even Point
Index
EAN: 2147483647
Pages: 206

