11.2 Specifications of the various processes
11.2 Specifications of the various processes
We present a family of stochastic processes describing the evolution of forward rates and hence uniquely determining the short- term interest rate and bond price processes. A series of conditions is presented ensuring the processes are bounded and well behaved.
11.2.1 Forward and short-term interest rate processes.
Technical conditions are applied to the processes defining the short and forward interest rates as well as the money market account.
| |
A family of forward rate processes. Define a family of forward rate processes f ( t, T ), for fixed T ˆˆ [0, ]:
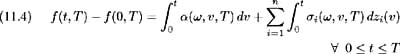
where [2] :
-
{ f (0, T ): T ˆˆ [0, ] } is a fixed, non-random initial forward rate curve, measurable as a mapping f (0, ·):([0, ],
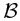 [0, ]) ’ ( R ,
[0, ]) ’ ( R , 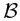 ) where
) where 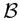 [0, ] is a Borel ƒ -algebra restricted to [0, ].
[0, ] is a Borel ƒ -algebra restricted to [0, ]. -
± : {( t, s ):0 t s T } ’ R is a family of drift functions jointly measurable from F
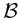 {( t, s ):0 t s T } ’
{( t, s ):0 t s T } ’ 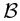 , adapted and having
, adapted and having 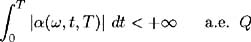
-
ƒ i : {( t, s ): 0 t s T } ’ R are volatilities, jointly measurable from
F
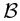 {( t, s ): 0 t s T } ’
{( t, s ): 0 t s T } ’ 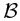 , adapted and with
, adapted and with 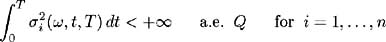
| |
Starting from the initial fixed forward rate curve { f (0, T ): T ˆˆ [0, ]}, the n independent Brownian motions determine the stochastic evolution of the whole forward curve through time. The sensitivity of the change in a given maturity forward rate to each Brownian motion, is specified by the volatility coefficients. The only restrictions imposed on the forward rate process that have economic implications are:
-
time is continuous and
-
stochastic movement is specified by a finite number of random shocks.
| |
Regularity of the money market account. Given the forward rate process in (11.4), the dynamics of the short-term interest rate may be expressed as:
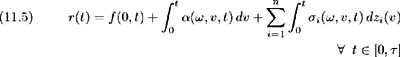
Now, define an accumulation factor or money market account B ( t ), as:
with initial condition B (0) = 1. The value of this money market account must satisfy :
To guarantee that this condition is satisfied, we require:
| |
11.2.2 Bond price process.
Here technical conditions are applied to parameters of the bond price process, thereby allowing the resulting bond price process to be well behaved.
| |
Regularity of the bond price process. To ensure a well-behaved bond price process, the following regularity conditions are imposed:
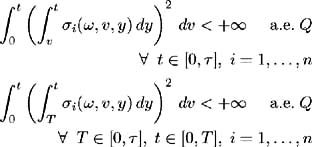
and
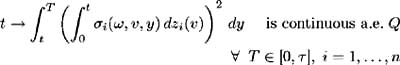
| |
Given Conditions 2 and 3 and using the lemma and two corollaries below, we determine the bond price process.
| |
This is a generalised form of the Fubini theorem for stochastic integrals. Given the following:
| ( , F,Q ) | ˆ’ | probability space, |
| { F t } | ˆ’ | filtration generated by a Brownian motion { z ( t ): t ˆˆ [0, ]} |
let { ( , t, a ):( t, a ) ˆˆ [0, ] [0, ]} be a family of real random variables such that
-
(( , t ), a ) ˆˆ {( [0, ]) [0, ]} ’ ( , t, a ) is L B [0, ] measurable [3] ,
-
ˆ« t 2 ( , s, a ) ds < + ˆ a.e. ˆ t ˆˆ [0, ];
-
ˆ« ( ˆ« ( , s, a ) da ) 2 ds < + ˆ a.e. ˆ t ˆˆ [0, ].
If t ’ ˆ« ( ˆ« ( , s, a ) dz ( s )) da is continuous a.e. then:
| |
| Proof | Let A and B be characteristic functions such that: and where A is a set { t : t ˆˆ [ s , )} and B ˆˆ F s . Now we have: where » is the Lebesque measure and Q the measure associated with filtration F . Also: Therefore: Let
Now an elementary function ˆ ˆˆ Since ˆˆ for some 0 S T . Therefore the Ito Integral of some function h ˆˆ where the limit is taken in L 2 ( P ) and { ˆ n } is a sequence of elementary functions such that: We have shown that the integral of any function h ˆˆ For an alternative description of this proof see [ 33 , Chapter 3, Problem 6.12]. |
| |
Assume Lemma 0.1 holds and define:
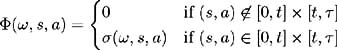
Then
| |
| Proof | 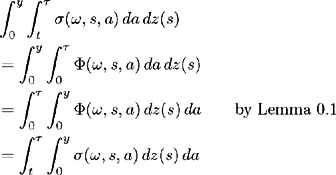 |
| |
Assume Lemma 0.1 holds and define:
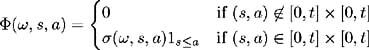
Then
| |
| Proof | 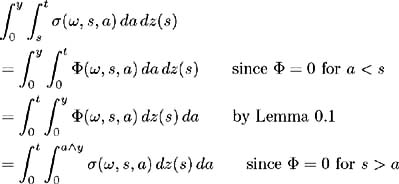 |
Now consider the bond price (11.2):
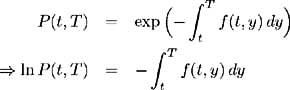
Substituting (11.4) we have [6] :
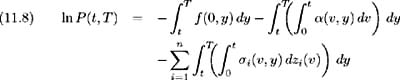
Now, apply the standard Fubini theorem to the double integral on ± ( v,y ) and Corollary 0.1.1 to the double integral on ƒ i ( v,y ) toget:
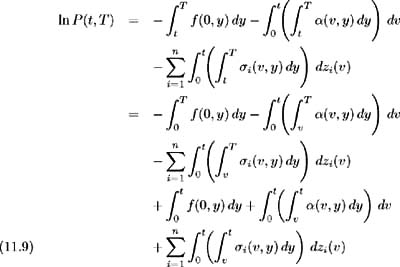
Applying Corollary 0.1.2 to the last two terms of (11.9) gives:
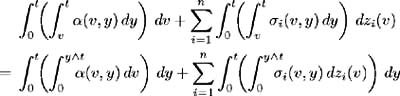
and from (11.2) we know:
Hence (11.9) becomes:
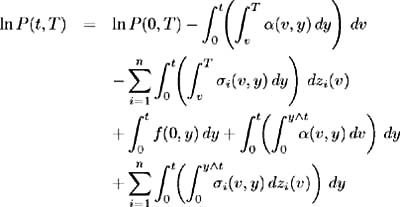
However, from (11.5) we have [7] :
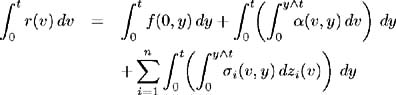
and so the dynamics of the bond price process are:
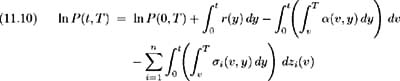
Let:
and (11.10) becomes:
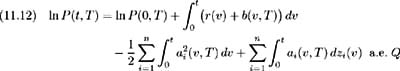
which may be expressed in differential form as:
Now applying Ito's Lemma, we find the differential equation satisfied by the bond price P ( t, T ), to be:
where
and so:
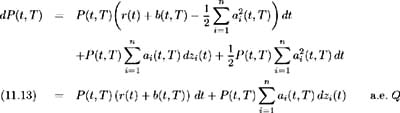
Since in (11.13) both the drift term r ( t )+ b ( , t, T ) and the volatility coefficients
a i ( , t, T ), i = 1, , n , may depend on the history of the Brownian motions, the bond price process is non-Markovian.
11.2.3 Relative bond price process.
Let ![]() T ˆˆ [0, ], t ˆˆ [0, T ] be the time t relative price of a T -maturity bond. Here, the bond price is expressed in terms of the money market account, so its drift with respect to the short-term interest rate is removed. Make use of Ito's Lemma to determine the dynamics of the relative bond price as [8] :
T ˆˆ [0, ], t ˆˆ [0, T ] be the time t relative price of a T -maturity bond. Here, the bond price is expressed in terms of the money market account, so its drift with respect to the short-term interest rate is removed. Make use of Ito's Lemma to determine the dynamics of the relative bond price as [8] :
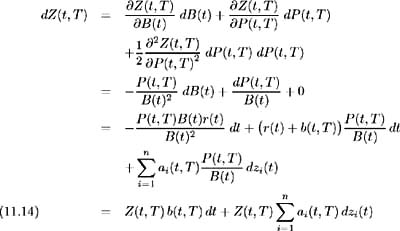
Also:
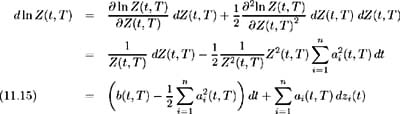
Hence the integral form of the relative bond price process is:
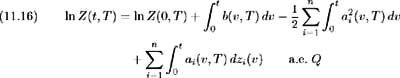
Again, the relative bond price is non-Markovian since the drift and volatility coefficients may depend on the history of the Brownian motions through the cumulative forward rate drift and volatility terms b ( , ·, T ) and a i ( , ·, T ), i = 1, , n .
[2] Here, and in subsequent formulae denotes the possible dependence on the history of the Brownian motions.
[3] L is the smallest ƒ -field on [0, ] such that all left-continuous F t -adapted processes Y :( , t ) ˆˆ [0, ] ’ Y ( , t ) ˆˆ R d are measurable.
[4] As before ![]() represents the Borel ƒ -algebra on [0, ˆ ).
represents the Borel ƒ -algebra on [0, ˆ ).
[5] The following has been adapted from [ 43 ].
[6] Here to improve readability, we suppress the notational dependence on .
[7] Directly integrating (11.5) yields:
However, by definition of ± ( v, y ) and ƒ i ( v, y ) as drift and volatility parameters of the forward rate process, we require v y for all v, y ˆˆ [0, ] and hence the upper limit on the inner integrals must become min ( y, t ) y ˆ§ t .
[8] Dynamics of the money market account are easily found from (11.6) as:
EAN: 2147483647
Pages: 132