Chapter 2: The Cox, Ingersoll and Ross Model
Cox, Ingersoll and Ross (CIR) view the problem of interest rate modelling as one in "general equilibrium theory" [ 18 ]. Anticipation of future events, risk preferences, other investment alternatives and consumption preferences all affect the term structure. CIR make use of a general equilibrium asset pricing model to endogenously determine the stochastic process followed by the short- term interest rate and the partial differential equation satisfied by the value of any contingent claim. Bond prices are then determined as solutions to this partial differential equation, contingent on the underlying short- term interest rate.
2.1 General equilibrium in a simple economy
In describing the equilibrium economy, Cox, Ingersoll and Ross [ 18 ] integrate real and financial markets. The specification of endogenous production and randomly changing technology allows randomly changing investment opportunities. Within a continuous time model, this characteristic allows the inclusion of effects that cannot be approximated in static, single period specifications of the economy.
| |
There is a single physical good which may be allocated to investment or for consumption. All values are expressed in terms of this good.
| |
| |
Production possibilities are represented as a set of n linear activities. A vector of amounts · , denominated in units of the physical good, invested in production, evolves according to a stochastic process of the form:
where
| w ( t ) | - | ( n + k )-dimensional Wiener process, |
| Y | - | k -dimensional vector of state variables , |
| I · | - | ( n n )-dimensional diagonal matrix function of · . Here the i th diagonal element is the i th component of · , |
| ± ( Y,t ) | - | n -dimensional vector of rates of return on production, |
| G ( Y,t ) | - | n ( n + k )-dimensional matrix representing the standard deviation of rates of return on production. Hence GG ² is the covariance matrix of rates of return on production. |
The stochastic process (2.1) describes the growth of an initial investment, given that output is continuously reinvested.
| |
| |
The k -dimensional vector of state variables Y , evolves according to the system of stochastic equations:
where
| ¼ ( Y,t ) | - | k -dimensional vector of drifts of the state variables, |
| S ( Y,t ) | - | k ( n + k )-dimensional matrix of standard deviations of the state variables. Hence SS ² is the covariance matrix of changes in state variables. |
These assumptions imply that the probability distribution of current output depends on the current level of the state variables, which themselves change randomly over time. Hence the development of the state variables Y determines the future available production opportunities. Unless GS ² is a null matrix [1] , changes in state variables are correlated with returns on the production processes.
| |
| |
Entry to all production processes is free.
| |
| |
The market for instantaneous borrowing and lending exists. This occurs at a rate r which is determined as part of the equilibrium in the economy.
| |
| |
There is a market for a variety of contingent claims to some amounts of the good. The payoffs of these claims may depend on aggregate wealth and level of the state variables. The values of the claims depend on the same variables that describe the state of the economy; hence the movement of the value of claim i , F i , is described by the stochastic differential equation:
where
| F i ² i ˆ’ i | - | mean price drift . i is the payout flow received, hence F i ² i is the total mean return, |
| h i | - | 1 ( n + k )-dimensional vector of standard deviations, hence h i h i ² is the variance of the rate of return. |
The equilibrium value of ² i , the rate of return on the contingent claim, and r , the risk-free rate of interest, are determined endogenously.
| |
| |
There is a fixed number of identical individuals, each wishing to maximise their objective function of the form:
where
| | - | expectation conditional on current wealth and state of the economy, |
| C ( s ) | - | consumption flow at time s , |
| U | - | von Neumann-Morgenstern utility function. It is assumed to be increasing, strictly concave, twice differentiable and to satisfy : |
| U ( C ( s ), Y ( s ), s ) k 1 (1 + C ( s ) + Y ( s )) k 2 | ||
| for some k 1 , k 2 > 0. |
| |
| |
Trading and investment take place continuously, at equilibrium prices only and free of transaction costs.
Consider the problem of allocating an individual's wealth to investment opportunities. If contingent claims exist, the solution will usually not be unique. We select a basis to be a set of production opportunities and contingent claims, such that any other contingent claims may be expressed as a linear combination thereof. Hence define the opportunity set as a basis of investment opportunities consisting of n production activities and k contingent claims. Individuals may allocate wealth among these ( n + k ) basis opportunities and the ( n + k + 1) th opportunity: riskless lending or borrowing. Since allocation to non-basis contingent claims may be replicated by a portfolio of basis claims, a unique allocation to basis investment opportunities is sufficient for valuation purposes.
Define:
| W | - | current total wealth, |
| a i W | - | amount of wealth invested in the i th production process, |
| b i W | - | amount of wealth invested in the i th contingent claim. |
Hence, for each individual, we wish to choose controls aW , bW and C to maximise expected lifetime utility, subject to the budget constraint denoted as [2] :
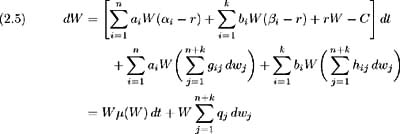
To maximise the utility function (2.4), the control must be a measurable function. This means the control, at any time, may only depend on information available at that time. Hence the following lemma defines the basic optimality condition for an individual's allocation (control) problem [3] (see [ 17 ]).
Lemma. Let J ( W, Y, t ) be the solution to the Bellman equation of the form:
for ( t, W, Y ) ˆˆ D [ t , t ² ) (0, ˆ ) R k and with boundary conditions
Then
-
J ( W, Y, t ) K ( v,W,Y,t ) for any admissible control v and initial W and Y ,
-
if v ^ is an admissible control such that
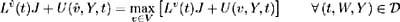
then

and v ^ is optimal.
Here J is called the indirect utility function.
| |
| |
There exists a unique function J and control v ^ satisfying the Bellman equation (2.6) and associated technical conditions.
Since investment proportions a i and consumption C must be non-negative, necessary and sufficient conditions for maximising ˆ = L v J + U as a function of C , a and b may be expressed as [ 17 ]:
Using (2.7) and the Bellman equation (2.6) ˆ , and b ^ may be found in terms of W , Y and t only. ˆ , and b ^ are chosen , taking r , ± and ² as given. The set of stochastic processes ( r , ² ; a, C ) (that is, the risk-free rate of interest, expected returns on contingent claims, production plan and consumption plan) define the equilibrium economy under the conditions ˆ‘ a i =1 and b i = 0 for all i . These conditions imply that in equilibrium, the interest rate and expected rates of return on the contingent claims are such that all wealth is invested in physical production processes only [ 18 ].
| |
[1] GS ² is the covariance matrix of changes in state variables and returns on production processes.
[2] Here w j denotes the j th element of w ( t ), i.e. the j th Wiener process.
[3] Here, define the following:
where v ( s ) is some admissible control. Also let L v ( t ) K be the associated differential operator defined as:
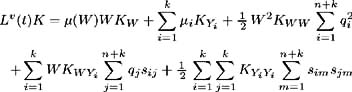
EAN: 2147483647
Pages: 132